A bob is attached to one end of weightless string and makes a vertical circle while looping . If the velocity of the bob at the lowest point is then calculate the radius of the greatest possible loop .
To solve the problem of finding the radius of the greatest possible loop for a bob attached to a weightless string, we can follow these steps:
Step 1: Understand the scenario
The bob is moving in a vertical circle, and we are given the velocity of the bob at the lowest point (70 m/s). We need to find the maximum radius of the loop.
Step 2: Apply the concept of centripetal force
At the highest point of the loop, the forces acting on the bob are its weight (mg) and the tension in the string (T). The centripetal force required to keep the bob moving in a circle is provided by the tension and the weight of the bob.
At the highest point, the equation can be written as:
T+mg=mv2r
Where:
- T is the tension in the string,
- m is the mass of the bob,
- g is the acceleration due to gravity (approximately 9.8m/s2),
- v is the velocity at the highest point,
- r is the radius of the loop.
Step 3: Determine the condition for maximum radius
For the maximum radius, the tension T at the highest point can be zero (the bob is just about to lose contact with the string). Thus, we can set T=0:
mg=mv2r
Step 4: Simplify the equation
Cancel out the mass m from both sides:
g=v2r
Rearranging gives us:
r=v2g
Step 5: Substitute the values
Now, substitute the values for v and g:
- v=70m/s
- g=9.8m/s2
So,
r=(70)29.8
Step 6: Calculate the radius
Calculating the above expression:
r=49009.8≈500m
Conclusion
The maximum radius of the loop is approximately 500m.
---
The bob is moving in a vertical circle, and we are given the velocity of the bob at the lowest point (70 m/s). We need to find the maximum radius of the loop.
At the highest point of the loop, the forces acting on the bob are its weight (mg) and the tension in the string (T). The centripetal force required to keep the bob moving in a circle is provided by the tension and the weight of the bob.
T+mg=mv2r
Where:
- T is the tension in the string,
- m is the mass of the bob,
- g is the acceleration due to gravity (approximately 9.8m/s2),
- v is the velocity at the highest point,
- r is the radius of the loop.
For the maximum radius, the tension T at the highest point can be zero (the bob is just about to lose contact with the string). Thus, we can set T=0:
mg=mv2r
Cancel out the mass m from both sides:
g=v2r
r=v2g
Now, substitute the values for v and g:
- v=70m/s
- g=9.8m/s2
r=(70)29.8
Calculating the above expression:
r=49009.8≈500m
The maximum radius of the loop is approximately 500m.
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Conceptual Questions
20 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Tough & Tricky (PROBLEMS)
15 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Chapter Practice Test
16 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A stone is tied to one end of a string and rotated in a vertical circle . What is the difference in tensions of the string at lowest and highest points of the vertical circle ?
View SolutionAn aeroplane describes a vertical circle when looping. Find the radius of the greatest possible loop if the velocity of the aeroplane at the lowest point of its path is 50ms−1. Take g=10ms−2.
View Solution
Knowledge Check
A point mass m is moved in vertical circle of radius r with help of string. Velocity of mass is 7gr√ at lowest point. Tension in string at lowest point is
A7 mgB8 mgC1 mgD6 mgA body of mass m tied to a string is moved in a vertical circle of radius r. The difference in tensions at the lowest point and the highest point is
A2mgB6mgC4mgD8mgA body of mass 0.1 Kg attached at the end of a string 6m long , is whirled in a vertical circle . The tension in the string is 6.4 N at the lowest point . What is the maximum angular velocity the body ?
(g=10m/s2)A12 rad /sB9 rad/sC6 rad /sD3 rad /s
A stone of mass 100 g attached to a string of length 50 cm is whirled in a vertical circle by giving it a velocity of 7 m/s at the lowest point. Find the velocity at the highest point .
View SolutionA child revolves a stone of mass 0 .5 kg tied to the end of a string of length 40 cmin a vertical circle . The speed of the stone at the lowest point of the circle is 3m/s Calculate tension in the string at this point.
View SolutionA stone is tied to a weightless string and revolved in a vertical circle of radius 5 m . What should be the minimum speed of the stone at the highest point of the circle so that the string does not slack ? What should be the speed of the stone at the lowest point of vertical circle ? Take g=9.8ms2 .
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
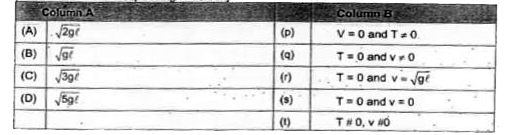
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View Solution
