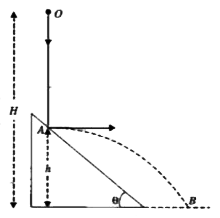
A body falling freely from a given height H hits an inclined plane in its path at a height h. As a result of this impact the direction of the velocity of the body becomes horizontal. For what value of (h/H), the body will take the maximum time to reach the ground.

Text Solution

Verified by Experts

The body falls from O and strikes the plane at A at a height h above the ground. After the impact the velocity of the body becomes horizontal.
Let the time taken by the body to fall through a height H-h be $t_{1}$
$s = u t + \frac{1}{2} a t^{2}$
$s = H - h, t = t_{1} = ?, a = g, u = 0$
$H - h = \frac{1}{2} g t_{1}^{2}$
$t_{1} = \sqrt{\frac{2 (H - h)}{g}}$
After the impact let the body strike the ground at B. Let $t_{2}$ be the time taken by the body to fall from A to B.
$s = h, u = 0, a = g, t = t_{2} = ?$
$h = \frac{1}{2} g t_{2}^{2} = \sqrt{\frac{2 h}{g}}$
Total time taken by the body $= t_{1} + t_{2}$
$t = \sqrt{\frac{2 (H - h)}{g}} + \sqrt{\frac{2 h}{g}}$
To find the value of h/H for which the body will take the maximum time $\frac{d t}{d h} = 0$ .
$\frac{d}{d h} [\sqrt{\frac{2 (H - h)}{g}} + \sqrt{\frac{2 h}{g}}] = 0$
$- \frac{1}{2} {(H - h)}^{- \frac{1}{2}} + \frac{1}{2} h^{- \frac{1}{2}} = 0, - \frac{1}{\sqrt{H - h}} + \frac{1}{\sqrt{h}} = 0$
$H - h = h, \frac{h}{H} = \frac{1}{2}$ .

Updated on:21/07/2023