A body floats on a liquid of specific gravity S1 with f part of it immersed in that liquid. It also floats on another liquid of specific gravity S2 with (1 - f) part of it immersed. Prove that the specific gravity of the body = S1S2S1+S2.Solution in Bengali
Step by step video & image solution for A body floats on a liquid of specific gravity S_(1) with f part of it immersed in that liquid. It also floats on another liquid of specific gravity S_(2) with (1 - f) part of it immersed. Prove that the specific gravity of the body = (S_(1)S_(2))/(S_(1)+S_(2)). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - HOTS NUMERICAL PROBLEMS
21 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - ENTRANCE CORNER (ASSERTION-REASON TYPE)
7 Videos
- HYDROSTATICSBOOK - CHHAYA PUBLICATIONCHAPTER - HYDROSTATICSEXERCISE - PROBLEM SET - I
29 Videos
- FRICTION BOOK - CHHAYA PUBLICATIONCHAPTER - FRICTION EXERCISE - CBSE SCANNER
7 Videos
- KINETIC THEORY OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - KINETIC THEORY OF GASESEXERCISE - CBSE Scanner
9 Videos
Similar Questions
A U-tube is partly filled with water. A liquid, immiscible in water, is poured into one arm until the level of the liquid comes to a height h above the water level in the other arm. The water in the second arm is at a height d above its previous level in this arm. Calculate the density of the liquid.
View SolutionStatement I : If a barometer is accelerated upwards, the level of mercury in the tube of the barometer will decrease.
Statement II : The effective value of g will increase, so upthrust will increase.
View SolutionThe specific gravity of silver is 10.5. Calculate the density of silver in the CGS system and in SI.
View SolutionDefine angle of friction. The inclination θ of a rough plane is increased gradually. The ply on the plane just comes into motion when inclination θ becomes 30∘. Find coefficient of friction. If the inclination is further increased to 45∘ then find acceleration of the body along the plane. (g = 10 m.s−2)
View SolutionWhat would be the effect on the rms speed of gas molecules if the temperature of the gas is increased by a factor of 4?
View SolutionIf S_(1),S_(2),S_(3) be respectively the sums of n,2n and 3n terms of a G.P.,prove that S_(1)(S_(3)-S_(2))=(S_(2)-S_(1))^(2)
View SolutionIf S1,S2,S3 be respectively the sums of n,2n,3n terms of a G.P., then prove that (S1)2+(S2)2=S1(S2+S3) .
View SolutionAs Fig. shows, S1 and S2, are spring balances. Block A is hanging from spring balance S1 and immersed in liquid L which is contained in beaker B. The mass of beaker B is 1kg and mass of liquid L is 1.5kg. Balances S1 and S2 reads 2.5kg and 7.5kg, respectively. What will be the readings of S1 and S2 when block A is pulled up out of the liquid. Find the reading of S1 and S_(2)?
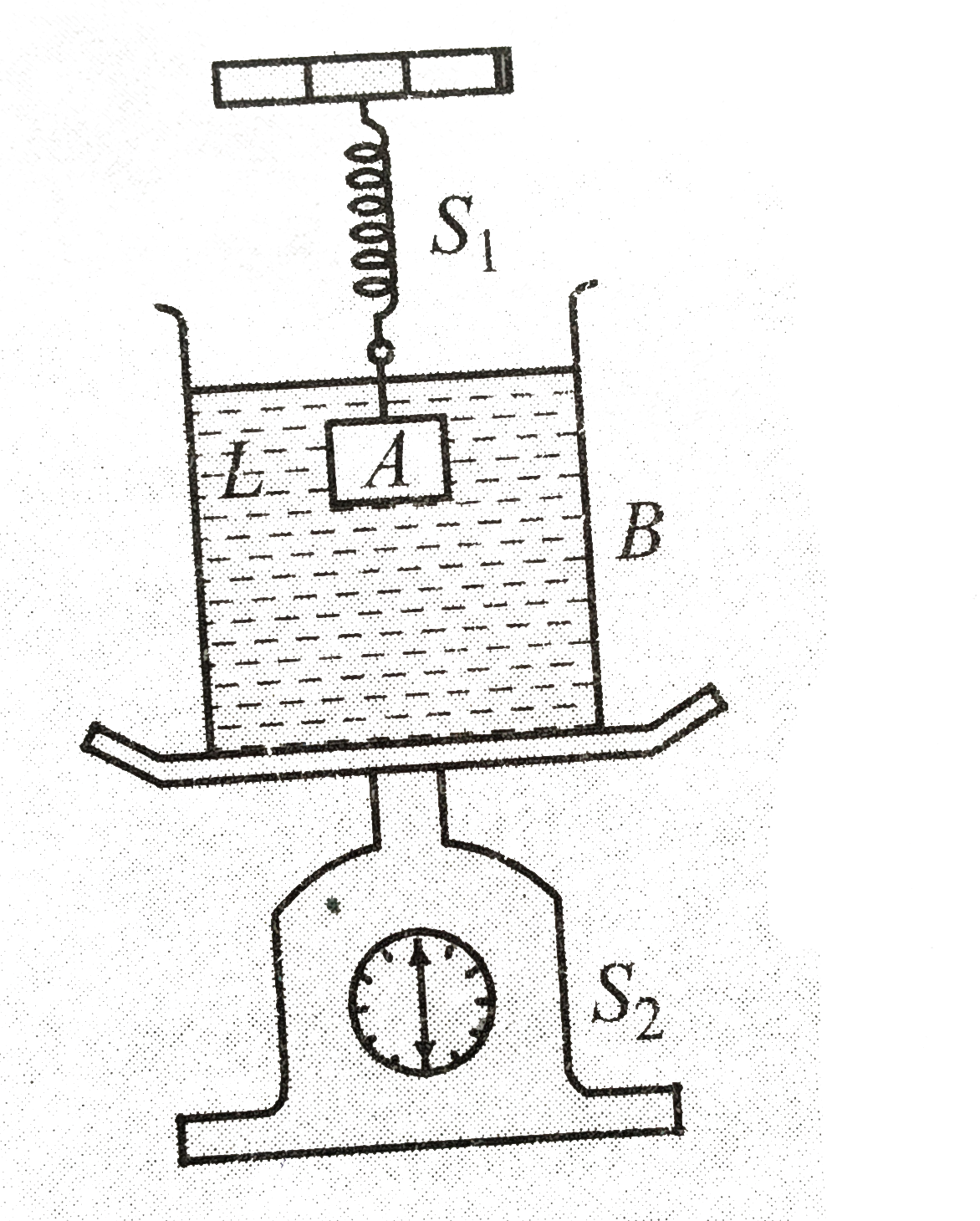
View SolutionIn making an alloy, a substance of specific gravity s_(1) and mass m_(1) is mixed with another substance of specific gravity of the alloy is
View SolutionIf S_(1) is the specific gravity of a solid with respect to a liqid and S_(2) is the specific gravity of the liquid with respect to water, then the specific gravity of the solid with respect to water is
View SolutionEqual masses of three liquids are thoroughly mixed. The specific heat capacities of the liquids ar s1,s2 and s3 and their temperatures t1,t2 and t3 respectively. Find the temperature of the mixture.
View Solutionयदि गुणोत्तर श्रेणी के n, 2n और 3n पदों के योगफल क्रमशः S1,S2 और S3 है, तो सिद्ध करो कि
S1(S3−S2)=(S2−S1)2
View SolutionIf S1,S2,S3 be respectively the sums of n, 2n and 3n terms of a G.P., prove that, S1(S3−S2)=(S2−S1)2.
View Solutionयदि गु० श्रे० के n,2n,3n पदों का योग क्रमशः S1,S2,S3 हों तो सिद्ध करें की S21+S22=S1(S2+S3).
View SolutionWhen a body is immersed separately into three liquids of specific gravities S1,S2andS3, its apparent weights become W1,W2andW3 respectivley. Show that S1(W2−W3)+S2(W3−W1)+S3(W1−W2)=0.
View Solution
