A body is pivoted at a point. A force of 10N is applied at a distance of 30cm from the pivot. Calculate the moment of force about the pivot.
To calculate the moment of force about the pivot, we can follow these steps:
1. Identify the Given Values:
- Force (F) = 10 N
- Distance (D) from the pivot = 30 cm = 0.3 m (convert cm to m)
2. Understand the Formula for Moment of Force:
- The moment of force (M) about a pivot is calculated using the formula:
M=F×D
where:
- M = moment of force (in Newton-meters)
- F = applied force (in Newtons)
- D = distance from the pivot to the point of force application (in meters)
3. Substitute the Values into the Formula:
- Substitute F = 10 N and D = 0.3 m into the formula:
M=10N×0.3m
4. Calculate the Moment of Force:
- Perform the multiplication:
M=3N m
5. State the Final Answer:
- The moment of force about the pivot is 3 Newton-meters (N m).
Final Answer:
The moment of force about the pivot is 3 N m.
---
- Force (F) = 10 N
- Distance (D) from the pivot = 30 cm = 0.3 m (convert cm to m)
- The moment of force (M) about a pivot is calculated using the formula:
M=F×D
where:
- M = moment of force (in Newton-meters)
- F = applied force (in Newtons)
- D = distance from the pivot to the point of force application (in meters)
- Substitute F = 10 N and D = 0.3 m into the formula:
M=10N×0.3m
- Perform the multiplication:
M=3N m
- The moment of force about the pivot is 3 Newton-meters (N m).
The moment of force about the pivot is 3 N m.
Topper's Solved these Questions
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1A
27 Videos
- FORCE BOOK - ICSECHAPTER - FORCE EXERCISE - EXERCISE 1(A) Multiple choice questions
2 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Video
- HOUSEHOLD CIRCUITS BOOK - ICSECHAPTER - HOUSEHOLD CIRCUITS EXERCISE - EXERCISE -9(B) (MULTIPLE CHOICE TYPE)
2 Videos
Similar Questions
If the Mechanical Advantage of a lever of 100, when a force of 10N acts as a distance of 20cm from the point of fulcrum. Calculate the force acting on the other side of the fulcrum and the distance of that force from the fulcrum.
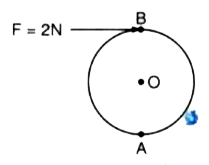
View SolutionA wheel of diameter 2m is shown in Fig 1.28 with axle at O. At force F= 2N is applied at B in the direction shown in figure, Calculate the moment of force about (i) the centre O, and (ii) the point A.
View SolutionA spanner is used to unscrew a nut. A force of 30 N is applied to the end of the spanner, which is 10 cm away from the centre of the nut. Calculate the moment of force when the spanner is horizontal.
View SolutionA spanner is used to unscrew a nut. A force of 40 N is applied to the end of the spanner, which is 10 cm away from the centre of the nut. Calculate the moment of force when the spanner is horizontal.
View SolutionA rod is pivoted about its centre. A 10 N force is applied 4 m from the pivot and another force of 5 N is applied 2 m from the pivot, as shown. The magnitude of the total torque about the pivot is

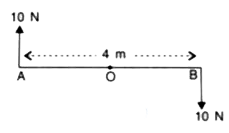
View SolutionThe forces each of magnitude 10N act vertically upwards and downwards respectively at the two ends A and B of a uniform rod of length 4m which is pivoted at its mid point O as shown is fig. 1.30, Determine the magnitude of the resultant moment of forces about the pivot O.
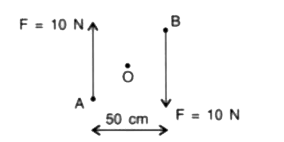
View SolutionFig.1.31 shows two forces each of magnitude 10N acting at points A and B at a separation of 50cm, in opposite directions. Calculate the resultant moment of the two forces about the point (i) A (ii) B and (iii) O situtated exactly at the middle of the two forces.
View SolutionThe following diagram (Fig. 1.20) shows two parallel and opposite forces F1andF2 each of magnitude 5N, with their lines of action separated by a distance of 2m. A point X is pivoted midway between F1andF2 while a point Y is pivoted on F2
(a) calculate the total moment of the two forces about the points (i) X, and (ii) Y
(b) State the effect produced by the two forces about the points (i) X and (ii) Y
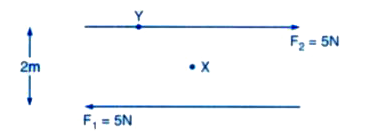
View SolutionIn the figure (i) half of the meter scale is made of wood while the other half, of steel. The wooden part is pivoted at O. A force F is applied at the end of steel part. In figure (ii) the steel part is pivoted at O and the same force is applied at the wooden end

View SolutionThe diagram in Fig.1.29 shows two forces F1=5NandF2=3N acting at points A and B of a rod pivoted at a point O, such that OA=2m and OB=4m
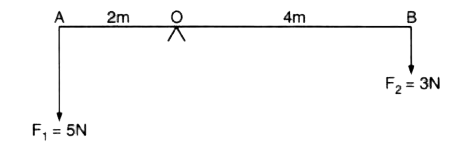
Calculate:
(i) the moment of force F1 about O.
(ii) the moment of force F2 about O.
(iii) total moment of the two forces about O.
View Solution
