Question

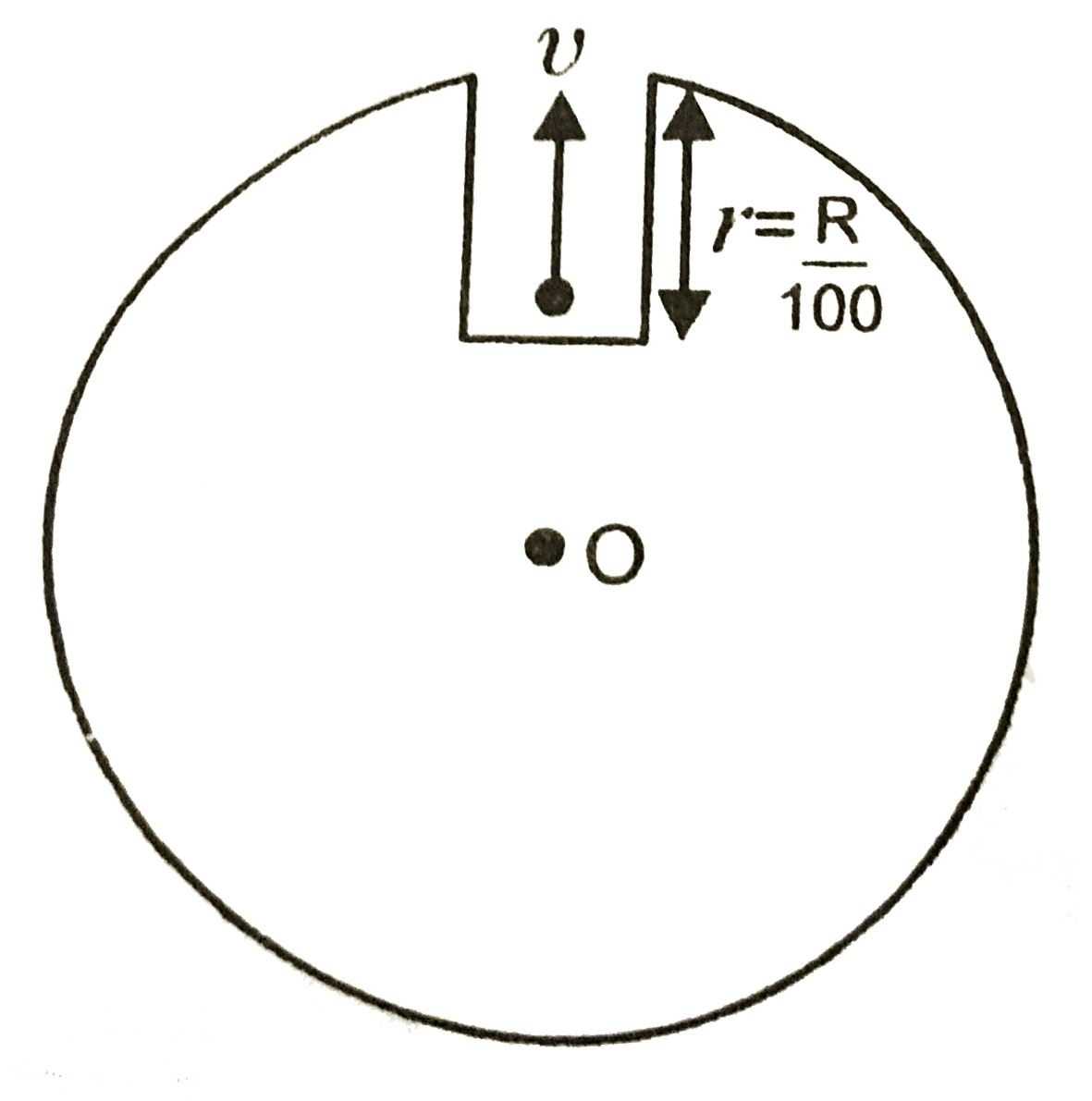
A body is projected vartically upwards from the bottom of a crater of moon of depth $\frac{R}{100}$ where R is the radius of moon with a velocity equal to the escape velocity on the surface of moon. Calculate maximum height attained by the body formt eh surface of the moon.

Options

A. `R`
B. `85 R`
C. `99 R`
D. `100 R`

Answer

C

Explanation

Let `upsilon` be the velocity of projection of the particle from the cavity at a depth `r(R//100)` in the moon,
where `upsilon = sqrt(2GM//R)`
We know that if point lies inside the sphere of radius `R`, mass `M` at disatnce `r` from the centre of sphere, where `r lt R`, then gravitational potential at that point is

`V = -(GM(3R^(2) -r^(2)))/(2R^(3))`
In the givenquestion, `r = (R-R//100)`
`= (99 R//100)`
Form law of conservation of total energy
`(PE + KE)` at the cavity `= PE` at height `h`
`-(GM m)/(2R^(2))[3R^(2)-((99R)/(100))^(2)]+ (1)/(2)m upsilon^(2) = -(GMm)/(R + h)`
or `-(GMm)/(2R^(3))[3R^(2)-0.98R^(2)] + (1)/(2)m((2GM)/(R )) = -(GMm)/(R + h)`
On solving, we get, `h = 99R`