A body of density ρ is dropped from rest from height h (from the surface of water) into a lake of density of water σ(σ>ρ). Neglecting all disspative effects, the acceleration of body while it is in the take is
g(σρ−1) upwards
g(σρ−1) downwards
g(σρ) upwards
g(σρ) downwards
The correct Answer is:A
To solve the problem of determining the acceleration of a body of density ρ when it is dropped into a lake of density σ (where σ>ρ), we can follow these steps:
1. Identify the Forces Acting on the Body:
- When the body is submerged in the water, two main forces act on it:
- The weight of the body acting downwards: Fweight=V⋅ρ⋅g
- The buoyant force acting upwards: Fbuoyant=V⋅σ⋅g
- Here, V is the volume of the body, g is the acceleration due to gravity, ρ is the density of the body, and σ is the density of the water.
2. Set Up the Equation of Motion:
- According to Newton's second law, the net force acting on the body is equal to the mass of the body times its acceleration:
Fnet=Fbuoyant−Fweight=m⋅a
- The mass m of the body can be expressed as m=V⋅ρ.
3. Substitute the Forces into the Equation:
- Substitute the expressions for the forces into the equation:
V⋅σ⋅g−V⋅ρ⋅g=V⋅ρ⋅a
4. Simplify the Equation:
- Factor out V⋅g from the left side:
V⋅g(σ−ρ)=V⋅ρ⋅a
- Cancel V from both sides (assuming V≠0):
g(σ−ρ)=ρ⋅a
5. Solve for Acceleration a:
- Rearranging the equation gives:
a=g(σ−ρ)ρ
6. Final Expression for Acceleration:
- Thus, the acceleration of the body while it is in the lake is:
a=g(σρ−1)
Conclusion:
The acceleration of the body while it is in the lake is directed upwards and is given by the formula:
a=g(σρ−1)
- When the body is submerged in the water, two main forces act on it:
- The weight of the body acting downwards: Fweight=V⋅ρ⋅g
- The buoyant force acting upwards: Fbuoyant=V⋅σ⋅g
- Here, V is the volume of the body, g is the acceleration due to gravity, ρ is the density of the body, and σ is the density of the water.
- According to Newton's second law, the net force acting on the body is equal to the mass of the body times its acceleration:
Fnet=Fbuoyant−Fweight=m⋅a
- The mass m of the body can be expressed as m=V⋅ρ.
- Substitute the expressions for the forces into the equation:
V⋅σ⋅g−V⋅ρ⋅g=V⋅ρ⋅a
- Factor out V⋅g from the left side:
V⋅g(σ−ρ)=V⋅ρ⋅a
- Cancel V from both sides (assuming V≠0):
g(σ−ρ)=ρ⋅a
- Rearranging the equation gives:
a=g(σ−ρ)ρ
- Thus, the acceleration of the body while it is in the lake is:
a=g(σρ−1)
The acceleration of the body while it is in the lake is directed upwards and is given by the formula:
a=g(σρ−1)
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 More Than One Correct
10 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Comprehension Based
7 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A ball of density ρ is dropped from a height on the surface of a non-viscous liquid of density 2ρ. Choose the correct options.

View SolutionA body of density ρ is dropped from a height h into a lake of density σ(σ>ρ). The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
View SolutionA small body of density ρ' is dropped from rest at a height h into a lake of density ρ, where ρ>ρ’. Which of the following statements is/are correct if all dissipative effects are neglected (neglect viscosity)?
View SolutionAn object of density σ(<ρ) is dropped from rest at a height h into a liquid of density ρ kept in a tall vertical cylindrical container. Neglect all dissipative effects and assume that there is no spilling of the liquid.
The time taken by the object to reach the starting point (from which it is dropped) is
View SolutionA body of mass 2 kg is dropped from rest from a height 20 m from the surface of Earth. The body hits the ground with velocity 10 m/s, then work done: (g=10m/s2)
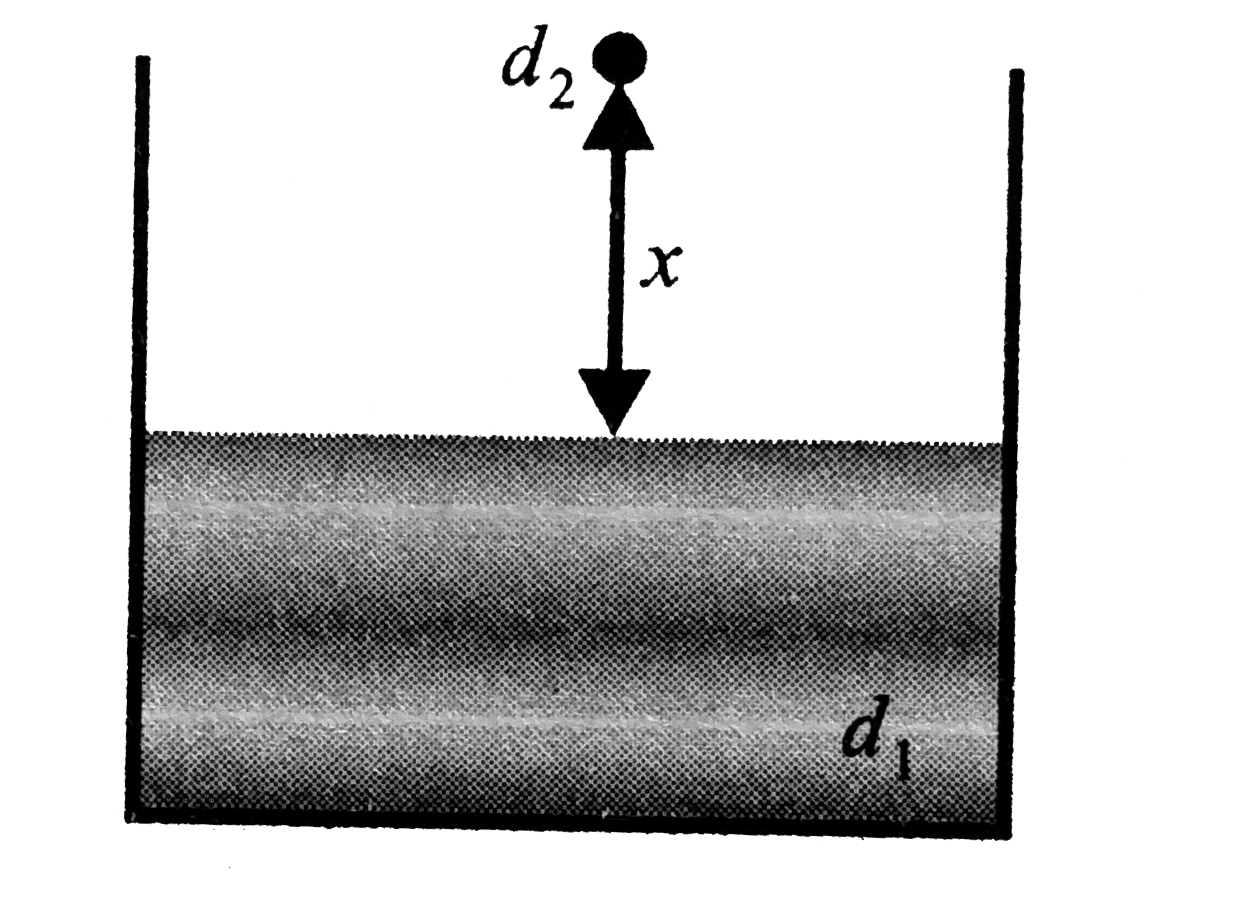
View SolutionA body of density d2 is dropped from rest at a height x into a beaker having a liquid of density d1(d2>d1). Which of the following is/are true?
View SolutionA small ball is dropped from a certain height on the surface of a non-viscous liquid of density less than the density of ball. The motion of the ball is
View SolutionA boll made up of a cork material of relative density 12, is dropped from rest from a height 20 m into a lake. Neglecting all dissipative forces, calculate the maximum dept to which the body sinks before returning to float on the surface (poission ratio is zero)
View SolutionA stone of relative density K is released from rest on the surface of a lake. If viscous effects are lgnored, the stone sinks in water with an acceleration of
View SolutionA ball of density ρ0 falls from rest from a point P onto the surface of a liquid of density ρ in the time T. It enters the liquid, stops, moves up, and returns to P in a total time 3 T. neglect viscosity, surface tension and splashing find the ratio of ρρ0
View Solution
