A bomp thrown with certain speed explodes in mid air before hitting its target. What will be effect on its total kinetic energy?
To analyze the effect on the total kinetic energy of a bomb that explodes in mid-air before hitting its target, we can break down the problem step by step.
1. Understanding Initial Conditions:
- When the bomb is thrown, it has an initial kinetic energy (K_initial) due to its speed. This kinetic energy can be calculated using the formula:
Kinitial=12mv2
where m is the mass of the bomb and v is its velocity.
2. Explosion of the Bomb:
- As the bomb explodes in mid-air, it breaks into several fragments. Each fragment will have its own kinetic energy after the explosion.
3. Energy Transformation:
- The explosion converts the chemical energy stored in the bomb into various forms of energy, including:
- Kinetic energy of the fragments
- Heat energy
- Sound energy
- Light energy
- Some of the chemical energy will be transformed into kinetic energy, which means the total kinetic energy after the explosion will be affected.
4. Final Kinetic Energy Calculation:
- After the explosion, the total kinetic energy (K_final) will be the sum of the kinetic energies of all the fragments. This can be expressed as:
Kfinal=Kfragment 1+Kfragment 2+…+Kfragment n
5. Comparison of Kinetic Energies:
- Since the explosion adds additional kinetic energy from the conversion of chemical energy, the total kinetic energy after the explosion will be greater than the initial kinetic energy of the bomb. Therefore:
Kfinal>Kinitial
6. Conclusion:
- The total kinetic energy of the bomb after it explodes will increase due to the conversion of chemical energy into kinetic energy, along with other forms of energy.
Final Answer:
The total kinetic energy of the bomb will increase after it explodes in mid-air.
- When the bomb is thrown, it has an initial kinetic energy (K_initial) due to its speed. This kinetic energy can be calculated using the formula:
Kinitial=12mv2
where m is the mass of the bomb and v is its velocity.
- As the bomb explodes in mid-air, it breaks into several fragments. Each fragment will have its own kinetic energy after the explosion.
- The explosion converts the chemical energy stored in the bomb into various forms of energy, including:
- Kinetic energy of the fragments
- Heat energy
- Sound energy
- Light energy
- Some of the chemical energy will be transformed into kinetic energy, which means the total kinetic energy after the explosion will be affected.
- After the explosion, the total kinetic energy (K_final) will be the sum of the kinetic energies of all the fragments. This can be expressed as:
Kfinal=Kfragment 1+Kfragment 2+…+Kfragment n
- Since the explosion adds additional kinetic energy from the conversion of chemical energy, the total kinetic energy after the explosion will be greater than the initial kinetic energy of the bomb. Therefore:
Kfinal>Kinitial
- The total kinetic energy of the bomb after it explodes will increase due to the conversion of chemical energy into kinetic energy, along with other forms of energy.
The total kinetic energy of the bomb will increase after it explodes in mid-air.
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Tough & Tricky (PROBLEMS)
15 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - NCERT FILE (NCERT Textbook Exercise)
23 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - PRACTICE PROBLEMS
27 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
If the speed of body is halved, what will be the change in its kinetic energy?
A1/4 timesB1/6 timesC1/8 timesD1/10 timesA bomb dropped from an aeroplane explodes in air. Its total :
Amomentum decreasesBmomentum increasesCkinetic energy increasesDkinetic energy decreasesThe speed of rolling of a ring of mass m changes from V to 3V . What is the change in its kinetic energy
A3MV2B4MV2C6MV2D8MV2
A shot fired from a cannon explodes in air. What will be the changes in the momentum and the kinetic energy?
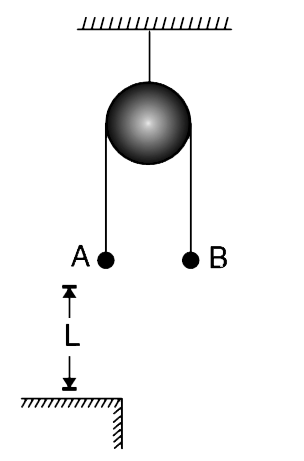
View SolutionTwo particle A and B, of mass 3mand2m respectively, are attached to the ends of a light inextensible string which passes over a smooth fixed pulley of negligible mass. After the system is released and A falls through a distance L, it hits a horizontal inelastic table so that its speed is immediately reduced to zero. Assume that B never hits the table or the pulley. Find
(a) the time for which A is resting on the table after the first collision and before it is jerked off,
(b) the difference between the total kinetic energy of the system immediately before A first hits the table and total kinetic energy immediately after A starts moving upwards for the first time. Explain the loss in kinetic energy.
View SolutionWhen a sphere rolls without slipping the ratio of its kinetic energy of translation to its total kinetic energy is.
View SolutionA solid spherical ball rolls on a table. Ratio of its rotational kinetic energy to total kinetic energy is
View SolutionA shell is fired from a cannon, it explodes in mid air, its total
View Solution
