A car goes on a horizontal circular road of radius R, the speed increasing at a constant rate dvdt=a. The friction coefficient between the road and the tyre is μ. Find the speed at which the car will skid.
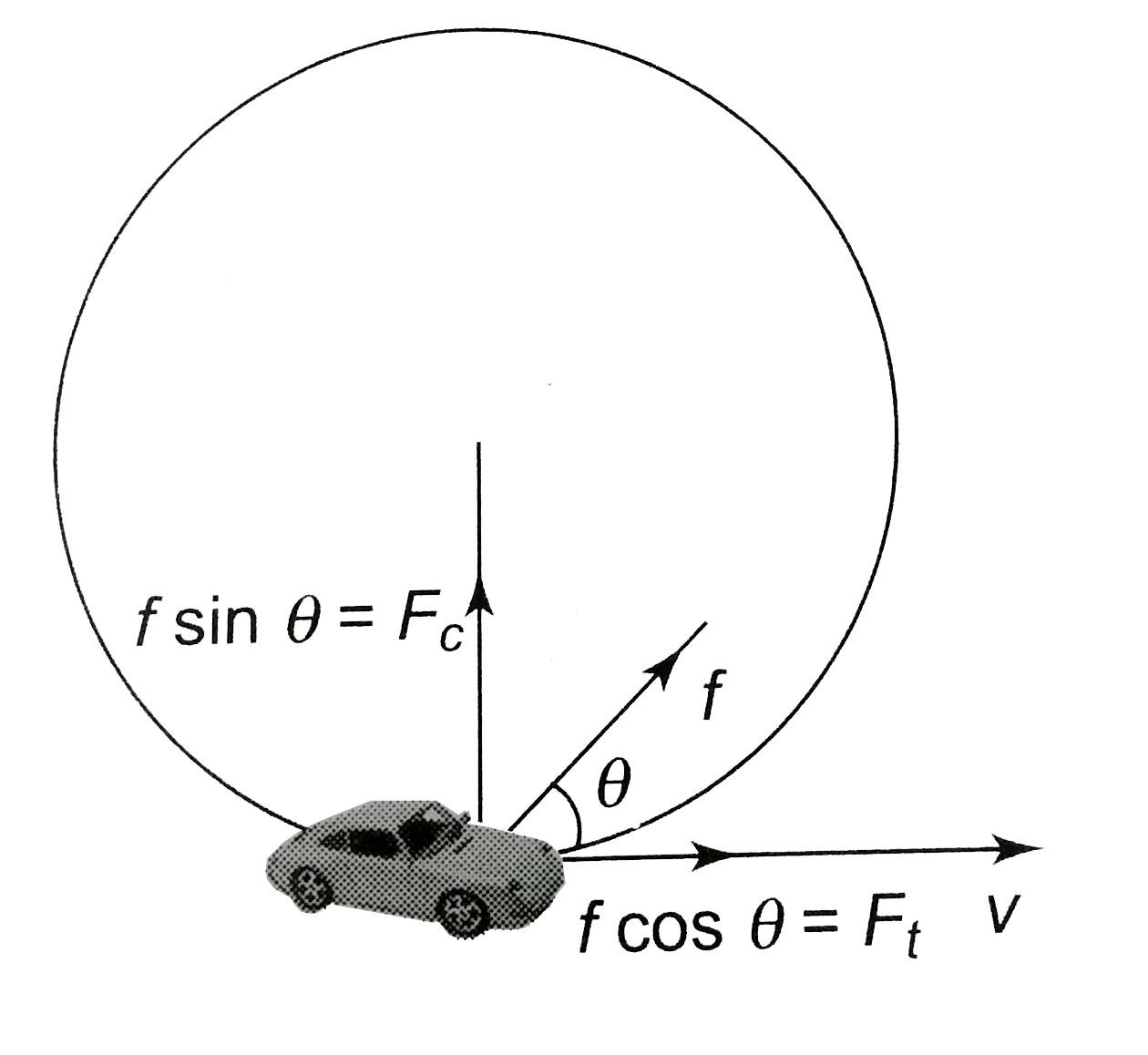
This is the case of non-uniform circular motion. Here the friction will be inclined to velocity. One component of the friction will provide necessary centripetal force and another component will provide tangential force.
fsinθ=Fc=mv2R
fcosθ=Ft=mat=mdvdt=ma0
f=√F2c+F2t=√(mv2R)2+(ma0)2
f=√_(max)=μN=μmg
for no slipping or skidding. we have
f≤fmax
√(mv2R)2+(ma0)2≤μmg
v4R2+a20≤μ2g2
v≤[(μ92)g2−a20)R2]1/4
Maximum speed for no skid
vmax=[(μ2g2−a20)R2]1/4
in the context of tangential acceleration:
V2=μ2+2a1s=0+2a0s
s=v2max2a0=R2[(μga0)2−1]1/2
Topper's Solved these Questions
Similar Questions
A car goes on a horizontal circular road of radius R. The speed of car is increasing at constant rate a m/s2. If the friction coefficient between the road and the tyre is μ, then the limiting speed of car is
View SolutionA car starts rest, on a horizontal circular road of radius R, the tangential acceleration of the car is a. The friction coefficient between the road and the tyre is μ Find the speed at which car will skid and also find the distance after travelling it skids.
View Solution
Knowledge Check
A car starts moving from rest on a horizontal circular road of radius of curvature R at . The speed of the car is increasing at a constant rate a. The coefficient of friction between the road and the tyres of the car is The car starts slipping at timet=t0 . Then t0 is equal to:
A.4√μ2g2R2aB.4√R2(μ2g2−a2)aC.4√R2(a2−μ2g2)aD.4√R2(μ2g2+a2)aA car moves along a horizontal circular road of radius r with constant speed v. The coefficient of friction between the wheels and the road is μ. Which of the following statement is not true ?
AThe car slips if v>√μrgBThe car slips if μ<(v2/rg)CThe car slips if μ>(v2/rg)DThe car slips at a lower speed if it moves with some tangential acceleration, then if it moves at constant speedA body moves on a horizontal circular road of radius of r, with a tangential acceleration aT. Coefficient of friction between the body and road surface is μ. It begin to slip when it's speed is v, then :
Av2=μrgBug=v2r+aTCμ2g2=v4r2+a2TDThe force of friction makes an angle tan−1(v2aT×r) with direction of motion at point of slipping.
A car starts from rest in a circular flat road of radius R with an acceleration a. The friction coefficient between the road and the tyred is μ Find the distance car will travel before is start skidding.
View SolutionA car starts from rest on a horizontal circular road of radius 190 m and gains speed at a uniform rate 1⋅2m/s2 . The coefficient of static between the road and the tyres is 0⋅37 . Calculate the distance travelled by the car before it begins to skid.
View SolutionA car is moving on a horizontal circular road of radius 0.1 km with constant speed. If coefficient of friction between tyres of car and road is 0.4, then speed of car may be (g=10m/s2)
View SolutionA car of maas M is moving on a horizontal circular path of radius r. At an instant its speed is v and is increasing at a rate a.
View SolutionA car of maas M is moving on a horizontal circular path of radius r. At an instant its speed is v and is increasing at a rate a.
View Solution
