A car is negotiating a curved road of radius R. The road is banked at angle $θ$ . The coefficeint of friction between the tyres of the car and the road is $μ_{s}$ . The maximum safe velocity on this road is

A

$\sqrt{g R (\frac{μ_{s} + tan θ}{1 - μ_{s} tan θ})}$

B

$\sqrt{\frac{g}{R} (\frac{μ_{s} + tan θ}{1 - μ_{s} tan θ})}$

C

$\sqrt{\frac{g}{{(R)}^{2}} (\frac{μ_{s} + tan θ}{1 - μ_{s} tan θ})}$

D

$\sqrt{g R^{2} (\frac{μ_{s} + tan θ}{1 - μ_{s} tan θ})}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

According to question, a car is negotiating a curved road of radius R. The road is banked at angle $θ$ and the coefficient of friction between the tyres of car and the road is $μ_{s}$ . So, this given situation can be drawn as shown as in figure below.

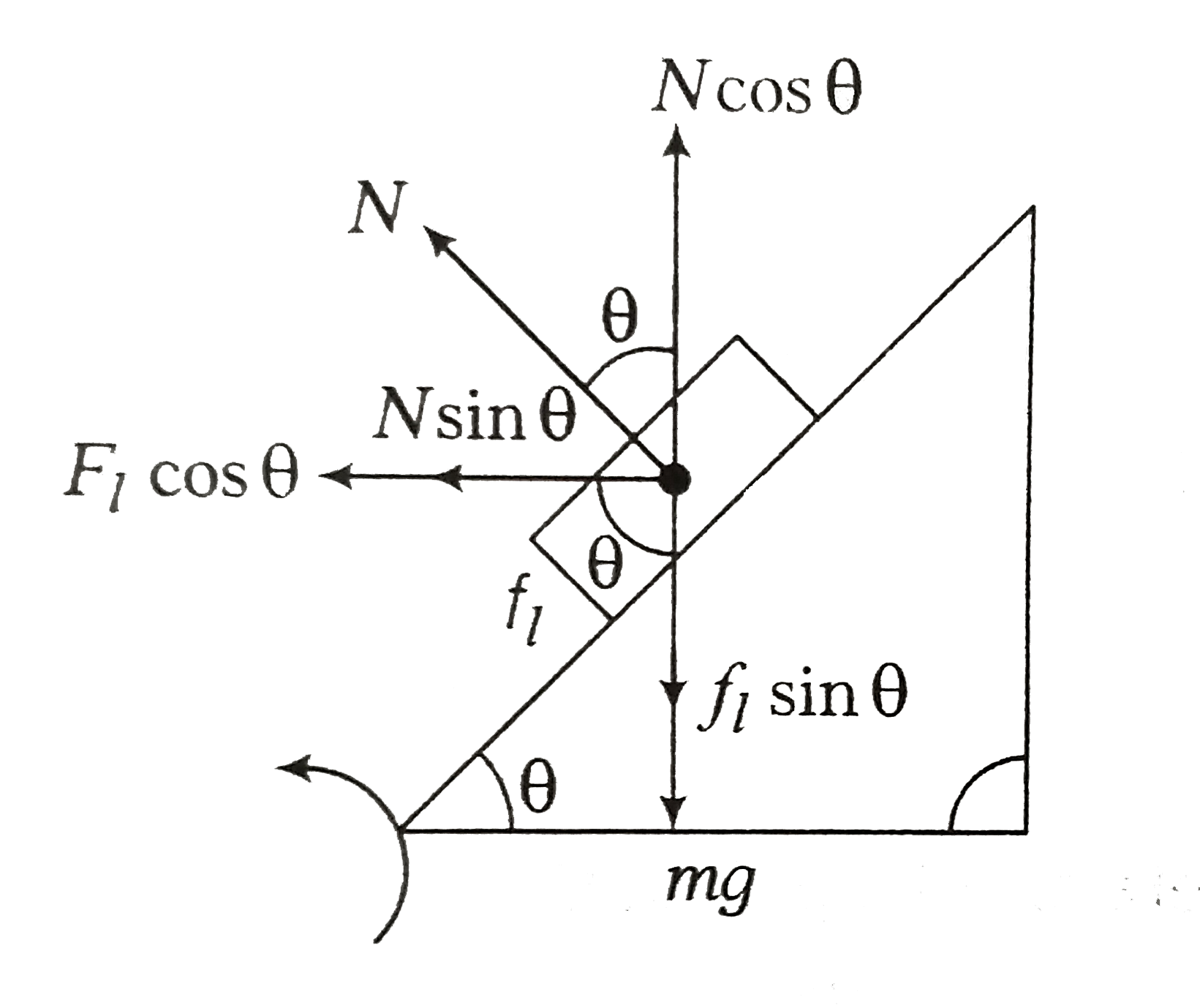
Considering the case of vertical equilibrium
$N cos θ = m g + f_{l} sin θ$
$\Rightarrow m g = N cos θ - f_{l} sin θ$ ....(i)
Considering the case of horizontal equilibrium,
$N sin θ + f_{l} cos θ = \frac{m v^{2}}{R}$ ....(ii)
On dividing Eq. (i) by Eq. (ii), we get
$\frac{V^{2}}{R g} = \frac{sin θ + μ_{s} cos θ}{cos θ - μ_{s} sin θ} [f_{l} \propto μ_{s}]$
$\Rightarrow v = \sqrt{R g (\frac{sin θ + μ_{s} cos θ}{cos θ - μ_{s} sin θ})} \Rightarrow v = \sqrt{R g (\frac{tan θ + μ_{s}}{1 - μ_{s} tan θ})}$

|

Updated on:21/07/2023

Class 11 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A car is negotisting a curved road of radius $R$ . The road is banked at an angle theta. The coefficient of friction between the tyres of the car and the road is $μ_{s}$ . The maximum safe velocity on this road is:
A $\sqrt{g R^{2} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
B $\sqrt{g R \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
C $\sqrt{\frac{g}{R} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
D $\sqrt{\frac{g}{R^{2}} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
Question 2 - Select One
A car is negotiating a curved road of radius R. The road is banked at an angle $θ$ . The coefficient of friction between the tyres of the car and the road is $μ_{s}$ . The maximum safe velocity on this road is
A $\sqrt{\frac{g}{R} \frac{(μ_{s} + tan θ)}{R (1 - μ_{s} tan θ)}}$
B $\sqrt{\frac{g}{R^{2}} \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}}$
C $g R^{2} \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}$
D $\sqrt{g R \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}}$
Question 3 - Select One
A car is negotisting a curved road of radius $R$ . The road is banked at an angle theta. The coefficient of friction between the tyres of the car and the road is $μ_{s}$ . The maximum safe velocity on this road is:
A $\sqrt{g R^{2} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
B $\sqrt{g R (μ_{s} + tan θ) (1 - μ_{s} tan θ)}$
C $\sqrt{\frac{g}{R} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$
D $\sqrt{\frac{g}{R^{2}} \frac{μ_{s} + tan θ}{1 - μ_{s} tan θ}}$

DC PANDEY-CIRCULAR MOTION-Medical entrances s gallery

Home
Ask Doubt
Profile