A car moving at a speed v is stopped in a certain distance when the brakes produce a deceleration a. If the speed of car was nv , what must be the decceleration of the car to stop it in the same distance and in the same time? Solution in Kannada
n3a
na
n2a
√na
The correct Answer is:c
When speed is made n times then distance require to stop the body by same retarding force become n2 time.
Topper's Solved these Questions
- DESCRIPTION OF MOTION IN ONE DIMENSION BOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN ONE DIMENSION EXERCISE - MULTIPLE CHOICE QUESTION (LEVEL-II)
54 Videos
- DESCRIPTION OF MOTION IN ONE DIMENSION BOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN ONE DIMENSION EXERCISE - Recent Competitive Question
12 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - RECENT COMPETITIVE QUESTIONS
32 Videos
- DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONBOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONEXERCISE - Revision TEST
36 Videos
Similar Questions
A man moves along the path abode as shown in the Fig. What is his displacement from the point of start?
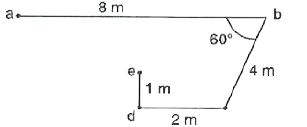
View SolutionA train is moving slowly on a straight track with a constant speed of 2 m s−1 . A passengers in the train starts walking at a stready speed of 2 m s−1 to the back of the train in the opposite direction of the motion of the train. So to an observer standing on the platform directly in front of that passenger, the velocity of the passenger appears to be
View SolutionA battery of emf E has an internal resistance r. A variable resistance R is connected to the terminals of the battery. A current i is drawn from the battery. V is the terminal potential difference. If R alone is gradually reduced to zero, which of the following best describes i and V?
View SolutionThe torque of a force →F=−3ˆi+ˆj+5ˆk acting at a point is →τ. If the position vector of the point vector of the point is 7ˆi+3ˆj+ˆk, then →τ is :
View SolutionA car moving with a speed of 40km/h can be stopped by applying the brakes after at least 2 m. If the same car is moving with a speed of 80km/h, what is the minimum stopping distance?
View SolutionWhen the speed of the car is v, the minimum distance over which it can be stopped is x. If the speed becomes nv, what will be the minimum distance over which it can be stopped during same time:
View SolutionA car moving with a speed of 40 km/hr can be stopped by applying brakes after at least 2 m. If the same car is moving with a speed of 80 km/hr. What is the stopping distance ?
View Solution50 किमी/घण्टा की चाल से गतिमान एक कार ब्रेक लगाकर 6 मीटर की दूरी पर रोक दी जाती है। यदि कार 100 किमी/घण्टा की चाल से गतिमान हो तो कार उसी ब्रेक से कितनी न्यूनतम दूरी में रूक जायेगी?
View Solution80 किमी/घण्टा की चाल से गतिमान एक कार ब्रेक लगाकर 10 सेकण्ड में रोक ली जाती हैं यदि कार 120 किमी/घण्टा की चाल से गतिमान हो तो कार उसी ब्रेक से कितने न्यूनतम समय में रूक जायेगी।
View SolutionA car moving with speed v on a straight road can be stopped with in distance d on applying brakes. If same car is moving with speed 3v and brakes provide half retardation, then car will stop after travelling distance
View SolutionA car moving with a speed of 50km.h−1 can be stopped by brakes after at least 6 m. What will be the minimum stopping distance, if the same car is moving at a speed of 100km.h−1?
View SolutionA car moving with a speed of 50 km/hr can stopped by brakes, over a distance of 6 m. If the same car is moving at a speed of 100 km/hr. the stopping distance is
View SolutionA car is moving on a straight horizontal road with a speed v. When brakes are applied to give a constant retardation a, the car is stopped in a shortest distance S. If the car was moving on the same road with a speed 3v and the same retardation a is applied, the shortest distance in which the car is stopped will be
View SolutionIf x is the distance at which a car can be stopped when initially it was moving with speed u, then on making the speed of the car Nu, the distance at which the car can be stopped is
View SolutionA car moving with a speed of 40 km/h be stopped by applying brakes after at least 2 m , if the same car is moving with a speed of 80km/h . What is the minimum stopping distance ?
View Solution
