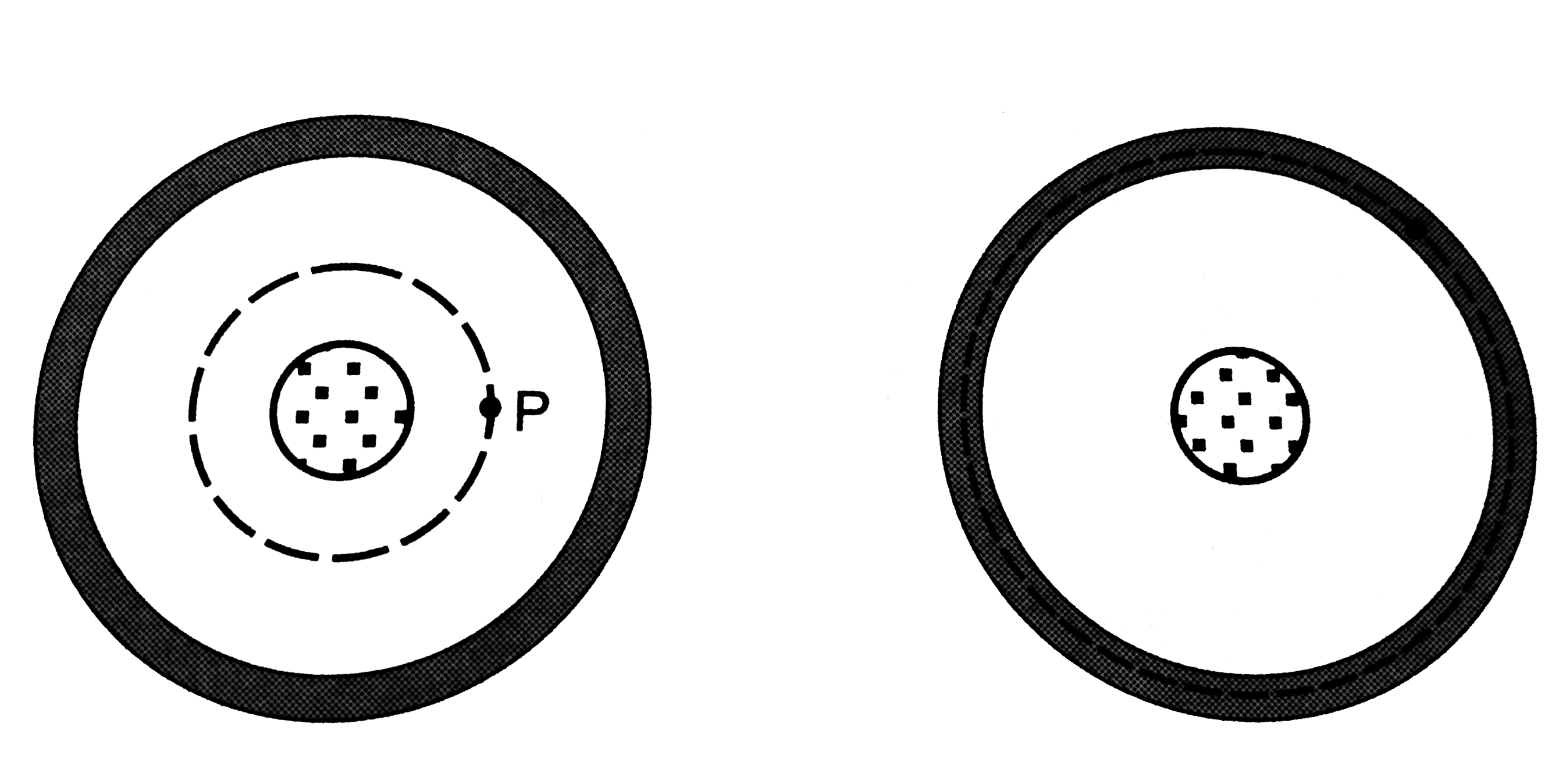
A Charge of 4×10−8C is distributed uniformaly on the surface of a sphere of radius 1 cm. It is covered by a concentric, hollow conducting sphere of radius 5 cm. (a) Find the electric field at a point 2 cm away from the centre. (b) A charge of 6×10−8C is placed on the hollow sphere. Find the surface charge density on the outer surface of the hollow sphere.Solution in Tamil
(a) Let us consider figure. Suppose, we have to find the field at the point P. Draw a concentric spherical surface thought P. All the points on this surface are equivalent and by symmetry, the field at all these points will be equal in magnitude and radial in direction.
The flux through this surface =∮→Ed→S
∮EdS=E→dS
=4πx2E,
where x=2cm=2×10−2m.
From Gauss's law, this flux is equal to the charge q contained inside the surface divided by ε0. Thus,
4πx2E=qeπlon0
or E=q4πeπlon0x2
=(9×109Nm2C−2)×4×10−8C4×10−4ma
=9×105NC−1.<br>(b)SeefigureTakeaGaussionsurfacethroughthematerialofthehollowsphere.Ase≤ctricfield∈aconduct∈gmaterialiazero,thefluxoint vecEdvecSThroughthisGaussiansurfaceiszera.UsingGauss'slaw,the→talchar≥enclsedμstbezer⊙Hence,thechar≥onthe∈≠rsurfaceofthehollowsphereis-4 xx 10^(-8) C.Butthe→talchar≥given→thishollowsphereis6 xx 10^(-8) C.Hencethechar≥ontheoutersurfacewillbe10 xx 10^(-8) C.
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - GAUSS LAWEXERCISE - Short Question7videos
GAUSS LAW
- BOOK - HC VERMACHAPTER - GAUSS LAWEXERCISE - Objective 17videos
GAUSS LAW
- BOOK - HC VERMACHAPTER - GAUSS LAWEXERCISE - Exercises24videos
GAUSS LAW
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise9videos
ELECTROMAGNETIC WAVES
- BOOK - HC VERMACHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercises79videos
GEOMETRICAL OPTICS
Similar Questions
A small plane area is rotated in an electric field. In which orientation of the area is the flux of electric field through the area maximum? In which orientation is it zero.?
01:10View SolutionA charge Q is uniformly distributed over a large plastic plate. The electric field at a point P close to the centre of the plate is 10Vm−1. If the plastic plate is replaced by a copper plate of the same geometrical dimension and carrying the same charge Q the electric field at the point P will become
02:34View SolutionThe electric field in a region is given by →E=35E0→i+45E0→j with E0=2.0×103NC−1. Find the flux of this field through a recatngular surface of area 0.2m2 parallel to the y-z plane.
01:21View SolutionShow that the dimensions of the displacement current (ε0dΦEdt) are that of an electric current.
04:33View SolutionA concave mirror having a radius of curvature 40 cm is placed in front of an illuminated point source at a distance of 30 cm from it. Find the location of the image.
02:12View SolutionA hollow metal sphere of radius 5 cms is charged such that the potential on its surface is 10 volts. The potential at the centre of the sphere is
02:34View SolutionA charge q is distributed uniformly on the surface of a sold sphere of radius R. It covered by a concentric hollow conduction sphere of radius 2R . Find the charges inner and outer surface of hollow sphere if it is earthed.
04:04View SolutionA hollow metal sphere of radius 5cm is charged so that the potential on its surface is 10V. The potential at the centre of the sphere is
View SolutionA hollow, spherical conductor of radius 10 cm is charged with 5×10−9C. Find the potential (a) at a distance of 20 cm from the centre ,(b) at the surface of the sphere , (c) at a distance of 5 cm from centre.
View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
View SolutionA charge q is distributed uniformly on the surface of a sold sphere of radius R. It covered by a concentric hollow conduction sphere of radius 2R. Find the charges inner and outer surface of hollow sphere if it is earthed.
 02:22View Solution
02:22View SolutionSurface charge density of a conducting sphere of a radius 10 cm is 8.85×10−8c/m2. Potential at the centre of the sphere is
01:57View SolutionA point charge is placed at the centre of a hollow conducting sphere of internal radius ' and outer radius '2r. The ratio of the surface charge density of the inner surface to that of the outer surface will be ___________ .
02:48View SolutionA hollow metallic sphere of radius 5 cm is charged so that the potential on its surface is 10 V. Thepotential at the centre of the sphere is
03:48View SolutionA hollow metal sphere of radius 5 cm is charged such that the potential on its surface is 10 V. What is the potential at the centre of the sphere?
01:27View SolutionA hollow metal sphere of radius 10 cm is charged such that the potential on its surface is 5 V. What is the potential at the centre of the sphere?
01:55View Solution

