A charged particle projected in a magnetic field
B=(3ˆi+4ˆj)×10−2T
The acceleration of the particle is found to be
a=(xˆi+2ˆj)ms2
find the value of x
To find the value of x in the given problem, we will follow these steps:
Step 1: Understand the relationship between force, acceleration, and magnetic field
The force F acting on a charged particle moving in a magnetic field is given by the equation:
F=q(v×B)
where q is the charge of the particle, v is the velocity vector, and B is the magnetic field vector.
Step 2: Recognize the direction of acceleration
Since the force is perpendicular to the magnetic field B, the acceleration a will also be perpendicular to B. This means:
a⋅B=0
Step 3: Write down the vectors
Given:
B=(3^i+4^j)×10−2T
a=x^i+2^jm/s2
Step 4: Set up the dot product equation
To find x, we set up the equation:
a⋅B=0
Substituting the vectors:
(x^i+2^j)⋅(3^i+4^j)×10−2=0
Step 5: Calculate the dot product
Calculating the dot product:
=(x⋅3+2⋅4)×10−2=0
This simplifies to:
3x+8=0
Step 6: Solve for x
Rearranging the equation gives:
3x=−8
x=−83m/s2
Final Answer
The value of x is:
x=−83m/s2
---
The force F acting on a charged particle moving in a magnetic field is given by the equation:
F=q(v×B)
where q is the charge of the particle, v is the velocity vector, and B is the magnetic field vector.
Since the force is perpendicular to the magnetic field B, the acceleration a will also be perpendicular to B. This means:
a⋅B=0
Given:
B=(3^i+4^j)×10−2T
a=x^i+2^jm/s2
To find x, we set up the equation:
a⋅B=0
Substituting the vectors:
(x^i+2^j)⋅(3^i+4^j)×10−2=0
Calculating the dot product:
=(x⋅3+2⋅4)×10−2=0
This simplifies to:
3x+8=0
Rearranging the equation gives:
3x=−8
x=−83m/s2
The value of x is:
x=−83m/s2
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - INTRODUCTORY EXERCISE1videos
MAGNETICS
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE1videos
MAGNETICS
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery59videos
MAGNETIC FIELD AND FORCES
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery1videos
MAGNETISM AND MATTER
Similar Questions
A charged particle moves in a uniform magnetic field B=(2ˆi−3ˆj) T
View SolutionA charged particle moves in a magnetic field →B=10ˆi with initial velocity →u=5→i+4ˆj. The path of the particle will be
View SolutionA charged particle has acceleration →a=2ˆi+xˆj in a magnetic field →B=−3ˆi+2ˆj−4ˆk.Find the value of x.
View SolutionA particle is projected from ground with velocity 3ˆi+4ˆj m/s. Find range of the projectile :-
View SolutionIf a particle of charge 1μC is projected into a magnetic field →B=(2ˆi+yˆj−zˆk) T with a velocity →V=(4ˆi+2ˆj−6ˆk)ms−1, then it passes undeviated. If it is now projected with a velocity →V=ˆi+ˆj , then find the force experienced by it?
View SolutionA charged particle carrying charge 1μC is moving with velocity (2ˆi+3ˆi+4ˆk)ms−1. If an external magnetic field of (5ˆi+3ˆj−6ˆk)×10−3T exists in the region where the particle is moving then the force on the particle is →F×10−9N. The vector →F is :
View SolutionVelocity and acceleration of a particle are v=(2ˆi−4ˆj)ms and a=(−2ˆi+4ˆj)ms2 Which type of motion is this?
View SolutionA particle has an initial velocity 3ˆi+3ˆj and acceleration of 0.41ˆi+0.3ˆj. Its speed after 10s is
View SolutionA particle has an initial velocity of 4ˆi+3ˆj and an acceleration of 0.4ˆi+0.3ˆj. Its speed after 10s is
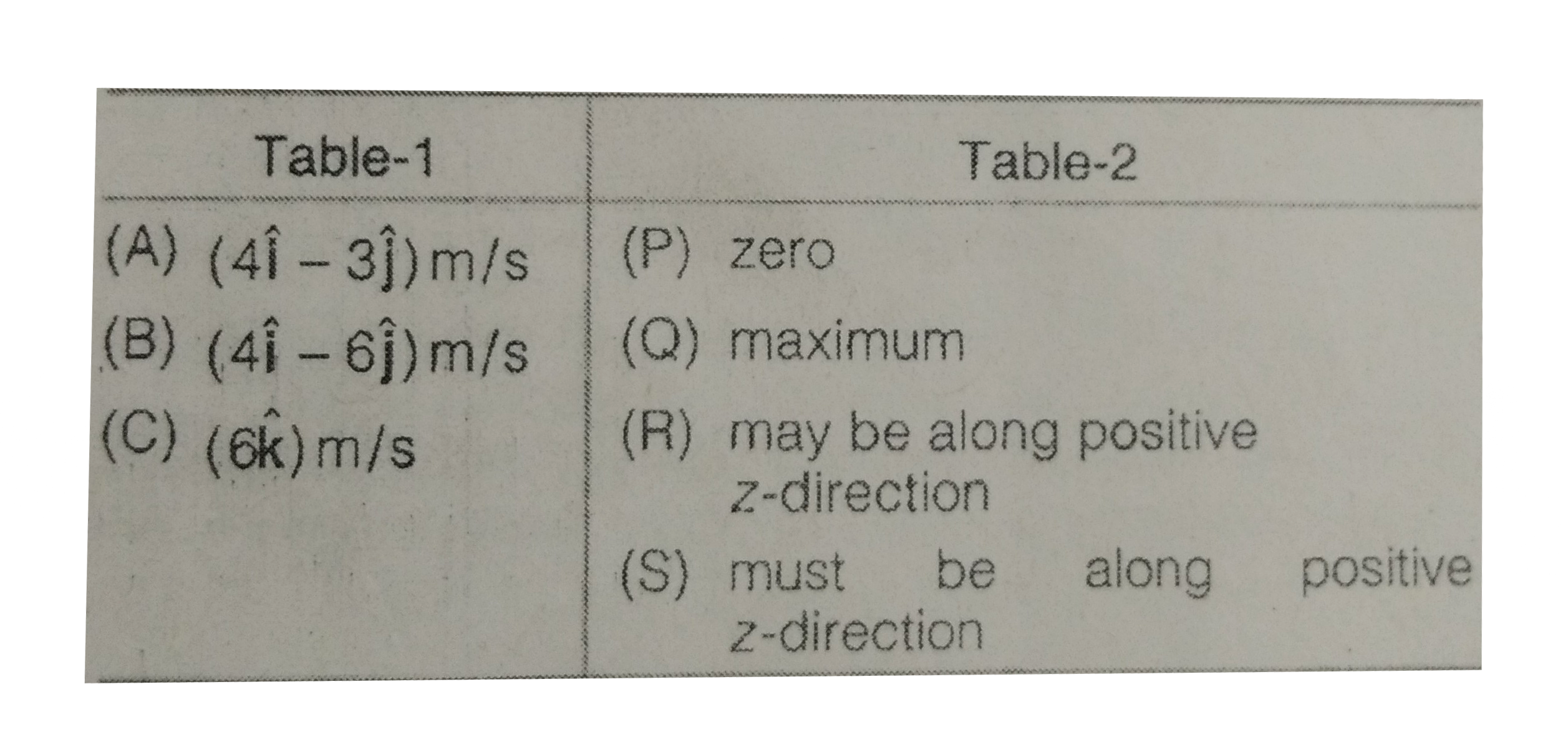
View SolutionIn magnetic field B=(2ˆi+3ˆj) T, velocity of a charged particle is given in table-1. Corresponding magnetic force is given in table-2. Match the two tables.
 View Solution
View Solution

