A circuit draws a power of 550 watt from a source of 220 volt, 50 H z . The power factor of the circuit is 0.8 and the current lags in phase behind the potential difference. To make the power factor of the circuit as 1.0, The capacitance should be connected in series with it is

A

1 42 π × 10 - 2 F

B

1 41 π × 10 - 2 F

C

1 5 π × 10 - 2 F

D

1 84 π × 10 - 2 F

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

As the current lags behind the potential difference, the circuit contains resistance and inductance.
Power, P = V rms × i rms × cos ϕ
Here, I rms = V rms Z , where Z = [ ( R ) 2 + ( ω L ) 2 ]
∴ P = V rms 2 Z × cos ϕ or Z = V rms 2 P × cos ϕ
So, Z = ( 220 ) 2 × 0.8 550 = 70.4 Ω
Now, Power factor cos ϕ = R Z or R = Z cos ϕ
∴ R = 70.4 × 0.8 = 56.32 Ω
Further, Z 2 = R 2 + ( ω L ) 2 or ω L = ( Z 2 - R 2 )
or ω L = ( 70.4 ) 2 - ( 56.32 ) 2 = 42.2 Ω
When the capacitor is connected in the circuit,
∴ Z = [ R 2 + ( ω L - 1 ω C ) 2 ]
According to the question,
Power factor cos ϕ = 1
Hence, R = Z cos ϕ = Z × 1 , R = Z
∴ Z = Z 2 + ( ω L - 1 ω C ) 2 , ( ω L - 1 ω C ) 2 = 0
∴ C = 1 ω ( ω L ) = 1 2 π υ ( 42.2 ) = 1 2 π × 50 × 42.2 ≈ 1 42 π × 10 - 2 F

Updated on:21/07/2023
|
Class 12PHYSICSALTERNATING CURRENT

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The power factor of the circuit in fig. is 1/√2. The capacitance of the circuit is equal to
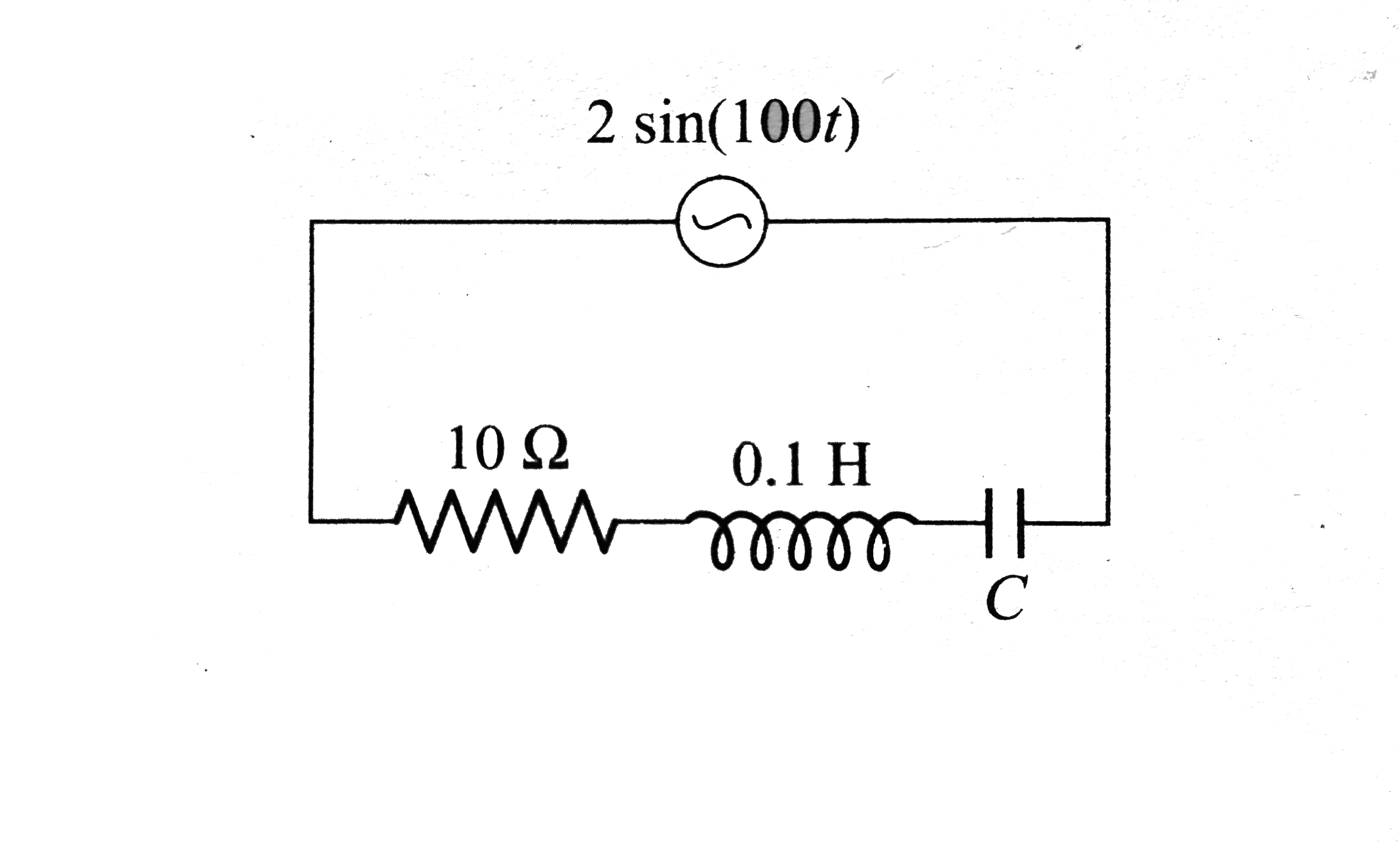    ocr_image

    A400(μ)F
    B300(μ)F
    C500(μ)F
    D200(μ)F

  • The power factor of a series LCR circuit at resonance will be

    A1
    B0
    C12
    D1√2

  • The power factor for a purely capacitive circuit is

    A1
    B√2
    C1√2
    DZero