A circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure . The density of the material and the thickness are same everywhere . Show that the centre of mass of the composite system lies inside the square plate .

The centre of masses of the circular plate and the square are at their centres namely C1 and C2 respectively .

Now the masses of the plates are proportional to their areas (density = density , t = thickness) .
m1=ρ⋅t⋅(πd24),m2=ρ⋅t⋅d2
With C1 as the origin
xCM=m1(0)+m2(d)m1+m2=ρ⋅t⋅d2⋅dρt(πd24+d2)=d(π4+1)
But 1+π4=1+3.144<2⇒11+π4>12 or d1+π4>d2
Thus the centre of mass of the system lies inside the square plate .
Topper's Solved these Questions
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - NEET CAFE (TOPICWISE PRACTICE QUESTIONS )
140 Videos
- MOTION OF SYSTEM OF PARTICLES AND RIGID BODY BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - CHECK YOUR NEET VITALS
14 Videos
- LAWS OF MOTIONBOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - AIPMT /NEET (MCQ)
24 Videos
- OSCILLATIONS AND WAVES BOOK - MTG GUIDECHAPTER - OSCILLATIONS AND WAVES EXERCISE - AIPMT / NEET MCQs
43 Videos
Similar Questions
A circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure. The densit of the material and the thickness are same
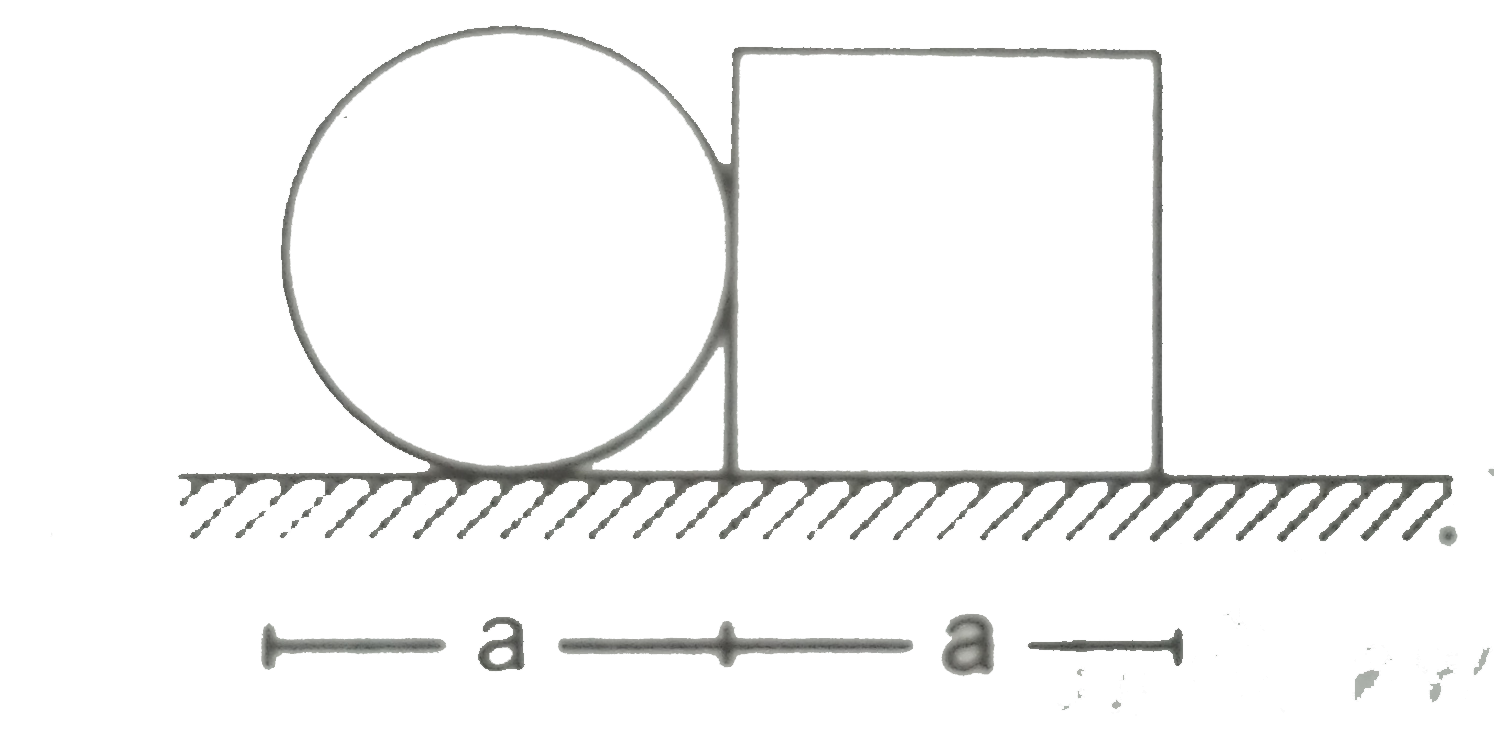 . Everywhere. The centre of mass of the composite system will be
. Everywhere. The centre of mass of the composite system will be
View SolutionA circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure. The densit of the material and the thickness are same
 . Everywhere. The centre of mass of the composite system will be
. Everywhere. The centre of mass of the composite system will be
View Solution
Knowledge Check
A circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure. The densities of the material and the thickness are same
 . Everywhere. The centre of mass of the composite system will be Ainside the circular plateBinside hte square plateCat the point of contactDoutside the system
. Everywhere. The centre of mass of the composite system will be Ainside the circular plateBinside hte square plateCat the point of contactDoutside the systemA circular plate of diameter a is kept in contact with a square plate of edge a as shown. The density of the material and the thickness are same everywhere. The centre of mass of the composite system will be
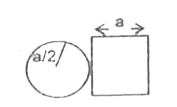 Ainside the circular plateBinside the square plateCat the point of contactDoutside the system
Ainside the circular plateBinside the square plateCat the point of contactDoutside the system
A circular plate of diameter 'a' is kept in contact with a square plate of side a as shown. The density of the material and the thickness are same everywhere. The centre of mass of composite system will be
View SolutionA circular plate of diameter 'a' is kept in contact with a square plate of side a as shown. The density of the material and the thickness are same everywhere. The centre of mass of composite system will be
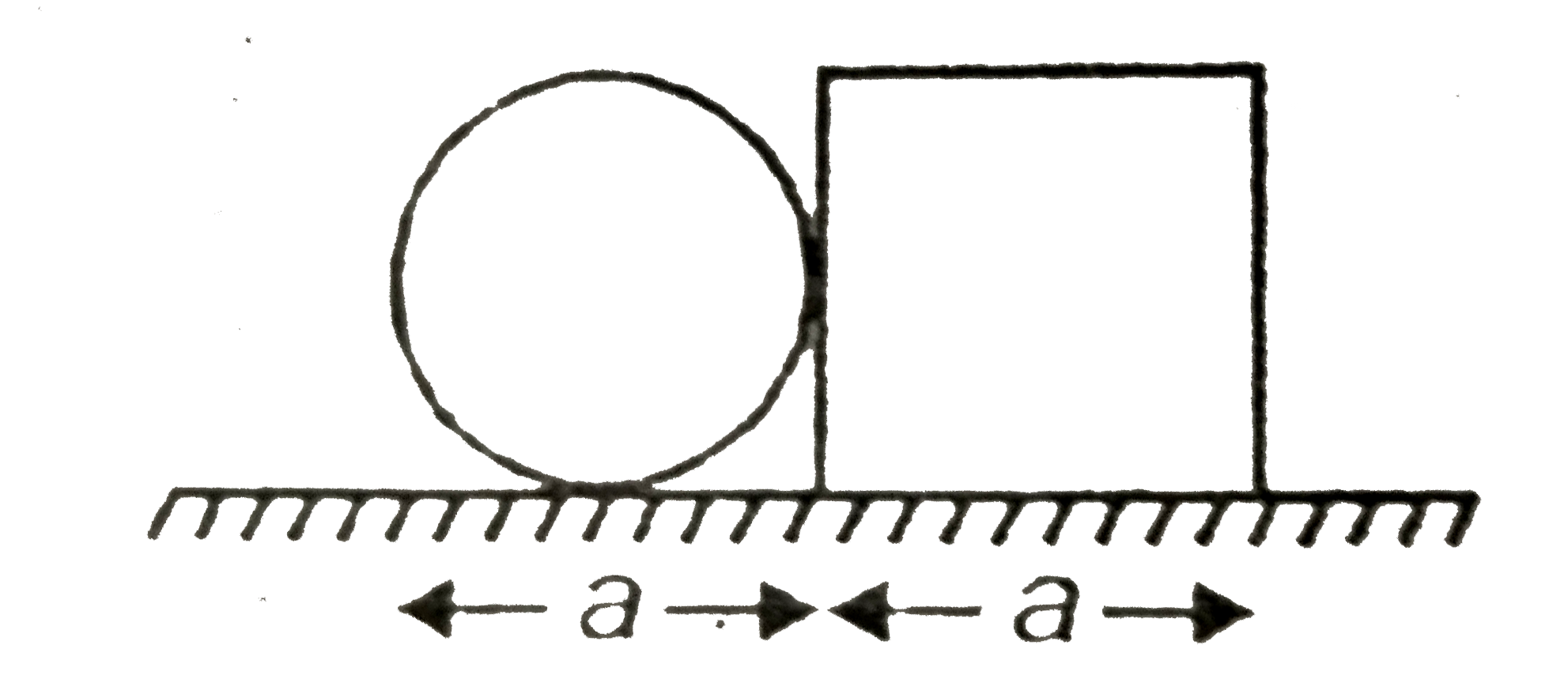
View SolutionA circular plate of diameter 'a' is kept in contact with a square plate of side a as shown. The density of the material and the thickness are same everywhere. The centre of mass of composite system will be

View SolutionA circular plate of diameter 'a' is kept in contact with a square plate of side a as shown. The density of the material and the thickness are same everywhere. The centre of mass of composite system will be
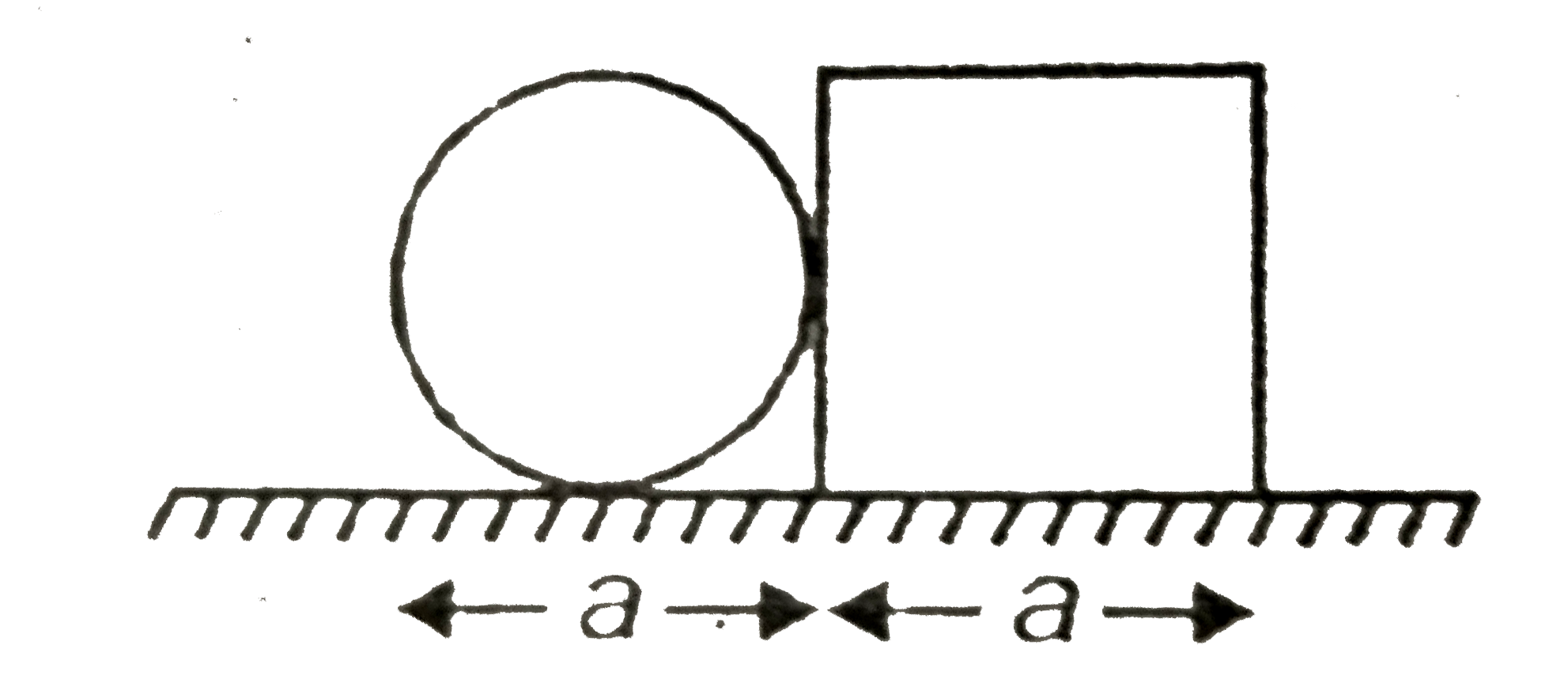
View SolutionA circular plate of diameter 'a' is kept in contact with a square plate of side a as shown. The density of the material and the thickness are same everywhere. The centre of mass of composite system will be
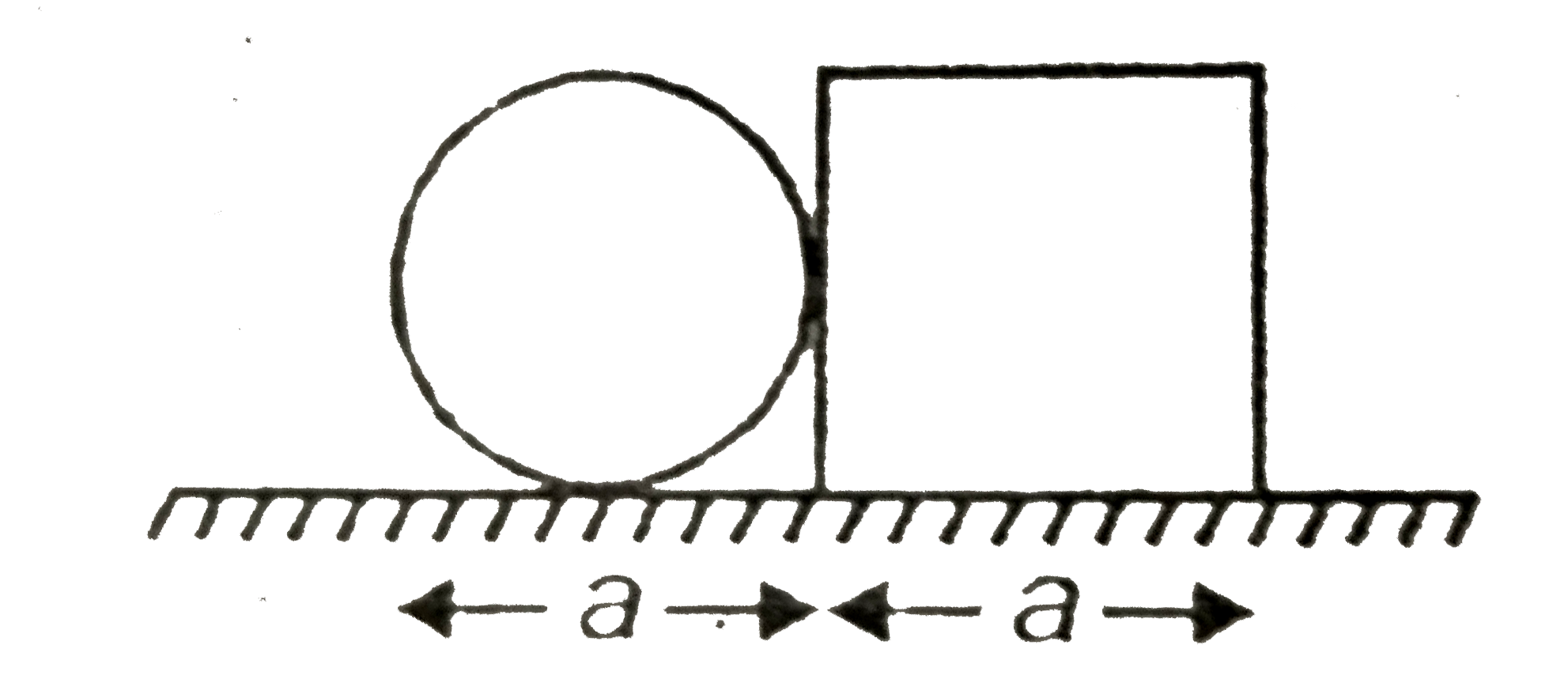
View SolutionCentre of mass of the system lies inside disc or square plate and why ?
View Solution
