A circular plate of uniform thickness has a diameter fo 56cm. A circular portion of diameter 42 cm is removed from one edge of the plate as shown in figure. Find the position of the centre of mass of the remaining portion.
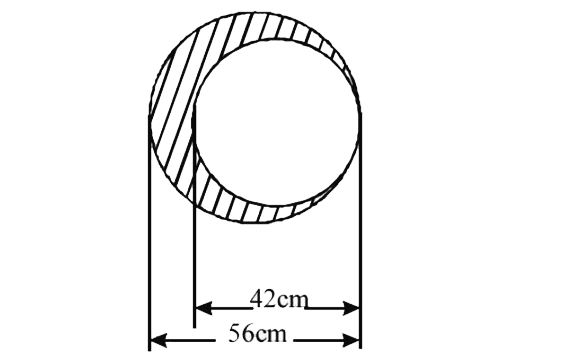
9 cm
7 cm
5 cm
4 cm
The correct Answer is:A
Initial area of the plane =π(28)2
Area of the removed plate (A1)=π(21)2
Area of the remaining plate (A2)=π(282−212)
=π(49×7)
Let d1andd2 be the distances of the centres of mass of the removed plate and remaining plate from the centre of the original plate.
d1=27−21=7cm
Since the plate is of uniform thickness, weight of the plate is proportional to the area .
[W−mg=v×ρg=Ahρg∴W∝A]
∴A1d1=A2d2
d2=(A1A2)d1=π(21)2π(49×7)×7=9cm
∴ It is at a distance of 9 cm from the centre of the original plate.
Topper's Solved these Questions
Similar Questions
A circular plate of unifrom thickness has a diameter of 56cm. A circular portion of diameter 42cm is removed from the edge of the plate as shown in Fig. Find the position of centre of mass of the remaining portion.
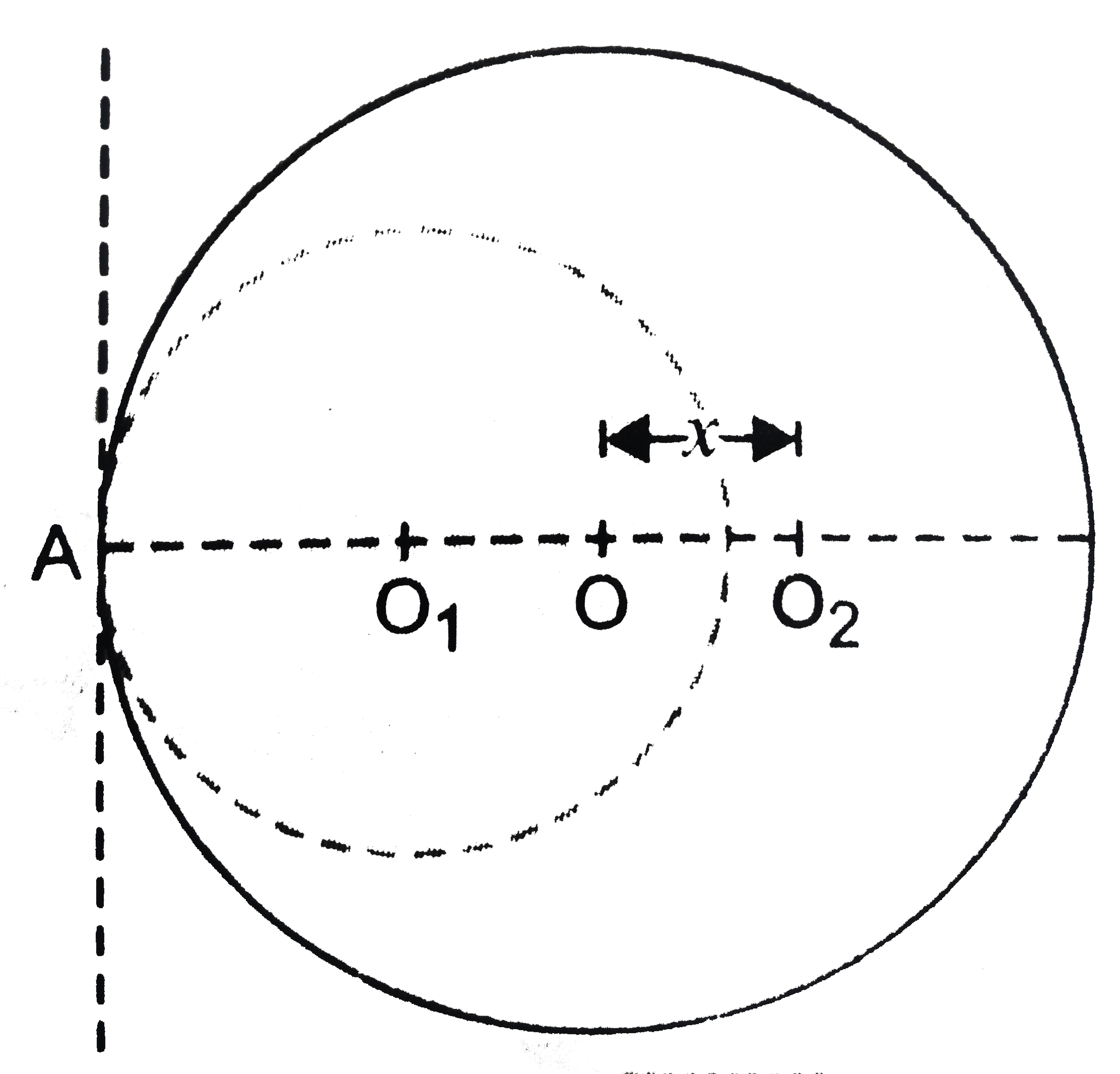
View SolutionA uniform circular plate of uniform thickness has a diameter of 56 cm. A circular portion of diameter 42 cm is removed from the edge of the plate. Find the position of the centre of mass of the remaining portion.
View Solution
Knowledge Check
A circular plate of uniform thickness has a diameter of 28cm. A circular portion of diameter 21cm is removed from the plate as shown. Ois the centre of mass of complete plate. The position of centre of mass of remaining portion will shift towards left from 'O' by
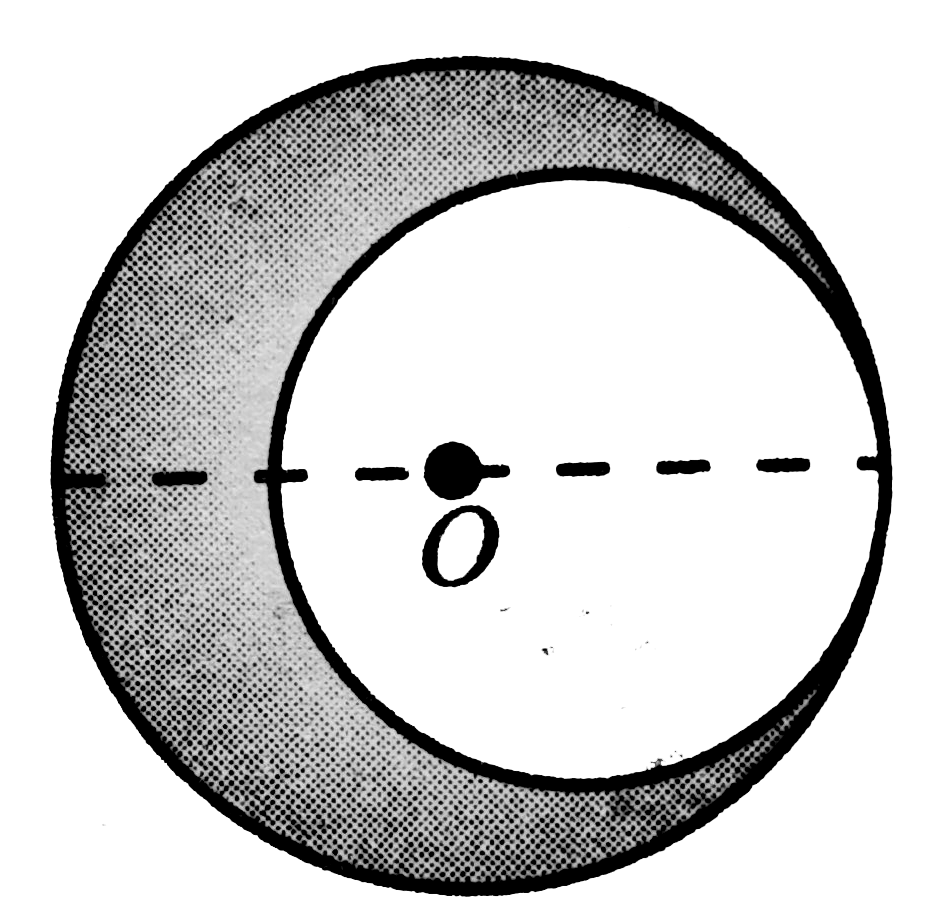 A5cmB9cmC4.5cmD5.5cm
A5cmB9cmC4.5cmD5.5cmA square plate of side 20cm has uniform thickness and density. A circular part of diameter 8cm is cut out symmetrically and shown in figure. The position of center of mass of the remaining potion is
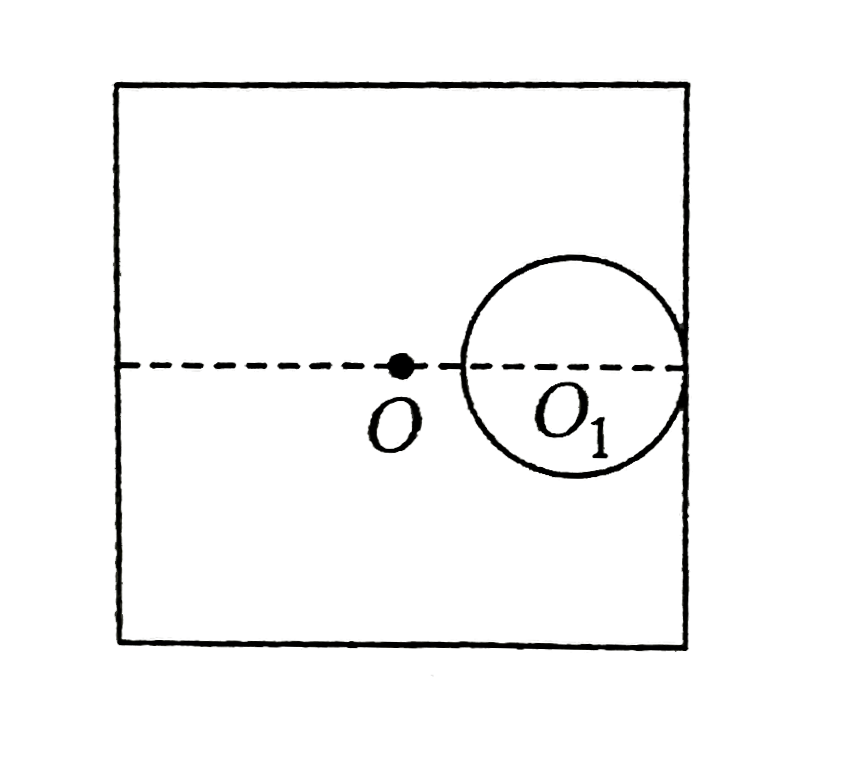 Aat O1Bat OC0.54 cm from O on the left hand sideDNone of the above
Aat O1Bat OC0.54 cm from O on the left hand sideDNone of the above
A disc of radius a2 is cut out from a uniform disc of radius a as shown in figure . Find the X Coordinate of centre of mass of remaining portion
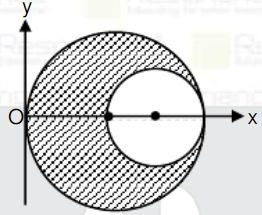
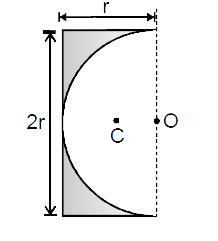
View SolutionA semicircular portion of radius 'r' is cut from a uniform rectangular plate as shown in figure. The distance of centre of mass 'C' of remaining plate, from point 'O' is
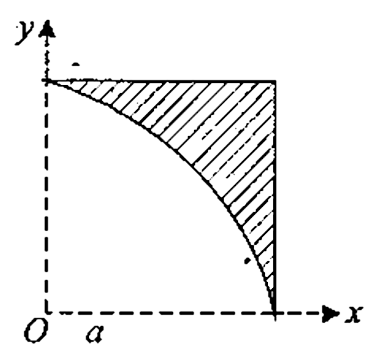
View SolutionFron a sqaure plate of side a,a quarter circular disc of radius a is removed as shown in figure. Find the coordinates of centre of mass of the remaining part.
View SolutionFrom a uniform circular disc of diameter d,a circular disc of diameter d/6 and having centre at a distance d/4 from the centre of the disc is scooped out. Determine the centre of mass of remaining portion.
View SolutionA circular grass plot 4m in diameter is cut by a straight path 1m wide, one edge of which passes through the centre of the plot. Area of the remaining portion is
View SolutionFrom a uniform circular disc of diameter D, a circular disc or hole of diameter D/6 and having its centre at a distance of D/4 from the centre of the disc is scopped out. Determine the centre of mass of the remaining portion.
View Solution
