A coin kept on a rotating gramophone disc just beging to slip if its centre is at a distance of 8 cm from the centre of the disc .The angular velocity of the gramophone disc is then doubled , Through what distance , the coin should be shifted towards the centre , so that the coin will just slip ?
2 cm
4 cm
6 cm
16 cm
The correct Answer is:C
To solve the problem step by step, we will analyze the forces acting on the coin when it is on the rotating gramophone disc.
Step 1: Understand the Initial Condition
The coin is initially at a distance r=8cm from the center of the disc. It is just about to slip, meaning the frictional force equals the required centripetal force for circular motion.
Step 2: Write the Equation for the Initial Condition
The centripetal force required to keep the coin moving in a circle is given by:
Fc=mω2r
where m is the mass of the coin, ω is the angular velocity, and r is the radius (8 cm in this case).
The frictional force f that prevents the coin from slipping is given by:
f=μmg
where μ is the coefficient of static friction and g is the acceleration due to gravity.
Since the coin is just about to slip, we have:
μmg=mω2r
Cancelling m from both sides, we get:
μg=ω2r
Step 3: Analyze the New Condition
When the angular velocity is doubled, the new angular velocity ω′=2ω. The coin will now slip at a new radius r′.
Step 4: Write the Equation for the New Condition
The centripetal force in the new condition can be expressed as:
F′c=mω′2r′=m(2ω)2r′=4mω2r′
Setting this equal to the frictional force, we have:
μmg=4mω2r′
Again cancelling m, we get:
μg=4ω2r′
Step 5: Relate the Two Conditions
From the first condition, we have:
μg=ω2r
Substituting r=8cm:
μg=ω2⋅8
Now substituting this into the second condition:
8=4r′
This implies:
r′=84=2cm
Step 6: Calculate the Shift Towards the Center
The coin needs to be shifted from r=8cm to r′=2cm. The distance x that the coin should be shifted is:
x=r−r′=8cm−2cm=6cm
Final Answer
The coin should be shifted 6 cm towards the center of the disc to just begin to slip again.
---
The coin is initially at a distance r=8cm from the center of the disc. It is just about to slip, meaning the frictional force equals the required centripetal force for circular motion.
The centripetal force required to keep the coin moving in a circle is given by:
Fc=mω2r
where m is the mass of the coin, ω is the angular velocity, and r is the radius (8 cm in this case).
f=μmg
where μ is the coefficient of static friction and g is the acceleration due to gravity.
μmg=mω2r
Cancelling m from both sides, we get:
μg=ω2r
When the angular velocity is doubled, the new angular velocity ω′=2ω. The coin will now slip at a new radius r′.
The centripetal force in the new condition can be expressed as:
F′c=mω′2r′=m(2ω)2r′=4mω2r′
Setting this equal to the frictional force, we have:
μmg=4mω2r′
Again cancelling m, we get:
μg=4ω2r′
From the first condition, we have:
μg=ω2r
Substituting r=8cm:
μg=ω2⋅8
8=4r′
This implies:
r′=84=2cm
The coin needs to be shifted from r=8cm to r′=2cm. The distance x that the coin should be shifted is:
x=r−r′=8cm−2cm=6cm
The coin should be shifted 6 cm towards the center of the disc to just begin to slip again.
Topper's Solved these Questions
- BOOK - MARVEL PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - TEST YOUR GRASP-11videos
CIRCULAR MOTION
- BOOK - MARVEL PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - TEST YOUR GRASP-21videos
CIRCULAR MOTION
- BOOK - MARVEL PUBLICATIONCHAPTER - ATOMS, MOLECULES AND NUCLEI EXERCISE - TEST YOUR GRASP30videos
ATOMS, MOLECULES AND NUCLEI
- BOOK - MARVEL PUBLICATIONCHAPTER - COMMUNICATION SYSTEMS EXERCISE - TEST YOUR GRASP -2010videos
COMMUNICATION SYSTEMS
Similar Questions
A gramophone disc rotates at 60 rpm. A coin of mass 18 g is placed at a distance of 8 cm from the centre. Calculate the centrifugal force on the coin. Take π2=9.87.
View SolutionA gramophone disc rotates at 60 rpm . A coin of mass 18 g is placed at a distance of 8 cm from the centre . Calculate centrifugal force on the coin . Take π2=9.87 .
View Solution
Knowledge Check
- Question 1 - Select One
A coin placed on a rotating turntable just slips if it is placed at a distance of 16 cm from the centre. If the angular velocity of the turnable is doubled, it will just slip at a distance ( in cm) of
A1 cmB2 cmC4 cmD8 cm - Question 2 - Select One
A coin placed on a rotating turn table just slips if it is placed at a distance of 8 cm from the centre. If angular velocity of the turn table is doubled . It will just slip at a distance of
A1 cmB2 cmC4 cmD8 cm - Question 3 - Select One
A coin placed on a rotating gramophone disc, remains at rest when it is at a distance of 9 cm from its centre ,the angular velocity of the disc is then tripled .At what distance from the centre ,the coin should be placed , so that it will remain at rest ?
A2cmB1 cmC3 cmD6 cm
Moment of inertia of a disc about an axis parallel to diameter and at a distance x from the centre of the disc is same as the moment of inertia of the disc about its centre axis. The radius of disc is R. The value of x is
View SolutionA coins kept on a horizontal rotating disc has its centre at a distance of 0.25 m from the axis of rotation of the disc .If μis0.2, then the angular velocity of the disc at which the coion will slip off, (g=9.8m/s2)
View SolutionA coin is place at a distance 9 cm from centre on a rotating turn table slip. If the angular velocity of the turn table is tripled, then the distance ofn the coin from the centre will be
View SolutionA coin placed on a rotating table just slip when it is placed at a distance 4 r from the centre, on doubling the angular velocity of the table , the coin will just slip now the coin is at a distance from centre is
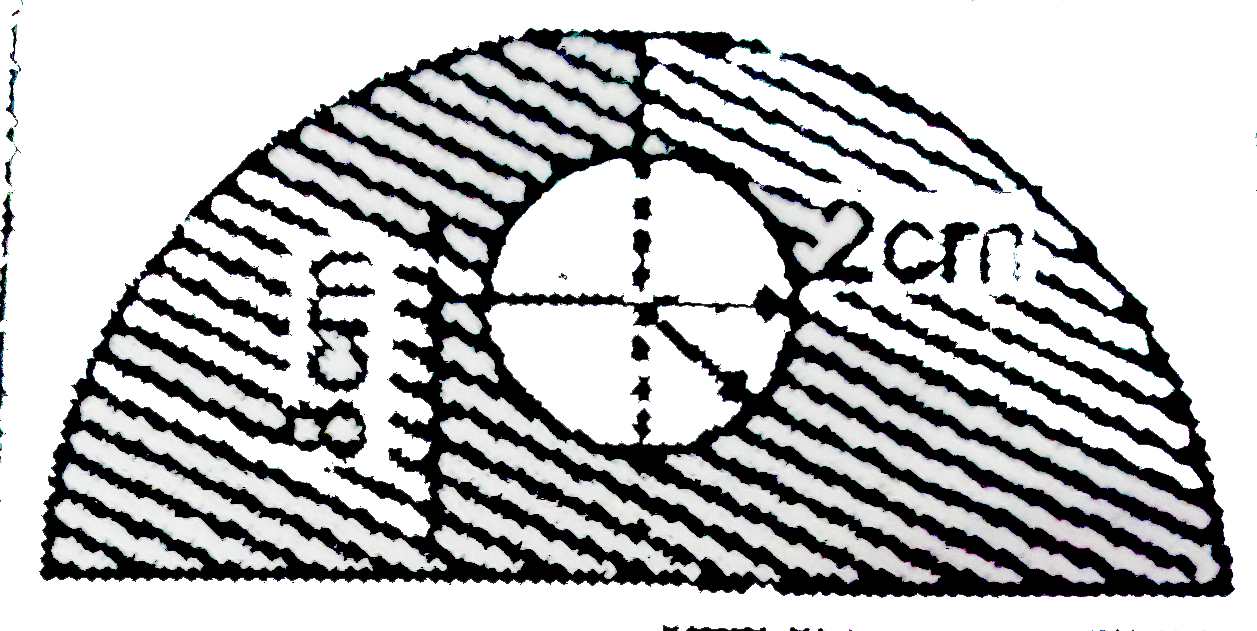
View SolutionIn the figure shown a hole of radius 2 cm is made in a semicircular disc of radius 6π at a distance 8 cm from the centre C of the disc. The distance of the centre of mass of this system from point C is :
 View Solution
View Solution

