Question

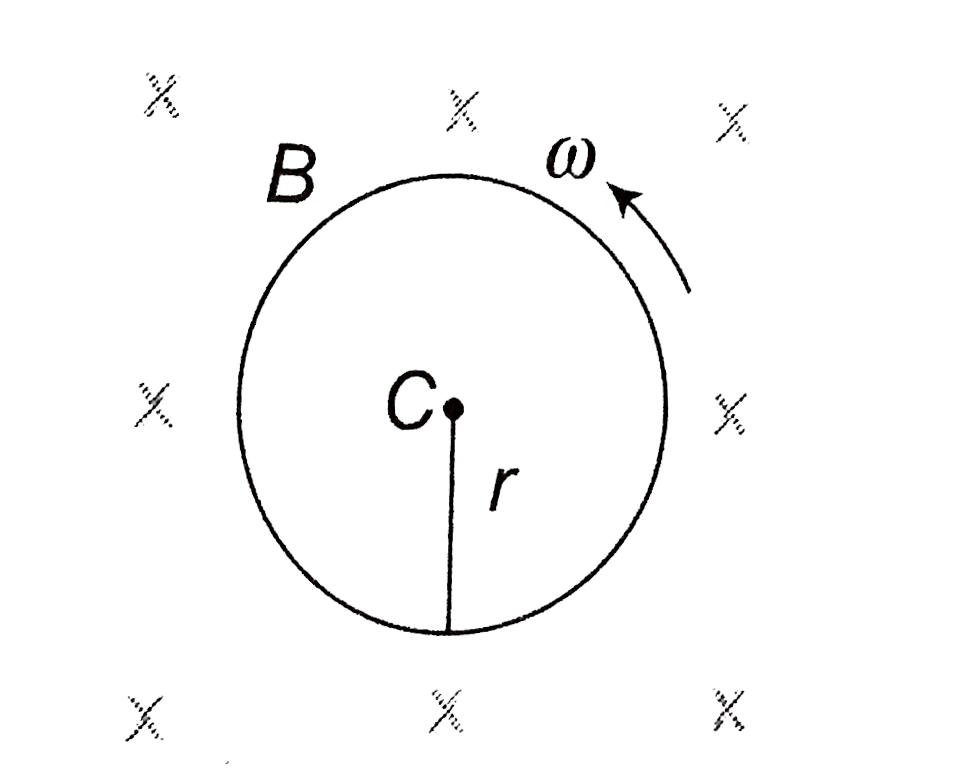
A conducting disc of radius ${\displaystyle r}$ spins about its axis an angular velocity ${\displaystyle \omega }$ . There is a uniform magnetic field of magnitude ${\displaystyle B}$ perpendicular to the plane of the disc ${\displaystyle C}$ is the centre of the disc.
(i) No emf is induced in the disc
(ii) The potential difference between ${\displaystyle C}$ and the rim is ${\displaystyle 1/2B{r}^2\omega }$
${\displaystyle C}$ is at a higher potential than the rim
(iv) Current flows between ${\displaystyle C}$ and the rim

Options

A. `(i), (iii)`
B. `(ii), (iii)`
C. `(ii), (iv)`
D. none

Answer

B

Explanation

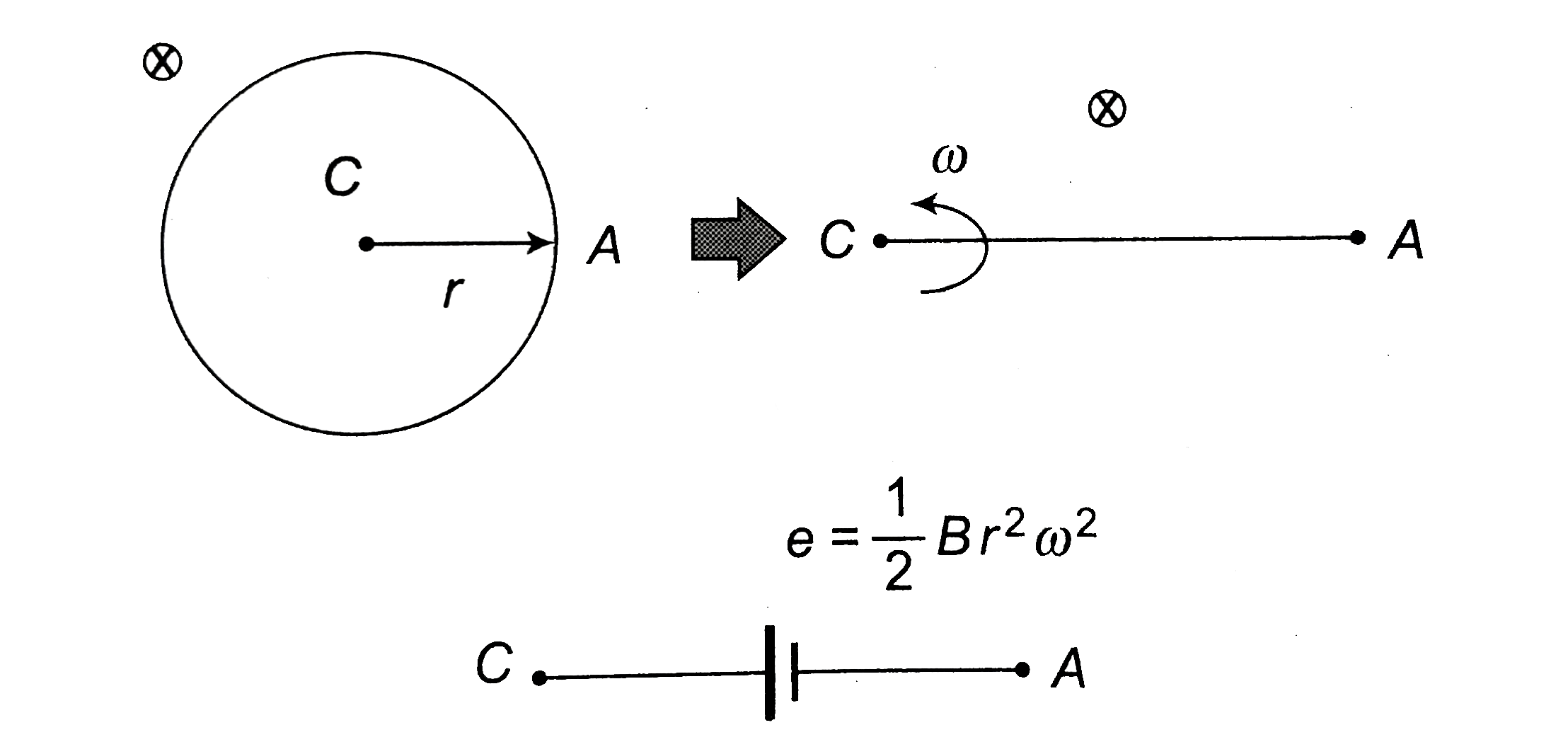
A conducting disc is equivalent to a rod
`V_(C)-V_(A)=(1)/(2)Br^(2)omega`