A conducting sphere of radius 0.1 m has a uniform positive charge density of 1.8μC/m2 on its surface. The electric field in V/m in free space at a radial distance of 0.2m from a point on the surface of the sphere is given by
6.0×10−8/ε0
2.0×10−7/ε0
4.5×10−7/ε0
6.0×10−6/ε0
The correct Answer is:B
To solve the problem, we need to calculate the electric field at a distance of 0.2 m from the surface of a conducting sphere with a given surface charge density. Here’s a step-by-step solution:
Step 1: Understand the Problem
We have a conducting sphere of radius R=0.1m with a uniform surface charge density σ=1.8μC/m2. We need to find the electric field at a distance of 0.2m from a point on the surface of the sphere.
Step 2: Calculate the Total Charge on the Sphere
The total charge Q on the sphere can be calculated using the formula:
Q=σ×A
where A is the surface area of the sphere given by A=4πR2.
Substituting the values:
A=4π(0.1)2=4π(0.01)=0.04πm2
Now, substituting σ:
Q=(1.8×10−6C/m2)×(0.04π)≈2.26×10−7C
Step 3: Determine the Distance from the Center of the Sphere
The distance from the center of the sphere to the point where we want to calculate the electric field is:
d=R+0.2=0.1+0.2=0.3m
Step 4: Use the Formula for Electric Field Due to a Point Charge
For a point charge, the electric field E at a distance d is given by:
E=kQd2
where k=14πϵ0.
Step 5: Substitute the Values into the Electric Field Formula
Substituting Q and d:
E=(1/(4πϵ0))⋅(2.26×10−7)(0.3)2
Calculating (0.3)2=0.09:
E=(2.26×10−7)0.09⋅(4πϵ0)
Step 6: Simplify the Expression
Since we are looking for the electric field in terms of ϵ0, we can simplify:
E=2.26×10−70.09⋅14πϵ0
Calculating 2.26×10−70.09≈2.51×10−6:
E≈2.51×10−64πϵ0
Step 7: Final Result
Thus, the electric field at a distance of 0.2 m from the surface of the sphere is:
E≈2.51×10−64πϵ0V/m
We have a conducting sphere of radius R=0.1m with a uniform surface charge density σ=1.8μC/m2. We need to find the electric field at a distance of 0.2m from a point on the surface of the sphere.
The total charge Q on the sphere can be calculated using the formula:
Q=σ×A
where A is the surface area of the sphere given by A=4πR2.
A=4π(0.1)2=4π(0.01)=0.04πm2
Now, substituting σ:
Q=(1.8×10−6C/m2)×(0.04π)≈2.26×10−7C
The distance from the center of the sphere to the point where we want to calculate the electric field is:
d=R+0.2=0.1+0.2=0.3m
For a point charge, the electric field E at a distance d is given by:
E=kQd2
where k=14πϵ0.
Substituting Q and d:
E=(1/(4πϵ0))⋅(2.26×10−7)(0.3)2
Calculating (0.3)2=0.09:
E=(2.26×10−7)0.09⋅(4πϵ0)
Since we are looking for the electric field in terms of ϵ0, we can simplify:
E=2.26×10−70.09⋅14πϵ0
Calculating 2.26×10−70.09≈2.51×10−6:
E≈2.51×10−64πϵ0
Thus, the electric field at a distance of 0.2 m from the surface of the sphere is:
E≈2.51×10−64πϵ0V/m
Topper's Solved these Questions
- ELECTROSTATICSBOOK - TARGET PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - COMPETITIVE THINKING
124 Videos
- ELECTROSTATICSBOOK - TARGET PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - EVALUATION TEST
14 Videos
- ELECTROSTATICSBOOK - TARGET PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - EVALUATION TEST
14 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - TARGET PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EVALUATION TEST
8 Videos
- GRAVITATIONBOOK - TARGET PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EVALUATION TEST
24 Videos
Similar Questions
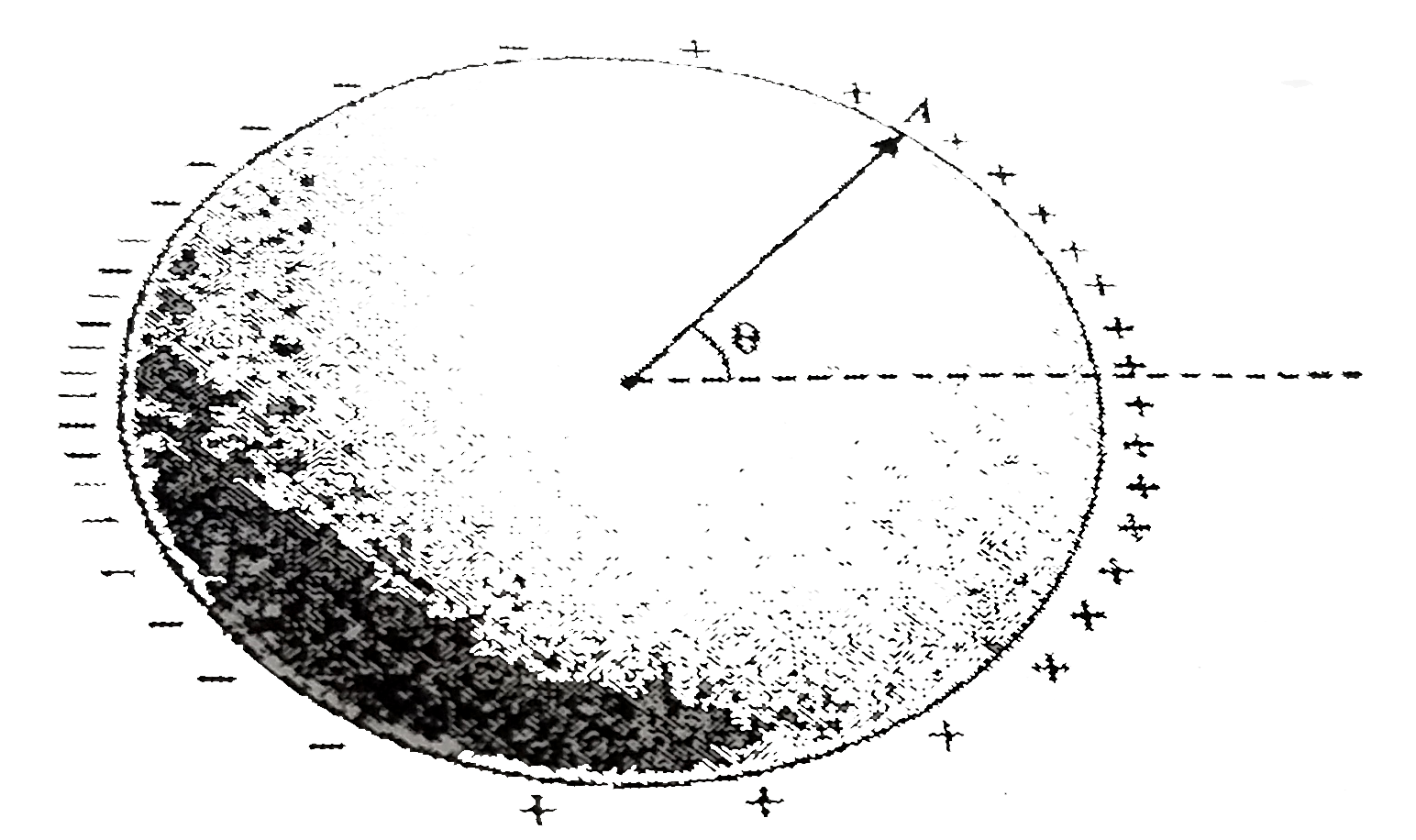
A solid conducting sphere of radius R is placed in a uniform electric field E as showo in figure. Due to electric field non uniform surface charges are induced on the surface of the sphere. Consider a point A on the surface of sphere at a polar angle θ from the direction of electric field as shown in figure. Find the surface density of induced charges at point A in terms of electric field and polar angle θ : lt brgt
View SolutionA uniformaly charged conducting sphere of diameter 2.4 m has a surface charge density of 80.0μCm−2. What is the total electric flux leaving the surface of the sphere ?
View Solution
Knowledge Check
An isolated conducting sphere of radius 0.1m placed in vacuum carries a positive charge of 0.1μC. Find the electric intensity at a point at a distance 0.2 m from the centre of the sphere.
A2.25×102N/CB2.25×104N/CC2.52×102N/CD2.52×104N/CAn insulating solid sphere of radius R has a uniformly positive charge density ρ. As a result of this uniform charge distribution there is a finite value of electric potential at the centre of the sphere, at the surface of the sphere and also at a point out side the sphere. The electric potential at infinity is zero.
Statement-1: When a charge 'q' is taken from the centre to the surface of the sphere, its potential energy changes by qρ3∈0
Statement-2 : The electric field at a distance r(r<R) from the centre of the the sphere is ρr3∈0A(a) Statement 1 is true, Statement 2 is true, Statement 2 is not the correct explanation of statement 1.B(b) Statement 1 is true Statement 2 is false.C(c) Statement 1 is false Statement 2 is true.D(d) Statement 1 is true, Statement 2 is true, Statement 2 is the correct explanation of Statement 1An insulating solid sphere of radius R has a uniformaly positive charge density ρ. As a result of this uniform charge distribution there is a finite value of electric potential at the centre of the sphere, at the surface of the sphere and also at a point out side the sphere. The electric potential at infinity is zero.
Statement-1: Wgen a charge 'q' is taken from the centre to the surface of the sphere, its potential energy charges by qρ3∈0
Statement-2 : The electric field at a distance r(r<R) from the centre of the the sphere is ρr3∈0AStatement-1 is true, Statement-2 is true and Statement-2 is the correct explanation of Statement-1BStatement-1 is true, Statement-2 is true and Statement-2 is not the correct explanation of Statement-1CStatement-1 is true, Statement-2 is falseDStatement-1 is false, Statement-2 is true
A uniformly charged conducting sphere of 4.4 m diameter has a surface change density of 60μCm−2. The charge on the sphere is
View SolutionA hollow insulated conducting sphere is given a positive charge of 10μC . What will be the electric field at the centre of the sphere it is radius is 2 metres ?
View SolutionTwo conducting spheres of radii r1 and r2 are charged to the same surface charge density . The ratio of electric field near their surface is
View SolutionA charge produce an electric field of 1 N/C at a point distant 0.1 m from it. The magnitude of charge is
View SolutionA hollow insulated conducting sphere of radius 2m, is given a charge of 5 μC. What will be the electric field at the centre of the sphere?
View Solution
