A conductor of resistance 25Ω of diameter 0.2 mm is connected to a battery of 200 V . Calculate the current density for the conductor.
To solve the problem of calculating the current density in a conductor with a given resistance and diameter, we can follow these steps:
Step 1: Calculate the Current (I)
We know the relationship between voltage (V), current (I), and resistance (R) is given by Ohm's Law:
I=VR
Given:
- Voltage, V=200V
- Resistance, R=25Ω
Substituting the values:
I=200V25Ω=8A
Step 2: Calculate the Cross-sectional Area (A)
The cross-sectional area of a cylindrical conductor can be calculated using the formula:
A=πr2
First, we need to find the radius (r) from the diameter. The diameter is given as 0.2mm, which we convert to meters:
Diameter=0.2mm=0.2×10−3m=0.0002m
Thus, the radius is:
r=Diameter2=0.0002m2=0.0001m
Now we can calculate the area:
A=π(0.0001m)2=π(0.00000001m2)≈3.14×10−8m2
Step 3: Calculate the Current Density (J)
Current density (J) is defined as the current (I) per unit area (A):
J=IA
Substituting the values we found:
J=8A3.14×10−8m2≈2.54×108A/m2
Final Answer
The current density for the conductor is approximately:
J≈2.54×108A/m2
We know the relationship between voltage (V), current (I), and resistance (R) is given by Ohm's Law:
I=VR
Given:
- Voltage, V=200V
- Resistance, R=25Ω
I=200V25Ω=8A
The cross-sectional area of a cylindrical conductor can be calculated using the formula:
A=πr2
First, we need to find the radius (r) from the diameter. The diameter is given as 0.2mm, which we convert to meters:
Diameter=0.2mm=0.2×10−3m=0.0002m
Thus, the radius is:
r=Diameter2=0.0002m2=0.0001m
A=π(0.0001m)2=π(0.00000001m2)≈3.14×10−8m2
Current density (J) is defined as the current (I) per unit area (A):
J=IA
Substituting the values we found:
J=8A3.14×10−8m2≈2.54×108A/m2
The current density for the conductor is approximately:
J≈2.54×108A/m2
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS
35 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - TOUGH AND TRICKY PROBLEMS
23 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Chapter Practice Test
15 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
A circular conductor of uniform resistance per unit length, is connected to a battery of 4 V. The total resistance of the conductor is 4ω. The net magnetic field at the centre of the conductor is
 Aμ02B8μ03C2μ0Dzero
Aμ02B8μ03C2μ0Dzero
If 240 J heat is generated in a conductor of resistance 50 Ω when 0.2 A current is passed through it, then for how long is the current flowing through the conductor?
View SolutionA resistance of 25Ω is connected to a 12V battery. Calculate the heat energy in joules generated per minute.
View SolutionA solenoid of resistance 50Ω and inductance 80 H is connected to a 200 V battery, How long will it take for the current to reach 50% of its final equlibrium value ? Calculate the maximum enargy stored ?
View SolutionA bulb of resistance 5Ω is connected to a battery of emf 4.2 V and internal resistance 1Ω. Find the current through the bulb.
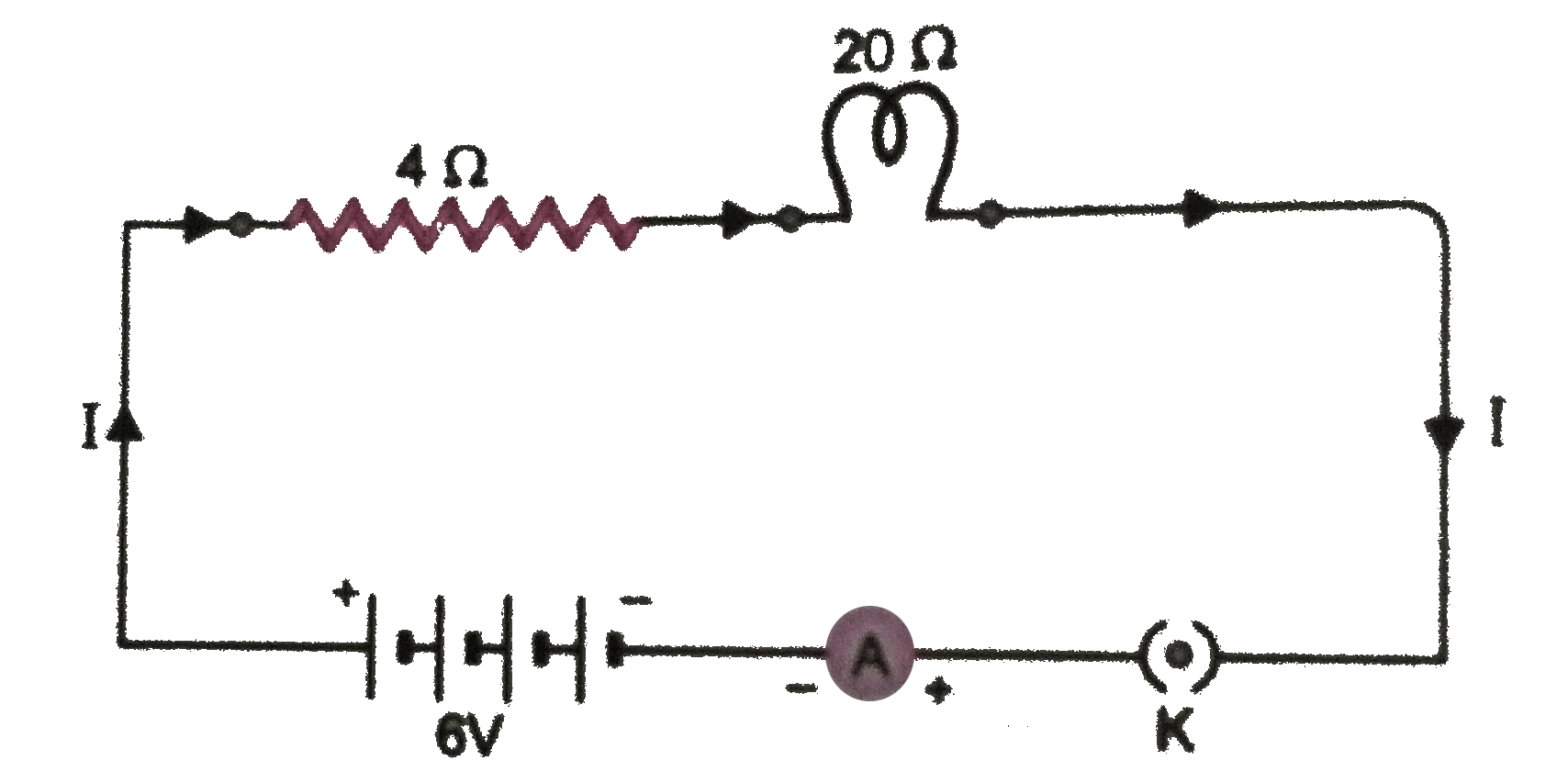
View SolutionAn electric lamp, whose resistance is 20Ω and a conductor of 4Ω resistance are connected to a 6V battery as shown in (Fig. 3.18) Calculate.
(a) the total resistance of the circuit,
(b) the current through the circuit, and
( c) the potential difference across the electric lamp and the conductor.
 .
.
View SolutionAn electric lamp whose resistance is 8 Ω and a conductor of 2 Ω resistance are connected in series to a 5 volt battery . Calculate( a) the total resistance of the circuit (b) the current through the circuit (c) the potential difference across the electric lamp and the conductor?
View SolutionA capacitor of 2.0μF is connected to a battery of 2V through a resistance of 10kΩ. Calculate (i) the initial current in the circuit and (ii) the current after 0.02s.
View Solution
