A constant temperature , the volume of a gas is to be decreased by 4 % The pressure must be increased by
0.04
0.0416
0.08
0.0386
The correct Answer is:B
To solve the problem of how much the pressure must be increased when the volume of a gas is decreased by 4% at constant temperature, we can use the ideal gas law, which states that PV=constant.
1. Understand the relationship: At constant temperature, the product of pressure (P) and volume (V) remains constant. This can be expressed as:
P1V1=P2V2
where P1 and V1 are the initial pressure and volume, and P2 and V2 are the final pressure and volume.
2. Express the volume change: The problem states that the volume is decreased by 4%. Therefore, the new volume V2 can be expressed as:
V2=V1−0.04V1=0.96V1
3. Substitute into the equation: Substitute V2 into the equation P1V1=P2V2:
P1V1=P2(0.96V1)
4. Cancel V1: Since V1 is common on both sides (assuming V1≠0), we can cancel it:
P1=0.96P2
5. Rearranging the equation: Rearranging gives us:
P2=P10.96
6. Calculate the change in pressure: The change in pressure can be found by calculating P2−P1:
P2−P1=P10.96−P1
=P1(10.96−1)
=P1(1−0.960.96)=P1(0.040.96)
7. Calculate the fractional change in pressure: The fractional change in pressure is given by:
P2−P1P1=0.040.96=124
8. Convert to percentage: To find the percentage increase in pressure:
Percentage Increase=(124)×100≈4.16%
Conclusion:
Thus, the pressure must be increased by approximately 4.16%.
P1V1=P2V2
where P1 and V1 are the initial pressure and volume, and P2 and V2 are the final pressure and volume.
V2=V1−0.04V1=0.96V1
P1V1=P2(0.96V1)
P1=0.96P2
P2=P10.96
P2−P1=P10.96−P1
=P1(10.96−1)
=P1(1−0.960.96)=P1(0.040.96)
P2−P1P1=0.040.96=124
Percentage Increase=(124)×100≈4.16%
Thus, the pressure must be increased by approximately 4.16%.
Topper's Solved these Questions
- KINETIC THEORY OF GASES ANDRADIATIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - KINETIC THEORY OF GASES ANDRADIATIONEXERCISE - MHT CET Corner
40 Videos
- KINETIC THEORY OF GASES ANDRADIATIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - KINETIC THEORY OF GASES ANDRADIATIONEXERCISE - Exercise 1
132 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHT BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHT EXERCISE - MHT CET Corner
24 Videos
- MAGNETIC EFFECT OF ELECTRIC CURRENTBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENTEXERCISE - MHT CET CORNER
20 Videos
Similar Questions
Knowledge Check
At constant temperature if the pressure of an ideal gas is increased by 10% then its volume must decrease by
A0.1B0.0901C0.1525D0.1101A sample of gas has a volume of V1 litre at temperature t1.∘C. When the temperature of the gas is changed to t2.∘C at constant pressure, then the volume of the gas was found to increase by 10%. The percentage increase in temperature is
A0.1B(10+2730t1)%C20%D(0.1+t−11)%If the volume of an ideal gas decreased by 5% at constant pressure, the increase of pressure is
A0.05B0.0518C0.0526D0.0541
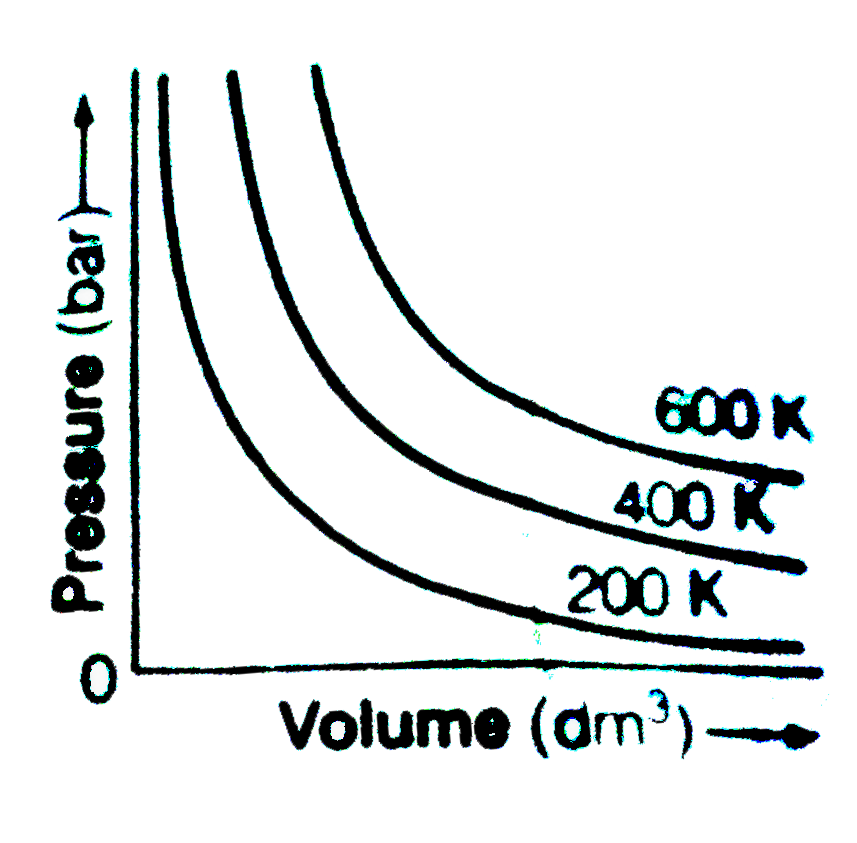
The variation of pressure with volume of the gas at different temperatures can be graphically represented as shown in figure. On the basis of this graph answer the following question.
(i) How will the volume of a gas change if its pressure is increased at constant temperature ?
(ii) At a constant pressure, how will the volume of a gas change if the temperature is increased from 200 K to 400 K ?
View SolutionIf the temperature of a gas is increased by 1K at constant pressure its volume increase by 0.0035 of the initial volume. The temperature of the gas is
View SolutionThe temperature of a gas contain in a closed vessel increased by 20C when the pressure is increased by 2% the intial temperature of the gas is
View SolutionAt constant temperature on increasing the pressure of a gas by 5% will decrease its volume by -
View SolutionAt constant volume, the temperature (in .∘C) of certain mass of a gas is increased by 20%. The pressure of gas:
View Solution
