A cricket ball of mass 150 g moving at a speed of 25 ms−1 is brought to rest by a player in 0.03 s. Find the average force applied by the player.
To solve the problem of finding the average force applied by a player to bring a cricket ball to rest, we can follow these steps:
Step 1: Identify the given values
- Mass of the cricket ball (m) = 150 g = 0.15 kg (since 1 g = 0.001 kg)
- Initial velocity (u) = 25 m/s
- Final velocity (v) = 0 m/s (since the ball is brought to rest)
- Time taken (t) = 0.03 s
Step 2: Use the formula for acceleration
We can use the equation of motion:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time taken
Substituting the known values:
0=25+a×0.03
Step 3: Solve for acceleration (a)
Rearranging the equation gives:
a×0.03=−25
a=−250.03
a=−833.33m/s2
Step 4: Calculate the average force
Using Newton's second law:
F=m×a
Substituting the values of mass and acceleration:
F=0.15kg×(−833.33m/s2)
F=−125N
Step 5: Interpret the result
The negative sign indicates that the force is applied in the opposite direction of the motion of the ball. Therefore, the magnitude of the average force applied by the player is:
|F|=125N
Final Answer
The average force applied by the player is 125 N.
---
- Mass of the cricket ball (m) = 150 g = 0.15 kg (since 1 g = 0.001 kg)
- Initial velocity (u) = 25 m/s
- Final velocity (v) = 0 m/s (since the ball is brought to rest)
- Time taken (t) = 0.03 s
We can use the equation of motion:
v=u+at
Where:
- v = final velocity
- u = initial velocity
- a = acceleration
- t = time taken
0=25+a×0.03
Rearranging the equation gives:
a×0.03=−25
a=−250.03
a=−833.33m/s2
Using Newton's second law:
F=m×a
Substituting the values of mass and acceleration:
F=0.15kg×(−833.33m/s2)
F=−125N
The negative sign indicates that the force is applied in the opposite direction of the motion of the ball. Therefore, the magnitude of the average force applied by the player is:
|F|=125N
The average force applied by the player is 125 N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - ICSECHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - 3(D)
17 Videos
- LAWS OF MOTIONBOOK - ICSECHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - 3(D) (Mutliple Choice type)
2 Videos
- LAWS OF MOTIONBOOK - ICSECHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - 3(C) Multiple Choice type
4 Videos
- ICSE ANNUAL EXAMINATION -2020BOOK - ICSECHAPTER - ICSE ANNUAL EXAMINATION -2020EXERCISE - SECTION-II
45 Videos
- LIGHTBOOK - ICSECHAPTER - LIGHTEXERCISE - TOPIC 2 Spherical Mirrors (4 Marks Questions )
9 Videos
Similar Questions
A cricket ball of mass 100 g moving with a speed of 30ms−1 is brought to rest by a player in 0.03 s. Find the average force applied by the player.
View SolutionA cricket ball of mass 100 g moving with a speed of 30ms−1 is brought to rest by a player in 0.03 s. Find the change in momentum of ball.
View SolutionA cricket ball of mass 200 gm moving with velocity 15 m/s is brought to rest by a player in 0.05 sec. What is the impulse of the ball and average force exerted by player ?
View SolutionA player caught a criket ball of mass 150 g moving at the rate of 20ms−1 . If the catching process the completed in 0.1s , the force of the blow exerted by the ball on the hands of the player is
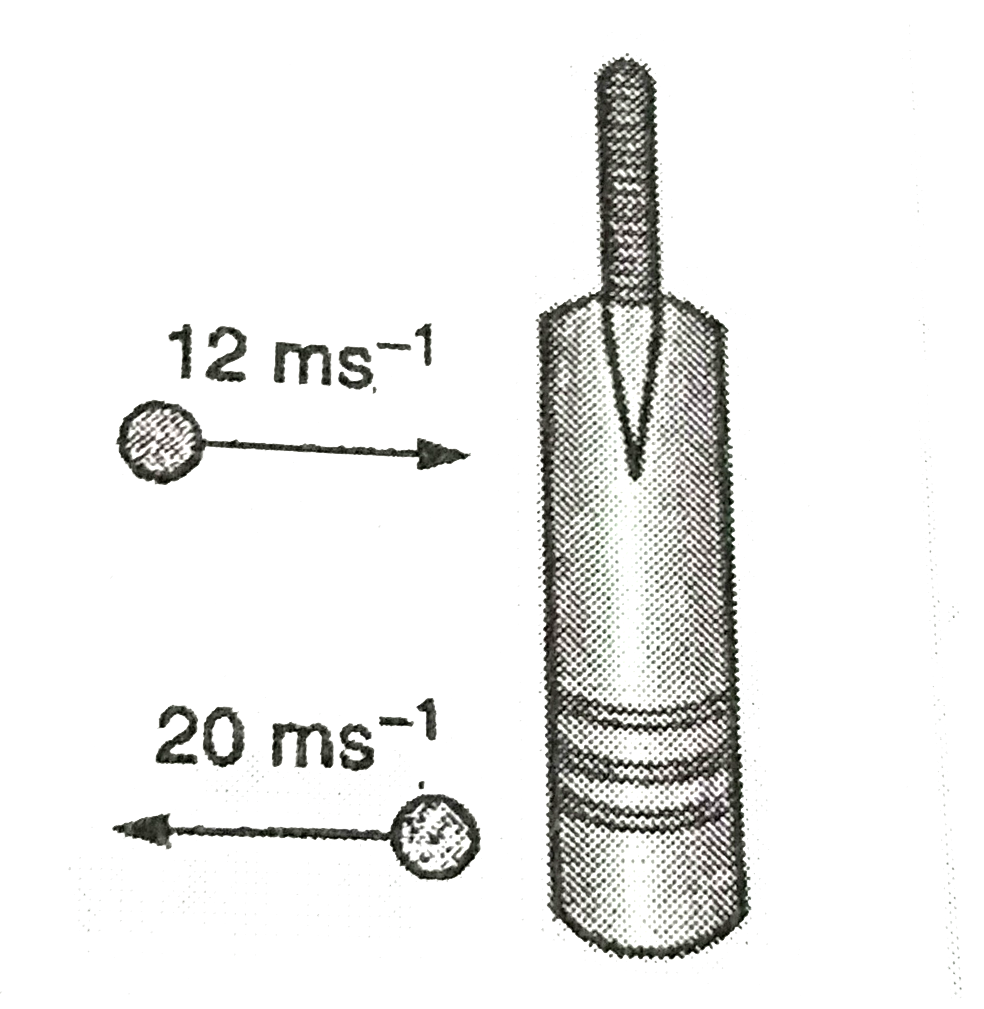
View SolutionA cricket ball of mass 150 g is moving with a speed of 12ms−1 and is hit by a bat so that the ball is turned back with velocity of 20ms−1 if duration of contact between bat and ball is 0.01 s then:
View SolutionA train moving with a velocity of 20 m s−1' is brought to rest by applying brakes in 5 s. Calculate the retardation.
View SolutionA player caught a cricket ball of mass 150g moving at a rate of 20m/s. If the catching process is completed in 0.1s, the force of the blow exerted by the ball on the hand of the player is equal to
View SolutionA golf player hits a golf ball of mass 100 g and imparts it a speed of 50 m/s. If golf stick remains in contact with the ball for 0.01 s, then the average force exerted by the golf stick on the ball.
View SolutionA ball of mass 150 g moving with an acceleration 20 m/s2 is hit by a force, which acts on it for 0.1 s. The impulsive force is
View Solution
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass m3 moving in vertical up wards direction with velocity v0 strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The time taken by the block bullet system to move from y=mgk (initial equilibrium position) to y=0 (natural length of spring) is (A represents the amplitude of motion)
View Solution
