A current I flows an infinitely long wire with cross section in the form of a semi - circular ring of radius R. The magnitude of the magnetic induction along its axis is :
μ0I2π2R
μ0I2πR
μ0I4πR
μ0Iπ2R
The correct Answer is:D
Current in a small element , dl=dθπI
Magnetic field due to the element
dB=μ04π2dIR
The component dBcosθ, of the field is cancelled by another opposite component.
Therefore,
B≠t=∫dBsinθ=μ0I2π2R0∫π0sinθdθ=μ0Iπ2R

Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - MCQs(d )
1 Videos
- MODERN PHYSICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MODERN PHYSICSEXERCISE - MCQ (One Correct Answer
1 Videos
- RAY AND WAVE OPTICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - RAY AND WAVE OPTICSEXERCISE - JEE Main And Advanced
209 Videos
Similar Questions
A current I flows in a long straight wire whose cross section is in the shape of a thin quarter ring of radius R. Find the induction of the magnetic field (B) at point O on the axis.

View SolutionA current I flows in a long straight wire with cross-section haviing the form of a thin half-ring of radius R (Fig). Find the induction of the magnitude field at the point O.

View Solution
Knowledge Check
A long straight wire is placed along the axis of a circuit ring of radius R. The mutual inductance of this system is
Aμ0R2Bμ0πR2Cμ02D0A wire is bent in the form of a circular arc with a straight portion AB. Magnetic induction at O when current flowing in the wire, is
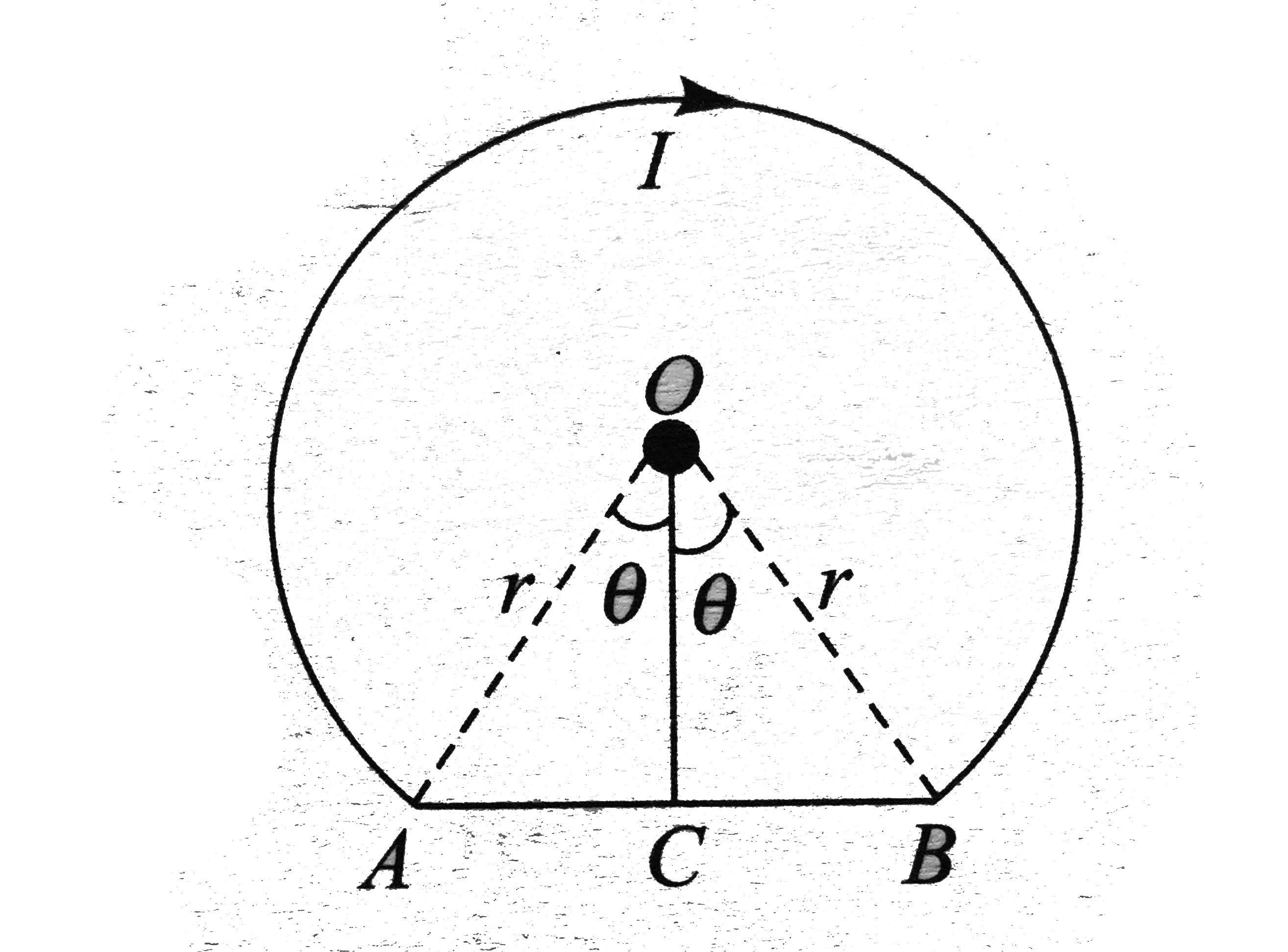 Aμ02r(π−θ+tanθ)Bμ0I2πR(π+θ−tanθ)Cμ0I2πR(π−θ+tanθ)Dμ0I2πR(−tanθ+π−θ)
Aμ02r(π−θ+tanθ)Bμ0I2πR(π+θ−tanθ)Cμ0I2πR(π−θ+tanθ)Dμ0I2πR(−tanθ+π−θ)A current I=IA flowing through an infinite long wire A1 divides between two infinite long wires A2 and A3 at junction B. The magnitude of the magnetic field at point O due to current through wires is :
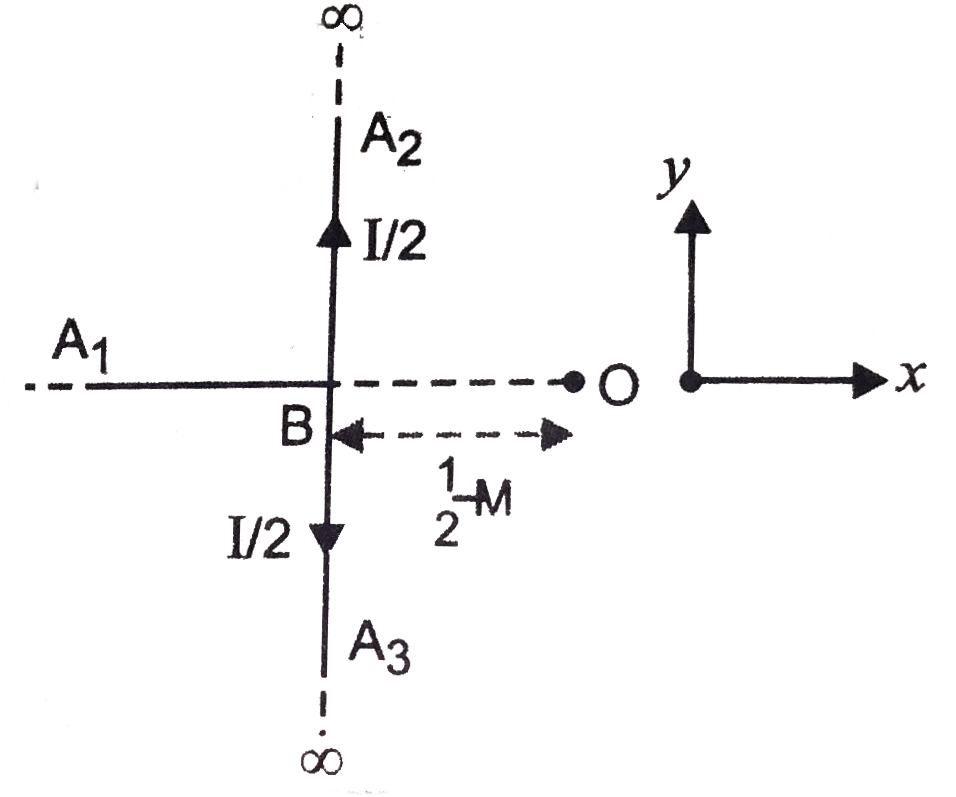 AzeroB2×10−7TC4×10−7TD8×10−7T
AzeroB2×10−7TC4×10−7TD8×10−7T
A direct current I flows in a long straight conductor whose cross section has the form of a thin halr-ring of radius R. The same current flows in the opposite direction along a thin conductor located on the axis of the first conductor. Find the magnetic induction froce between the given conductors reduced to a unit of their length.

View SolutionA current I flows in a long straight wire (into the plane of the paper) with cross-section having the from of a circular are of radius R a shown in the figure. Find the magnetic induction at the point O.
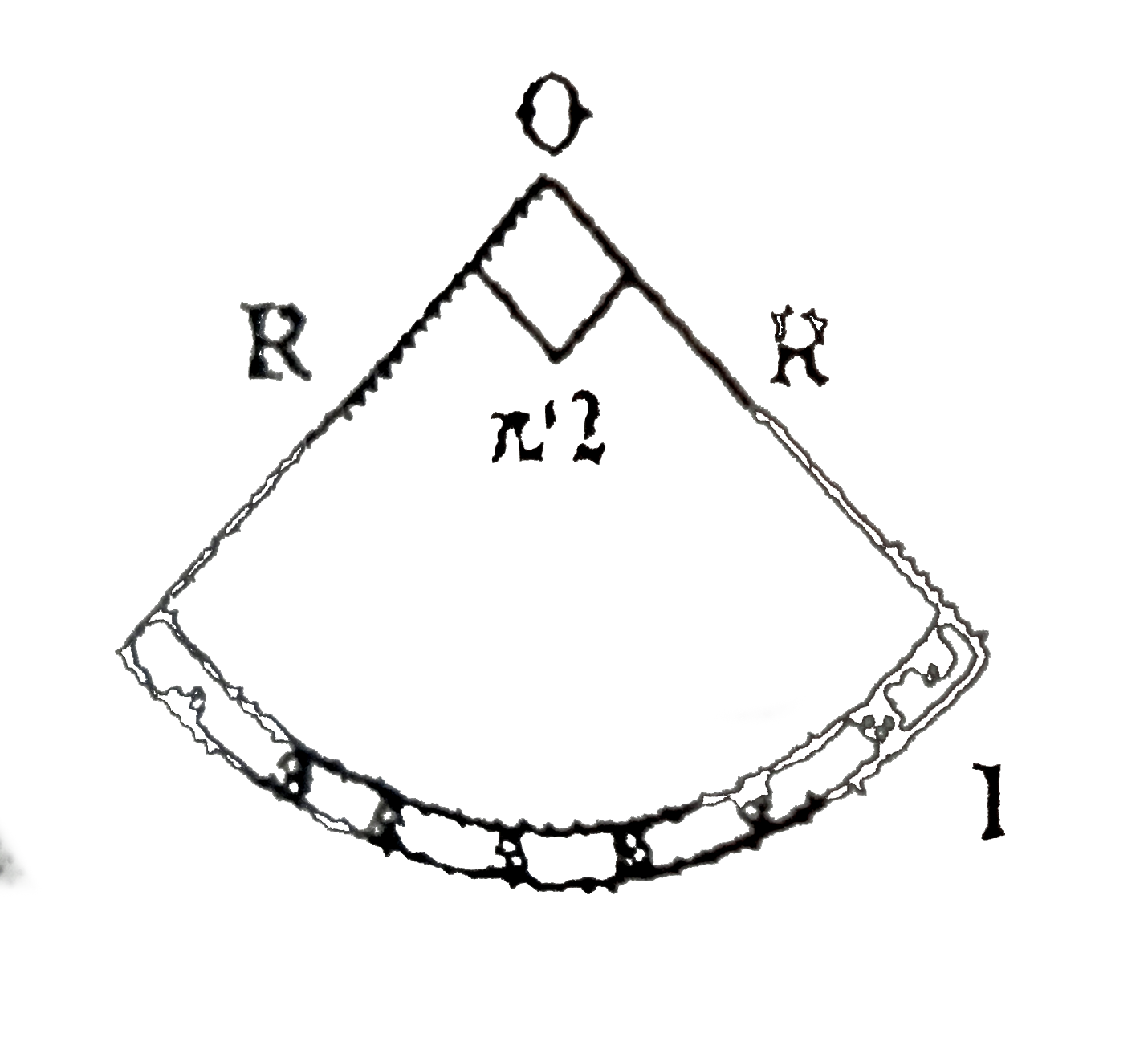
View SolutionA steady current I flows along an infinitely long straight wire with circular cross-section of radius R. What will be the magnetic field outside and inside the wire at a point r distance far from the axis of wire?
View SolutionA thin wire is bent in the form of a semi circular ring of radius R=0.9m carries a current I=1amp . The magnetic induction at a point P as shown in the fig. is given by nX10−7 Tesla. Find the value of n.[ln(√2+1)=0.9]

View SolutionA current 'I' flows along an infinitely long straight conductor. If 'r' is the perpendicular distance of a point from the lower end of the conductor, then the magnetic induction B is given by
View Solution
