A current of flows around a closed path in a circuit which is in horizontal plane as shown in the figure . The circuit consists of eight alternating arcs of 10 AAC CD due to the current at the center?
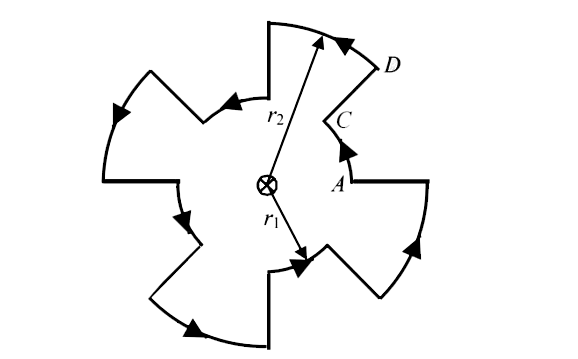
The correct Answer is:A, B, D
For finding the magnetic field produced by this circuit at the centre we can consider it to contain two semicircles of radii, . Since currents is flowing in the same direction , the magnetic field created by circular arcs will be in the same direction and therefore will be added.
:.
:. Directed outwards.
( right hand thumb rule)
(b) Force acting on a current carrying conductor placed in a magnetic field i is given by
(i) For force acting on the wire at the centre
in this case
:.
(ii) On arc due to current at the centre
on will be
The direction of this magnetic field on any small segment of will be tangential
:.
(iii) On segment .
Force on a small segment distant from
On integrating
directed downwards ( By Fleming left hand rule).

Topper's Solved these Questions
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - MCQs(d )1videos
MOVING CHARGES AND MAGNETISM
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MODERN PHYSICSEXERCISE - MCQ (One Correct Answer1videos
MODERN PHYSICS
- BOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - RAY AND WAVE OPTICSEXERCISE - JEE Main And Advanced209videos
RAY AND WAVE OPTICS
Similar Questions
A current of 10A flows around a closed path in a circuit which is int eh horizontal plne as shown in the figure. The circuit consists oif eight alternating arcs of radii r1=0.08m and r2=0.12m. Each subtends the same angle at the centre.
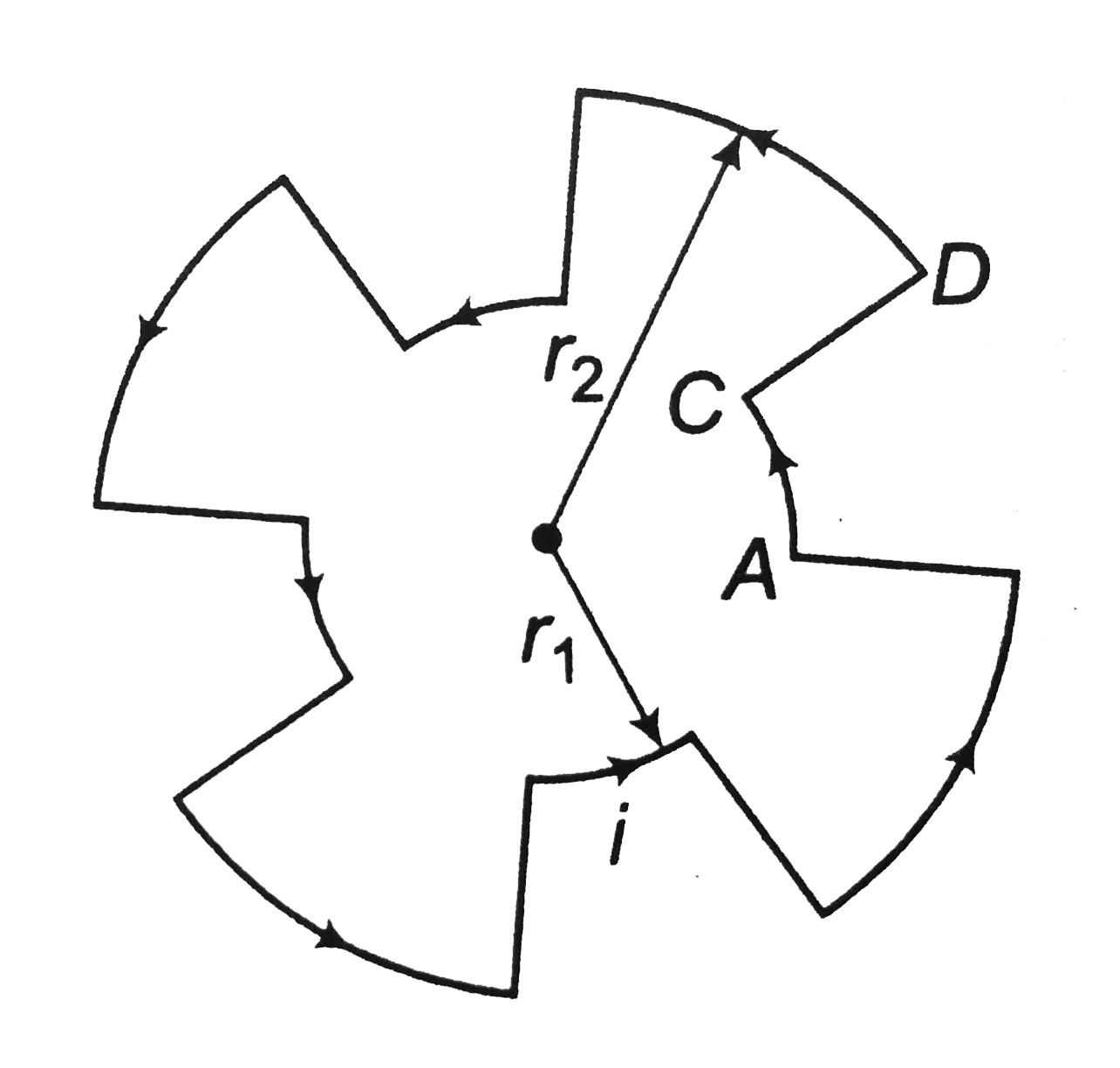
a. Find the magnetic field produced by this circuit at the centre.
b. An infinitely long straight wire carryin as current of 10A is passing through the centre of the above circuit vertically with the direction of the current being into the pane of the circuit. what is the force acting on the wire at the centredue to the curren in the circuit? What is the force acting on the arc AC and the straight segment CD due to the current at the centre?View SolutionA current of 4A flows around a circuit for 10s. How much charge flows past a point in the circuit in this time?
View Solution
Knowledge Check
- Question 1 - Select One
A current I flows around a closed path in the horizontal plane of circle as shown in the figure The path consists of eight arcs with alternating radii r and 2r . Each segment of arc subtends equal angle at the common centre P The magnetic field produced by current path at point P is
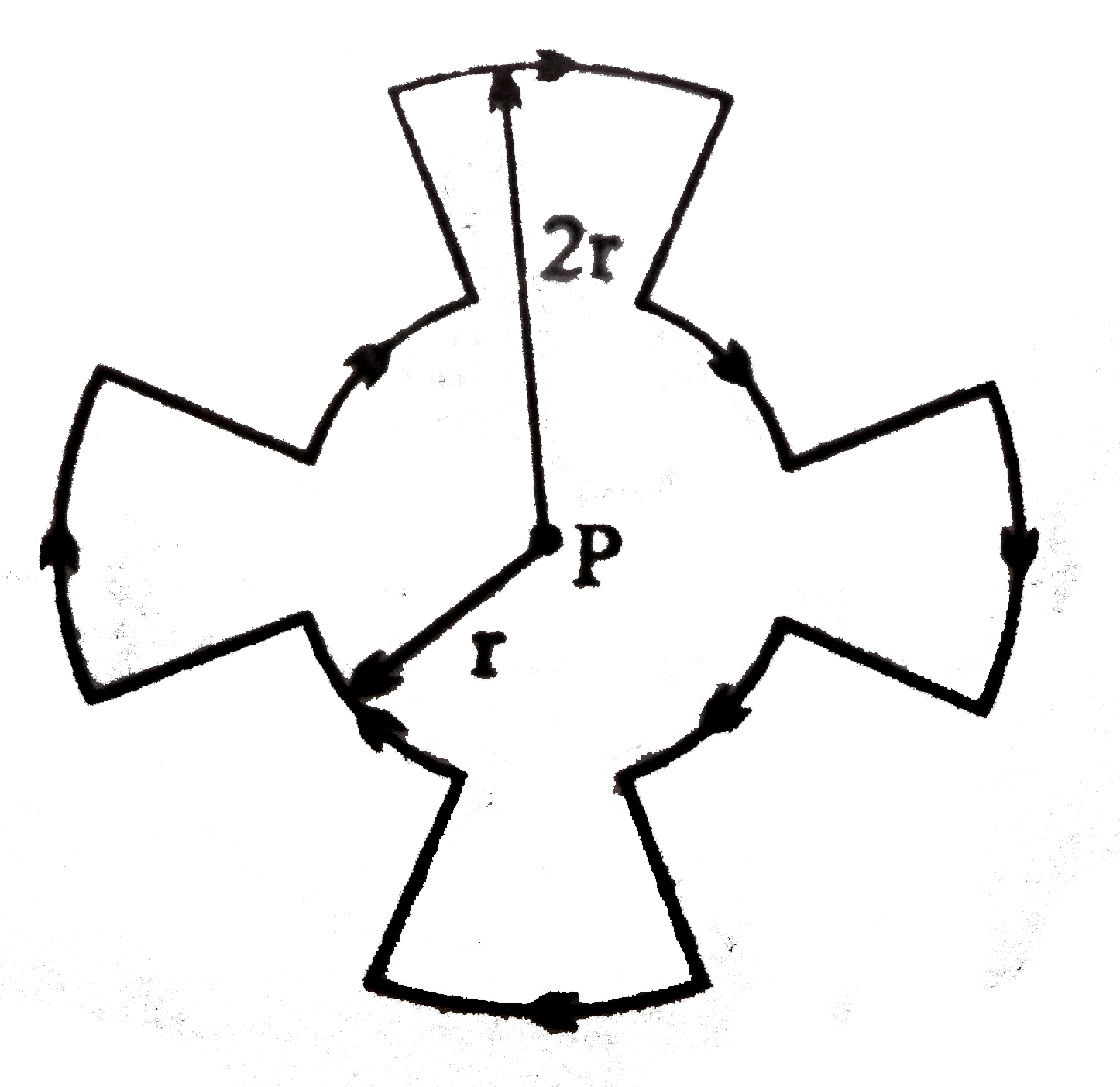 .A38μ0Ir , perpendicular to the plane of the paper and directed inward .B38μ0Ir , perpendicular to the plane of the paper and directed outward .C38μ0Ir , perpendicular to the plane of the paper and directed inward .D38μ0Ir , perpendicular to the plane of the paper and directed outward .
.A38μ0Ir , perpendicular to the plane of the paper and directed inward .B38μ0Ir , perpendicular to the plane of the paper and directed outward .C38μ0Ir , perpendicular to the plane of the paper and directed inward .D38μ0Ir , perpendicular to the plane of the paper and directed outward . - Question 2 - Select One
In the circuit shown in figure current in the circuit is
 A1.27AB2.23AC4.26AD4 A
A1.27AB2.23AC4.26AD4 A - Question 3 - Select One
In the figure shown, a current of 2 amperes flows in the circuit. The impedence of the circuit is-
 A170ΩB70ΩC130ΩD120Ω
A170ΩB70ΩC130ΩD120Ω
In a circle of radius 28 cm, an arc subtends an angle of 108∘ at the center. Find the length of the arc.
View SolutionThe current flowing through 10Ω resistor in the circuit shown in the figure is
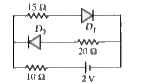 View Solution
View SolutionShown in the figure is an R−L circuit. Just after the key (K) is closed
 View Solution
View SolutionA current of 10A is flowing in a equilateral triangle of side length l=1M as shown in figure. The magnetic field at center of triangle is:
_2019_CBT6_E01_040_Q01.png) View Solution
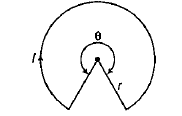
View SolutionA current I ampere flows in the loop having circular arc of radius r metre subtending an angle 6 as shown in figure. The magnetic field, at center of circle is
 View Solution
View Solution

