A cylinder is rolling without slipping on a horizontal plane P. The friction between the plank P and the cylinder is sufficient for no slipping. The coefficient of friction between the plank and the ground surface is zero. Initially, P is attached with a string S as shown in the figure. If the string is now burned, then.
the plank with start motion with a speed v along forward direction.
the plank will start motion with a speed v along backward direction.
the plank will remain static
linear velocity of the cylinder will decrease and angular velocity will increase.
The correct Answer is:C
( c) Initially there is no friction between cylinder and plank.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Problems Based On Mixed Concepts31videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Assertion Reasoning20videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - ROTATIONAL DYNAMICSEXERCISE - Work And Energy In Case Of Rotation22videos
ROTATIONAL DYNAMICS
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
- BOOK - A2ZCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Chapter Test30videos
THERMAL PROPERTIES OF MATTER
Similar Questions
If a solid cylinder rolls without slipping on an inclined plane of inclination 45° then the minimum coefficient of friction required to support pure rolling is
View SolutionShow that a cylinder will slip on an inclined plane if the coefficient of static friction between the plane and the cylinder is less than 13tanθ where θ is the angle of the inclination with the horizontal.
View Solution
Knowledge Check
- Question 1 - Select One
A sphere S rolls without slipping moving with a constant speed on a plank P. The friction between the upper surface of P and the sphere is sufficient to prevent slipping, while the lower surface of P is smooth and rest on the ground. Initially P is fixed on the ground by a pin N. If N is suddenly removed
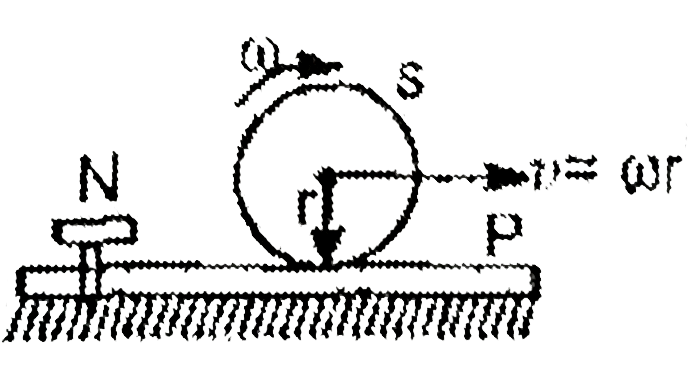 AS will begin to slip on PBP will begin to move backwardsCThe speed of S will decrease and its angular velocity with increaseDThere will be no change in the motion of S and P will still be in rest
AS will begin to slip on PBP will begin to move backwardsCThe speed of S will decrease and its angular velocity with increaseDThere will be no change in the motion of S and P will still be in rest - Question 2 - Select One
A solid cylinder is rolling without slipping on a plane having inclination θ and the coefficient of static friction μs. The relation between θ and μs is
Atanθ>3μsBtanθ≤3μsCtanθ<3μ2sDNone of these - Question 3 - Select One
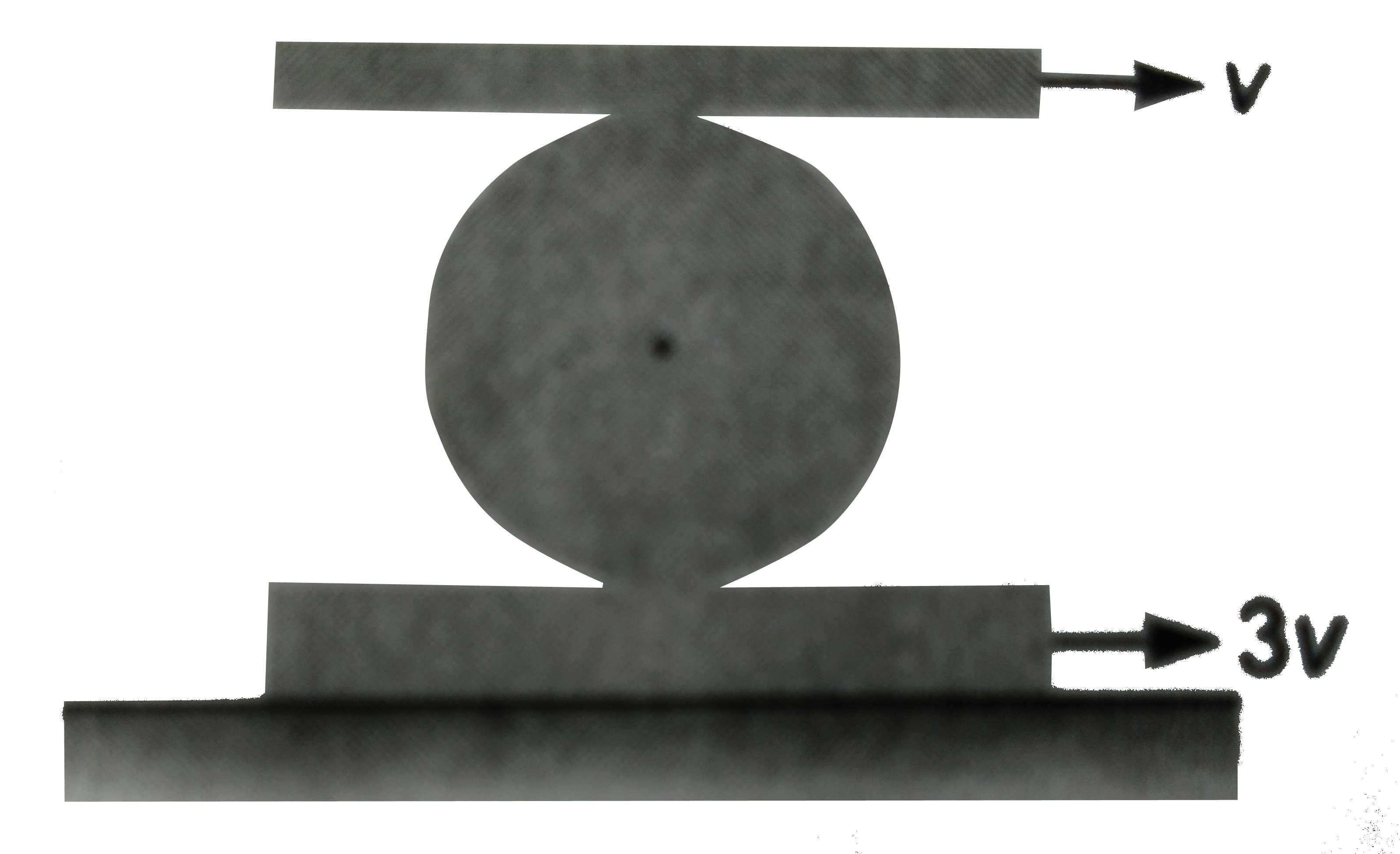
A cylinder of radius R is to roll without slippoing between two planks as shown in the figure. ThenAangular velocity of the cylinder is vR counter clockwiseBangular velocity of the cylinder is 2vR clockwiseCvelocity of centre of mass of the cylinder is v towards leftDvelocity of centre of mass of the cylinder is 2v towards right.
If a solid cylinder rolls without slipping on an inclined plane of inclination 45°, then the minimum coefficient of friction required to support pure rolling is
View SolutionA small block of mass 'm' is placed on a plank of mass 'M'. The block is connected to plank with the help of a light string passing over a light smooth pulley as shown in figure. The co-efficient of static friction between the block and plank is μ.
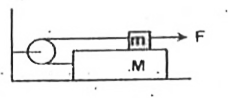
The co-efficient of friction between the plank and the horizontal surface is zero. What maximum horizontal force F applied on the block of mass m can make the block and plank not to slide relatively?View SolutionA solid cylinder is rolling down on an inclined plane of angle θ. The minimum value of the coefficient of friction between the plane and the cylinder to allow pure rolling
View SolutionA cylinder of radius R and mass M rolls without slipping down a plane inclined at an angle θ. Coeff. of friction between the cylinder and the plane is μ. For what maximum inclination θ, the cylinder rolls without slipping ?
View SolutionA solid cylinder rolls without slipping down a 30∘ slope. The minimum coefficient of friction needed to prevent slipping, will be
View Solution

