A cylindrical vessel of radius R=1m and height H=3m is filled with an ideal liquid up to a height of h=2 m. the contaienr with liquid is rotated about its central vertical axis such that the liquid just rises to the brim. Calculate the angular speed (ω) of the container.
The correct Answer is:ω=2√10rads−1
Step by step video, text & image solution for A cylindrical vessel of radius R=1m and height H=3m is filled with an ideal liquid up to a height of h=2 m. the contaienr with liquid is rotated about its central vertical axis such that the liquid just rises to the brim. Calculate the angular speed (omega) of the container. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A cylindrical vessel of diameter 0.3m and height 0.6m is filled two thirds with a liquid of specific gravity 0.8. The vessel is rotated about its axis. a. Determine the speed of rotation when the liquid just starts spilling. b.Find the speed of rotation when the base is just visible. c. What is the percentate of liquid left in the vessel?
View SolutionA cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
View Solution
Knowledge Check
A circular cylinder of radius R and height H is filled with water to a height (2/3)H. It starts rotating about its axis with constantly increasing angular speed. Choose the correct alternatives.
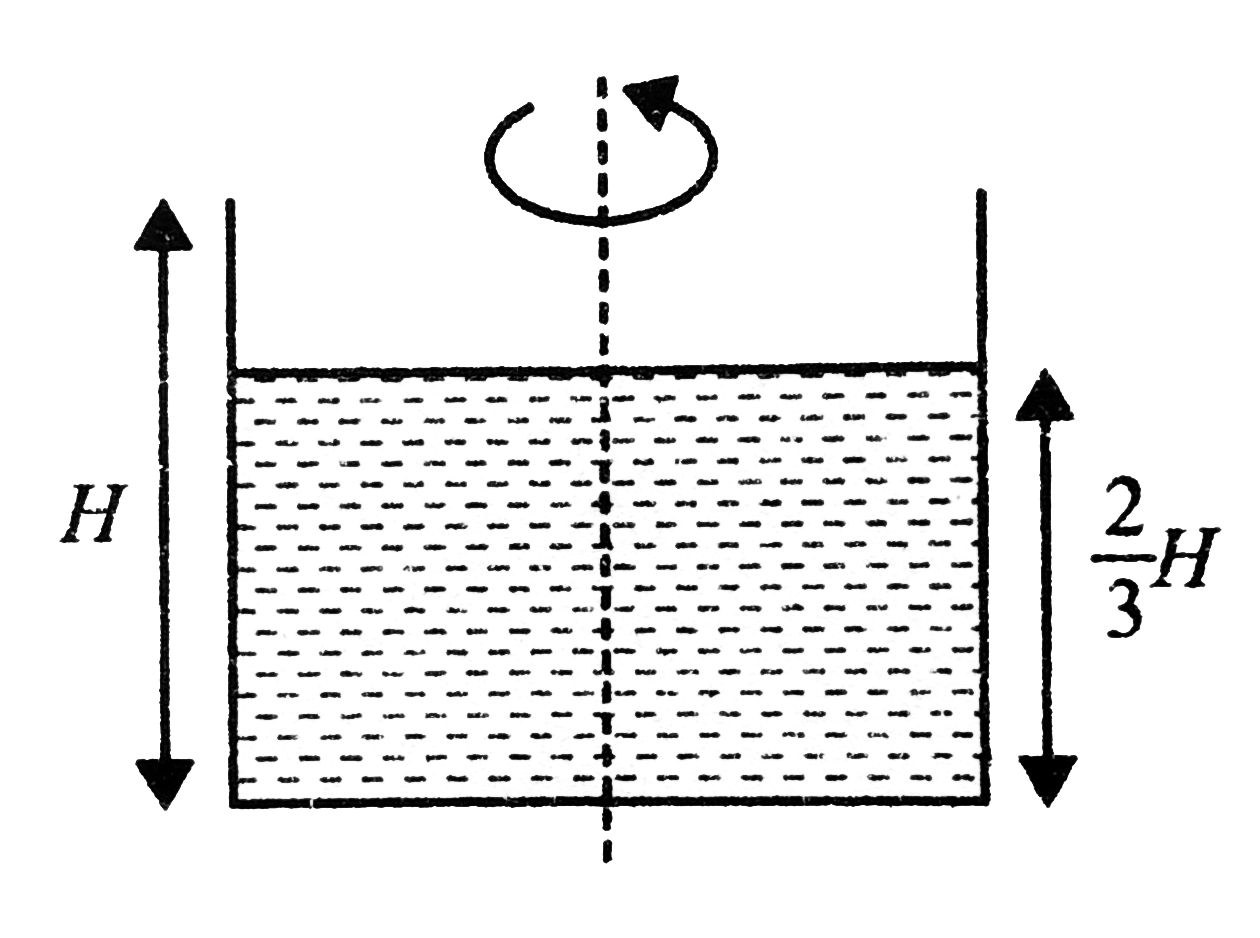 AAt all speeds, shape of the free surface is parabolloid.BThe free surface touches first the brim of the cylinder and then the base of the cylinder.CThe free surface cannot touch the base without spilling water.DThe free surface touches the brim as well as the base at the same instant.
AAt all speeds, shape of the free surface is parabolloid.BThe free surface touches first the brim of the cylinder and then the base of the cylinder.CThe free surface cannot touch the base without spilling water.DThe free surface touches the brim as well as the base at the same instant.A cylindrical vessel of radius r is filled with a homogeneous liquid upto a height h. It is found that the force exerted by the liquid column on the bottom of the cylinder is equal to the force exerted by the liquid on the sides of the cylinder. What is the relation between r and h?
Ar=h2Br=hCr=2hDr=32hA liquid is filled in a spherical container of radius R up to a height h. At this position the liquid surface at the end is also horizontal. The contact angle is
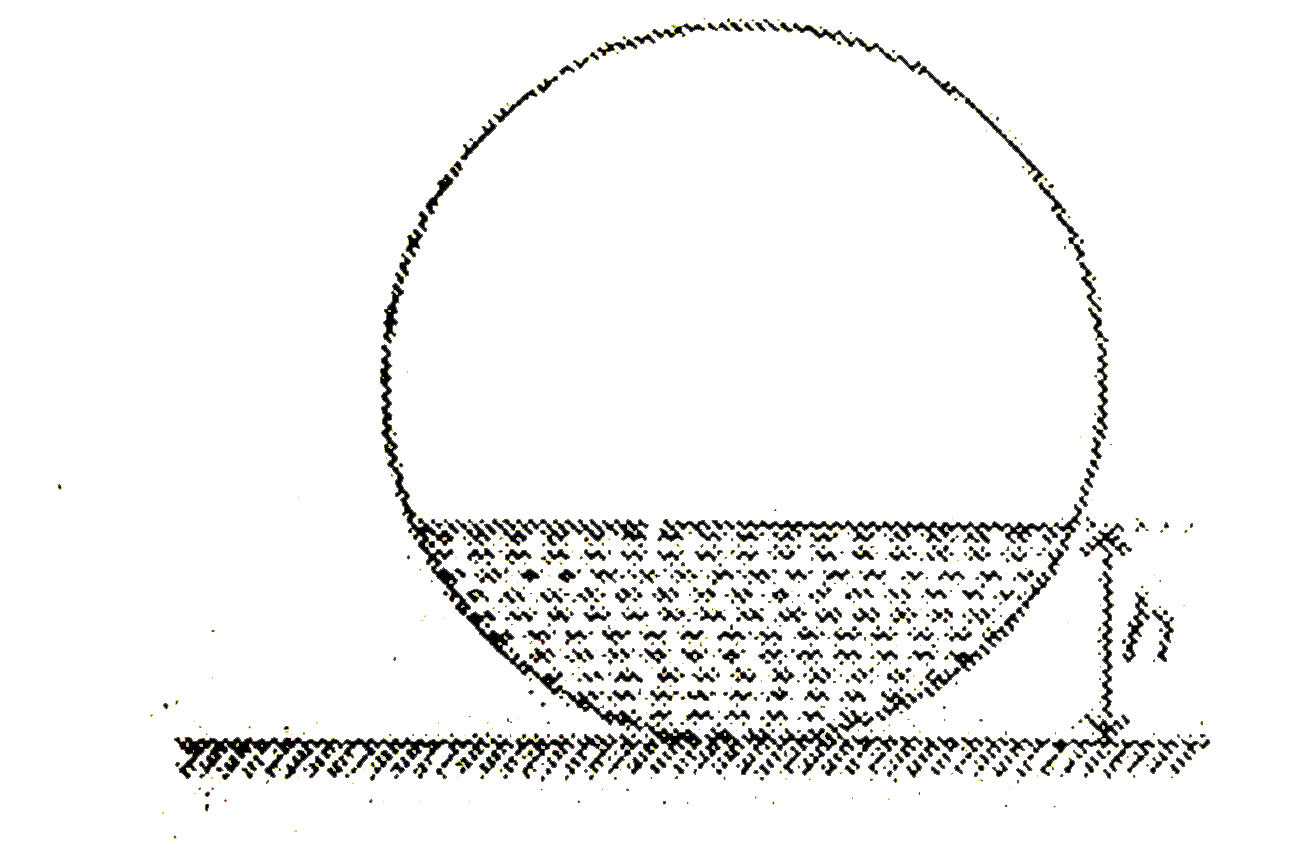 A0Bcos−1(R−hR)Ccos−1(hR)Dsin−1(R−hR)
A0Bcos−1(R−hR)Ccos−1(hR)Dsin−1(R−hR)
A long cylindrical vessel is half filled with a liquid. When the vessel is rotated about its own vertical axis, the liquid rises up near the wall. If the radius of vessel is 5 cm and its rotational speed is 2 rotations per second, then the difference in the heights between the centre and the sides, in cm, will be:
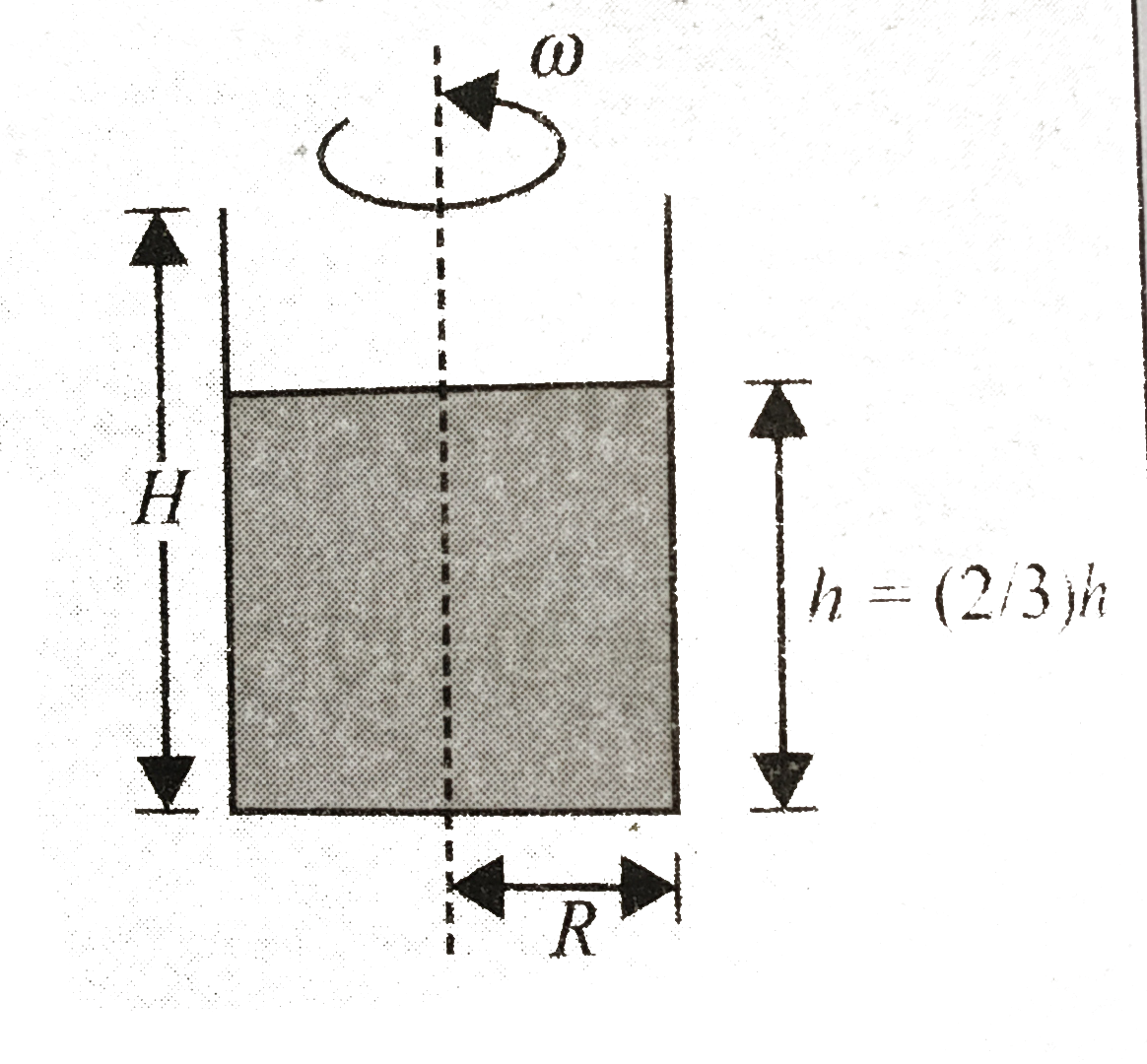
View SolutionA cylinder of radius R=1m and height H=3m two- thirds filled with water, is rotated about its vertical axis as shown in Fig. Determine the speed of rotation when
a. the: water just starts spilling over the rim.
b. the point at the centre of the base is just exposed.
View SolutionA cylindrical vessel is filled with a liquid up to a height H. A small hole is made in the vessel at a distance y below the liquid surface as shown in figure. The liquid emerging from the hole strike the ground at distance x
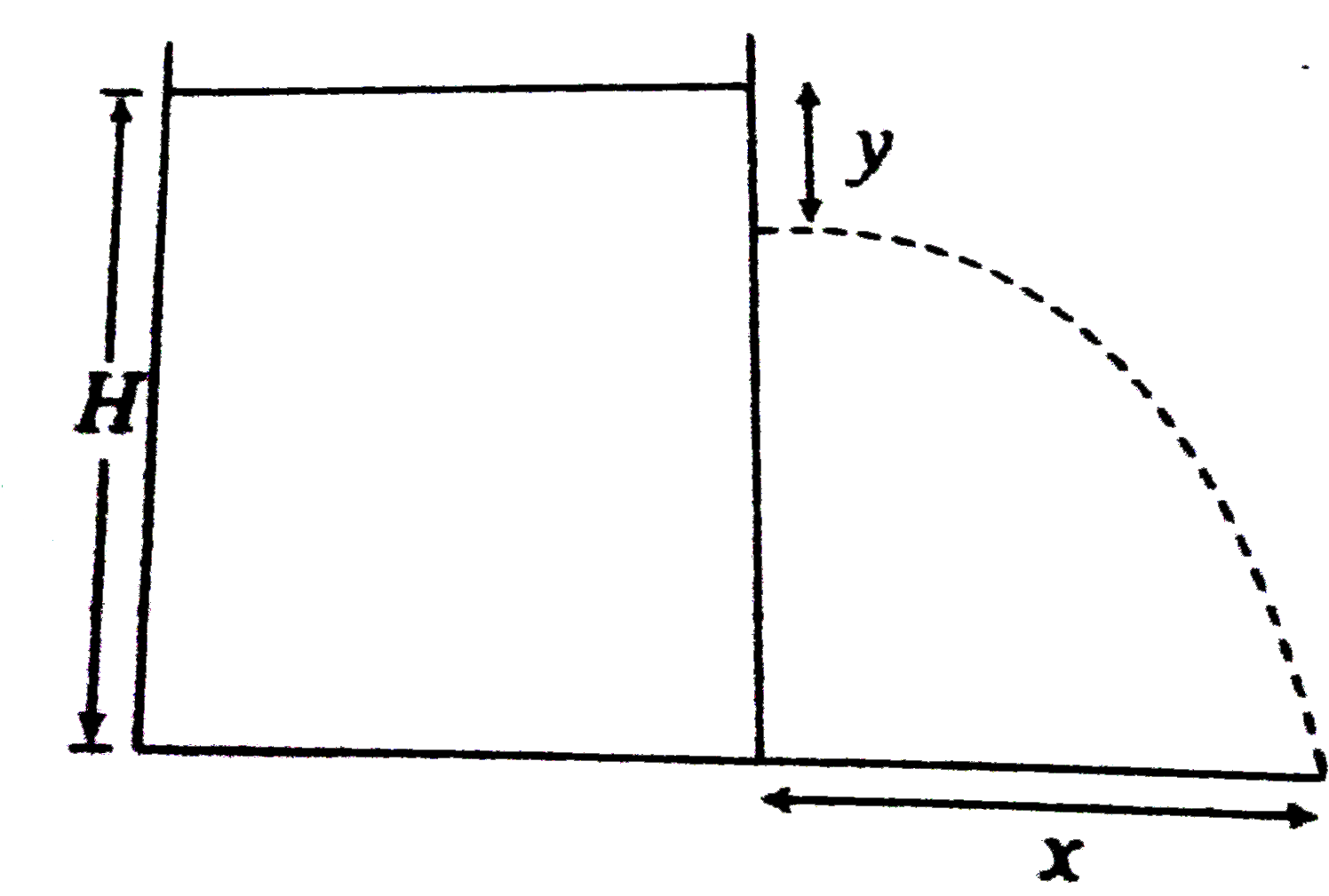
View SolutionA long cylinderical vessel is half filled with a liquid. When the vessel is rotated about its own vertical axis. The liquid rises up near the wall. If the radius of vessel is 5cm and its rotational speed is 2 rotatins per second, then the difference in the heights between the centre and the sides, in cm. will be :
View SolutionA long cylinderical vessel is half filled with a liquid. When the vessel is rotated about its own vertical axis. The liquid rises up near the wall. If the radius of vessel is 5cm and its rotational speed is 2 rotatins per second, then the difference in the heights between the centre and the sides, in cm. will be :
View Solution
