A doubly ionized lithium atom is hydrogen like with atomic number 3. Find the wavelength of the radiation required to excite the electron in Li++ from the first to the third Bohr orbit (ionization energy of the hydrogen atom equals 13.6 eV).
The correct Answer is:A, C, D
To find the wavelength of the radiation required to excite the electron in a doubly ionized lithium atom (Li²⁺) from the first to the third Bohr orbit, we can follow these steps:
Step 1: Identify the parameters
For a hydrogen-like atom, the energy levels can be calculated using the formula:
En=−Z2⋅13.6eVn2
where Z is the atomic number and n is the principal quantum number.
For Li²⁺, Z=3.
Step 2: Calculate the energy of the first and third orbits
- For the first orbit (n=1):
E1=−32⋅13.612=−9⋅13.61=−122.4eV
- For the third orbit (n=3):
E3=−32⋅13.632=−9⋅13.69=−13.6eV
Step 3: Calculate the change in energy
The change in energy (ΔE) when the electron transitions from the first to the third orbit is given by:
ΔE=E3−E1=−13.6eV−(−122.4eV)=−13.6+122.4=108.8eV
Step 4: Calculate the wavelength using the energy
The wavelength (λ) can be calculated using the formula:
λ=hcΔE
where h is Planck's constant (4.1357×10−15eV s) and c is the speed of light (3×108m/s).
In terms of electron volts and angstroms, we can use:
hc≈1240eV⋅nm
or
hc≈12375eV⋅Å
Thus,
λ=12375eV⋅Å108.8eV≈113.7Å
Final Answer
The wavelength of the radiation required to excite the electron in Li++ from the first to the third Bohr orbit is approximately 113.7 Å.
---
For a hydrogen-like atom, the energy levels can be calculated using the formula:
En=−Z2⋅13.6eVn2
where Z is the atomic number and n is the principal quantum number.
- For the first orbit (n=1):
E1=−32⋅13.612=−9⋅13.61=−122.4eV
E3=−32⋅13.632=−9⋅13.69=−13.6eV
The change in energy (ΔE) when the electron transitions from the first to the third orbit is given by:
ΔE=E3−E1=−13.6eV−(−122.4eV)=−13.6+122.4=108.8eV
The wavelength (λ) can be calculated using the formula:
λ=hcΔE
where h is Planck's constant (4.1357×10−15eV s) and c is the speed of light (3×108m/s).
hc≈1240eV⋅nm
or
hc≈12375eV⋅Å
λ=12375eV⋅Å108.8eV≈113.7Å
The wavelength of the radiation required to excite the electron in Li++ from the first to the third Bohr orbit is approximately 113.7 Å.
Topper's Solved these Questions
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 2 Single Correct
22 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 2 More Than One Correct
6 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 1 Objective
37 Videos
- MODERN PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICSEXERCISE - Integer Type Questions
17 Videos
- MODERN PHYSICS - 2BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Subjective
10 Videos
Similar Questions
A doubly ionized lithium atom is hydrogen like with atomic number 3. Find the wavelength of the radiation required to excite the electron in Li++ from to the third Bohr orbit (ionization energy of the hydrogen atom equals 13.6 eV).
View SolutionA doubly ionized lithium atom is hydrogen like with atomic
. number 3. Find the wavelength of the radiation to excite the electron in
. Li++ form the first to the third Bohr orbit. The ionization energy of the hydrogen
. Atom is 13.6V.
View Solution
Knowledge Check
the energy required to excite an electron in hydrogen atom to its first excited state is
A8.5eVB10.2eVC12.7eVD13.6eV
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is:
View SolutionThe ratio of the energy required to remove an electron from the first three Bohr's orbit of Hydrogen atom is
View SolutionExcitations energy of hydrogen atom is 13.6eV match the following
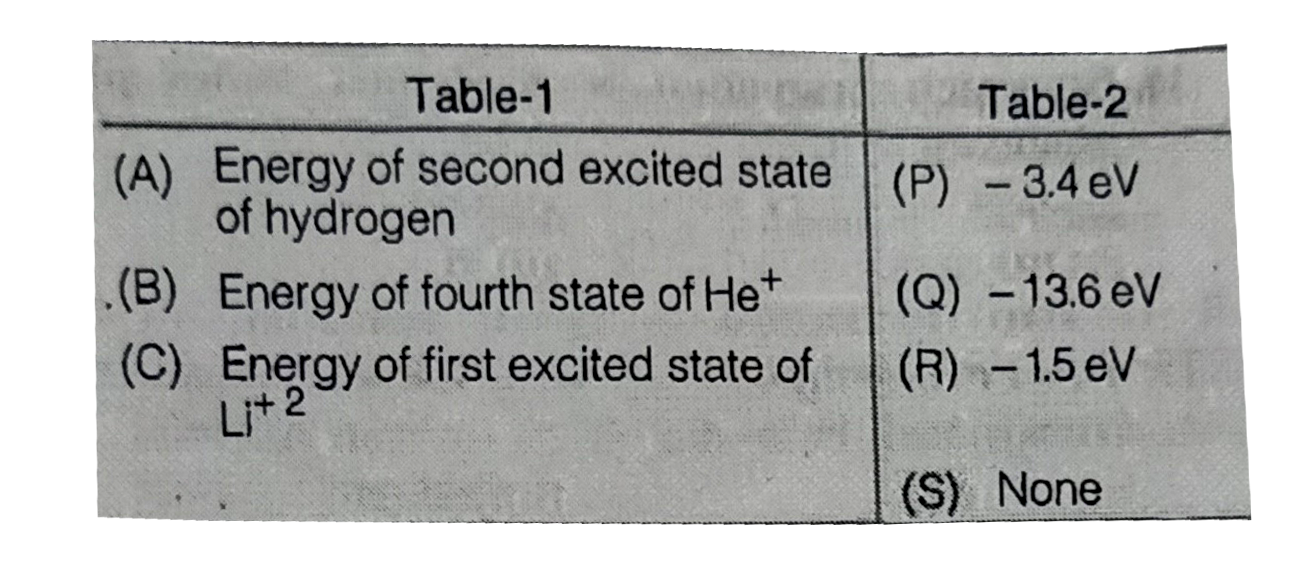
View SolutionThe ionization energy of a hydrogen like bohr atom is 4 Rydbergs (i) What is the wavelength of the radiation emitted when the electron jumps from the first excited state to the ground state ?(ii) what is the radius of the orbit for this atom ?
View SolutionFind the ratio of ionization energy of bohr 's hydrogen atom doubly liothium ion (Li2)
View SolutionIn hydrogen atom, if the difference in the energy of the electron in n=2 and n=3 orbits is E, the ionization energy of hydrogen atom is
View SolutionA single electron orbit around a stationary nucleus of charge +Ze where Z is a constant and e is the magnitude of the electronic charge. It requires47.2eV to excite the electron from the second bohr orbit to the third bohr orbit. Find
(i) The value of Z
(ii) The energy required by nucleus to excite the electron from the third to the fourth bohr orbit
(iii) The wavelength of the electronmagnetic radiation required to remove the electron from the first bohr orbit to inlinity
(iv) The energy potential energy potential energy and the angular momentum of the electron in the first bohr orbit
(v) The radius of the first bohr orbit (The ionization energy of hydrogen atom =13.6eV bohr radius =5.3×10−11matre velocity of light =3×108m/sec planks 's constant =6.6×10−34 jules - sec )
View Solution
