A farmer moves along the boundary of a square field of side $10 m$ in $40 s$ . What will be the magnitude of displacement of the farmer at the end of $2$ minutes $20$ seconds ?

Video Solution

Text Solution

Verified by Experts

First of all we will convert the total time of 2 minutes 20 seconds into seconds.
Total time = 2 minutes 20 seconds
$= 20 \times 60$ seconds + 20 seconds
= 120 seconds + 20 seconds
= 140 seconds
Now, In 40 s, number or rounds made = 1
So, In 140 s, number of rounds made = $\frac{1}{40} \times 140$
= 3.5

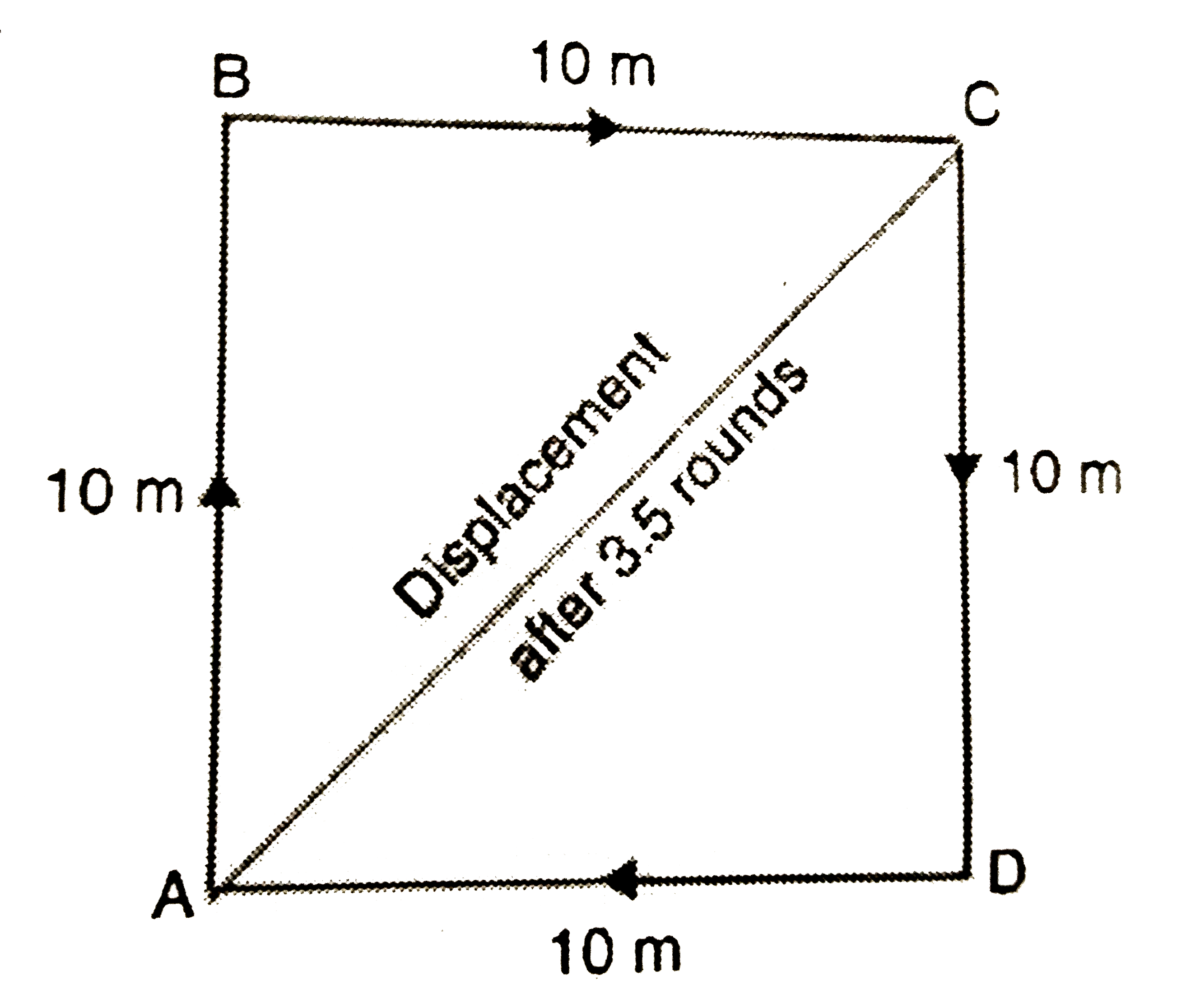
Thus, the farmer will make three and a half rounds (3.5 rounds) of the square field. If the farmer starts from position A (see Figure), then after three complete rounds, he will reach at starting position A. But in the next half round, the farmer will move from A to B, and B to C, so that his final position will be at C. Thus, the net displacement of the farmer will be AC. Now, ABC is a right angled triangle in which AC is the hypotenuse.
So,
${(A C)}^{2} = {(A B)}^{2} + {(B C)}^{2}$
${(A C)}^{2} = {(10)}^{2} + {(10)}^{2}$
${(A C)}^{2} = 100 + 100$
${(A C)}^{2} = 200$ ltBrgt $A C = \sqrt{200}$
AC = 14.143 m
Thus, the magnitude of displacement of the farmer at the end of 2 minutes and 20 seconds will be 14.143 metres.

Updated on:21/07/2023

A toy cyclist completes one round of a square track of side 2 min 40 seconds. What will be the displacement at the end of 3 minutes?
A52 m
Bzero
C16 m
D $2 \sqrt{2} m$
An athlete completes one round of a circular track of radius R in 20 second. What will be his displacement at the end of 2 minute 20 second?
AZero
B2R
C2nR
D7nR
A farmer moves along a square field of side 10 m in one hour. Write the following steps in sequential order to find his average speed.
(A) . Find th total distance (s) covered by the farmer.
(B) Note down the total time (t) required to cover the total distance.
(C) Use the formula , average speed = $\frac{total distance}{total time}$
(D) Substiute the values of s and t to determine the average speed.
AABCD
BACDB
CDABC
DCDAB