A fish is rising up vertically inside a pond with velocity of 4cm/s, and notices a bird , which is diving vertically downward and its velocity appears to be 16cm/s (to th fish). What is the real velocity of the diving bird, if refractive index of water is 4/3 ?
The correct Answer is:9cm/s
To solve the problem, we need to find the real velocity of the diving bird as observed by the fish, given the apparent velocity and the refractive index of water. Here’s a step-by-step solution:
Step 1: Understand the given information
- The fish is rising vertically with a velocity vf=4cm/s.
- The apparent velocity of the bird as seen by the fish is va=16cm/s.
- The refractive index of water is n=43.
Step 2: Relate the velocities using the refractive index
The relationship between the apparent velocity va, the real velocity of the bird vb, and the velocity of the fish vf can be expressed as:
va=vb+n⋅vf
Where:
- va is the apparent velocity of the bird,
- vb is the real velocity of the bird,
- n is the refractive index of water,
- vf is the velocity of the fish.
Step 3: Substitute the known values into the equation
Substituting the known values into the equation:
16=vb+(43)⋅4
Step 4: Calculate the term involving the refractive index
Calculate n⋅vf:
n⋅vf=43⋅4=163cm/s
Step 5: Substitute this value back into the equation
Now, substitute this back into the equation:
16=vb+163
Step 6: Solve for the real velocity of the bird vb
To isolate vb, we rearrange the equation:
vb=16−163
Convert 16 into a fraction with a denominator of 3:
16=483
Now substitute:
vb=483−163=323cm/s
Step 7: Convert to decimal form (if necessary)
Calculating 323:
vb≈10.67cm/s
Final Answer
The real velocity of the diving bird is approximately 10.67cm/s.
---
- The fish is rising vertically with a velocity vf=4cm/s.
- The apparent velocity of the bird as seen by the fish is va=16cm/s.
- The refractive index of water is n=43.
The relationship between the apparent velocity va, the real velocity of the bird vb, and the velocity of the fish vf can be expressed as:
va=vb+n⋅vf
Where:
- va is the apparent velocity of the bird,
- vb is the real velocity of the bird,
- n is the refractive index of water,
- vf is the velocity of the fish.
Substituting the known values into the equation:
16=vb+(43)⋅4
Calculate n⋅vf:
n⋅vf=43⋅4=163cm/s
Now, substitute this back into the equation:
16=vb+163
To isolate vb, we rearrange the equation:
vb=16−163
Convert 16 into a fraction with a denominator of 3:
16=483
Now substitute:
vb=483−163=323cm/s
Calculating 323:
vb≈10.67cm/s
The real velocity of the diving bird is approximately 10.67cm/s.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(single correct choice type)49videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(More than one correct choice type)14videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Check point6videos
GEOMETRICAL OPTICS : REFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
GEOMETRICAL OPTICS : REFLECTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GRAVITATIONEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)4videos
GRAVITATION
Similar Questions
A fish is rising up vertically inside a pond with velocity 4 cm/s and notices a bird, which is diving vertically downward and its velocity apperars to be 16cm/s (to the fish) . What is the real velocity of the diving bird, If refractive index of water is 4/3
View SolutionA fish is moving up vertically with speed 4 cm/s inside a pond. A bird is moving vertically downward (see figure). Velocity of the bird w.r.t. fish is 16 cm/s downward. Find out the actual velocity of the bird (in cm/s). Given refractive index of water is 43.
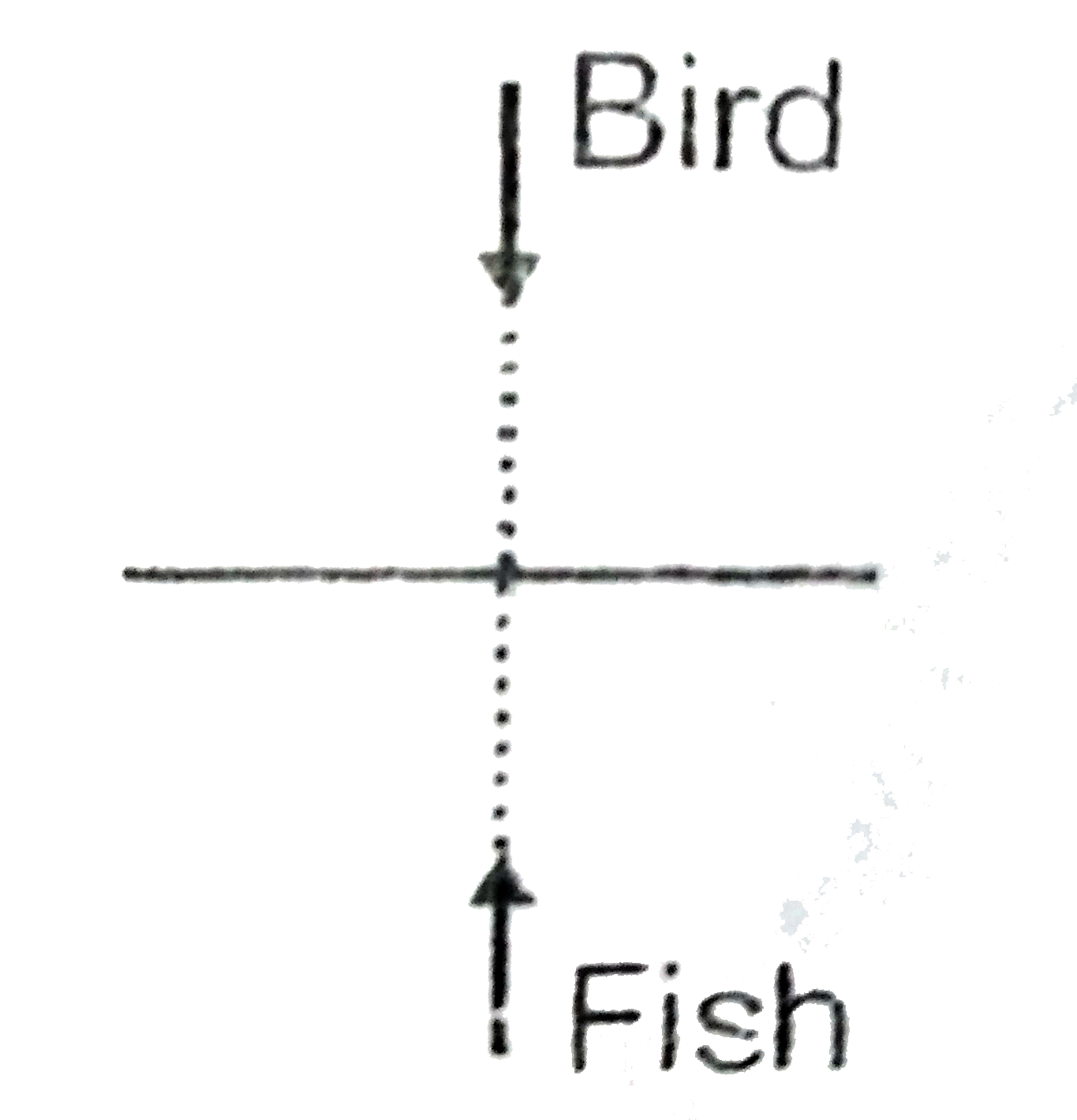 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In a lake, a fish rising vertically to the surface of water uniformly at the rate of 3 m/s, observes a bird diving vertically towards the water at the rate of 9 m/s. The actual velocity of the dive of the bird is (given, refractive index of water = 4/3)
A3.6 m/sB4.5 m/sC6.0 m/sD12.0 m/s - Question 2 - Select One
A fish rising up vertically toward the surface of water with speed 3ms−1 observes a bird diving down vertically towards it with speed 9ms−1 . The actual velocity of bird is
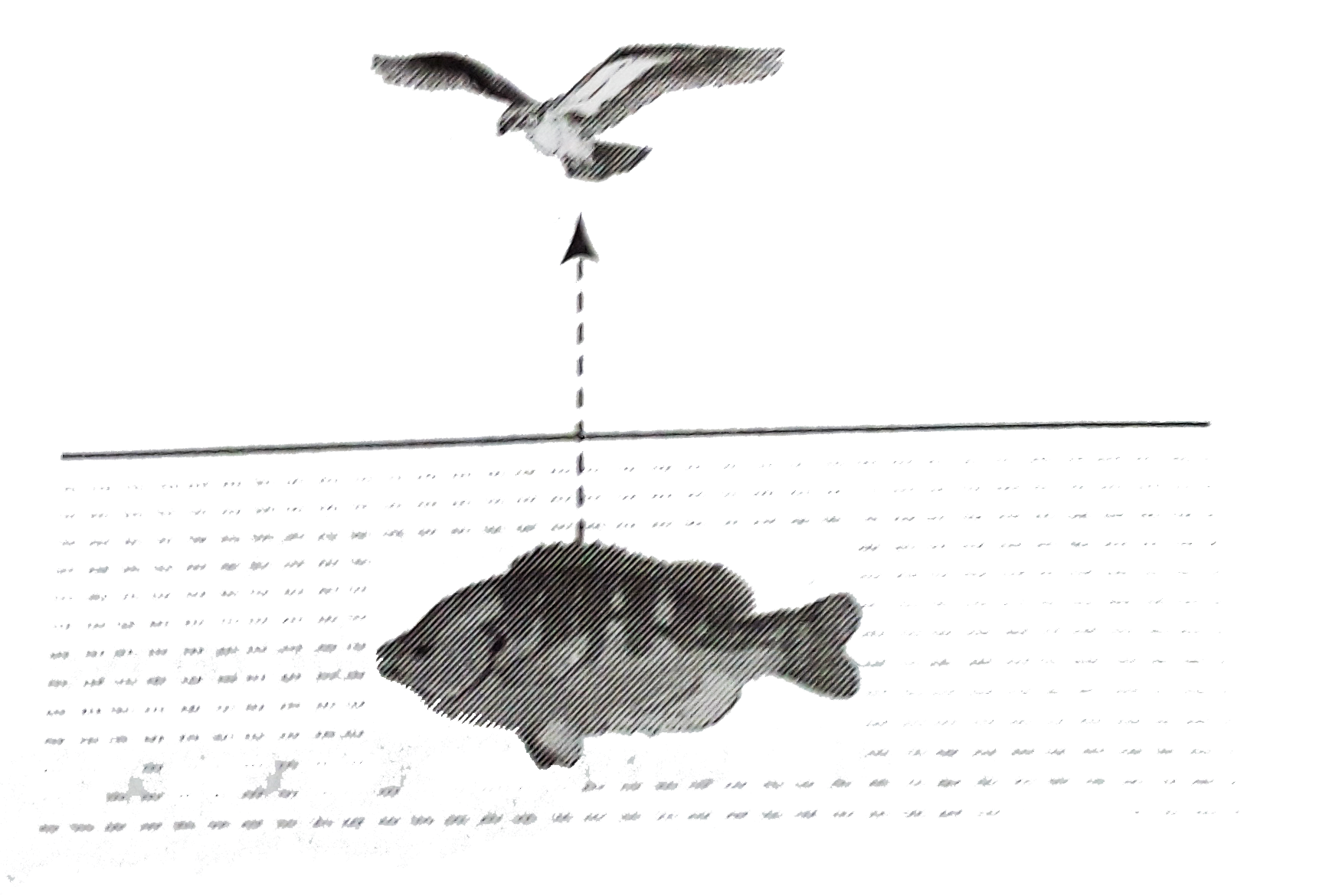 A4.5ms−1B5.4ms−1C3.0ms−1D3.4ms−1
A4.5ms−1B5.4ms−1C3.0ms−1D3.4ms−1 - Question 3 - Select One
Rain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
A5m/s at an angle tan−1(4/3) with horizontalB5m/s at an angle tan−1(3/4) with verticalC5m/s at an angle tan−1(4/3) with verticalDBoth (1) and (2)
A fish rising vertically up towards the surface of waterwith speed 3ms^(-1) observes a bird diving vertically down towards it with speed 9ms^(-1) The actual velocity of bird is (Given mu_ water =4/3
View SolutionA point object O and a mirror M move with velocities of 3cms−1 and 4cms−1 , respectively as shown in the figure. OO' is the normal to the mirror. What is the velocity of the image?
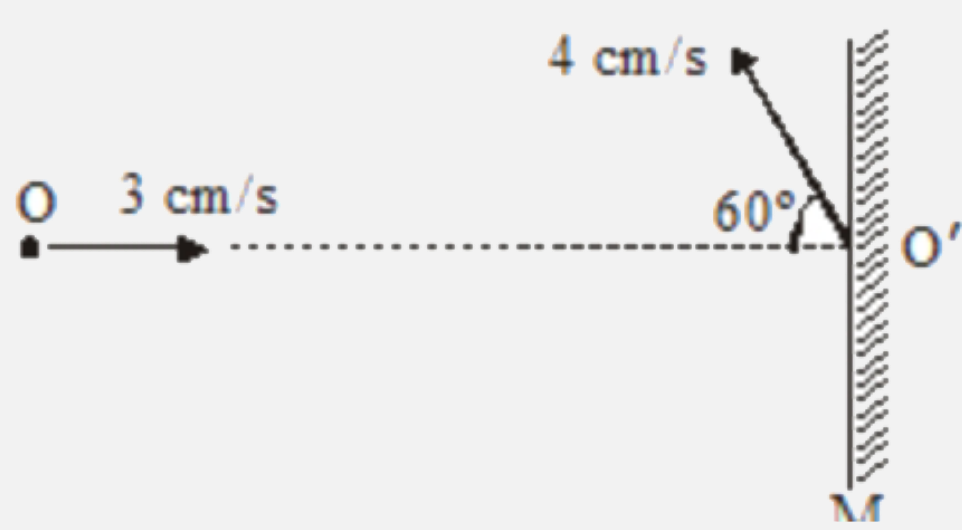 View Solution
View SolutionWhat is the real depth of a swimming pool when its bottom appears to be raised by 1 m ? Given refractive index of water is 4/3.
View SolutionA bird is flying 3m above the surface of water,If the bird is diving vertically down with speed =6m/shis apparent velocity as seen by a stationary fish underwater is:
View SolutionIn the previous question, if the bird is diving vertically down with speed =6m/s , his appearent speed as seen by a stationary fish underwater is
View Solution

