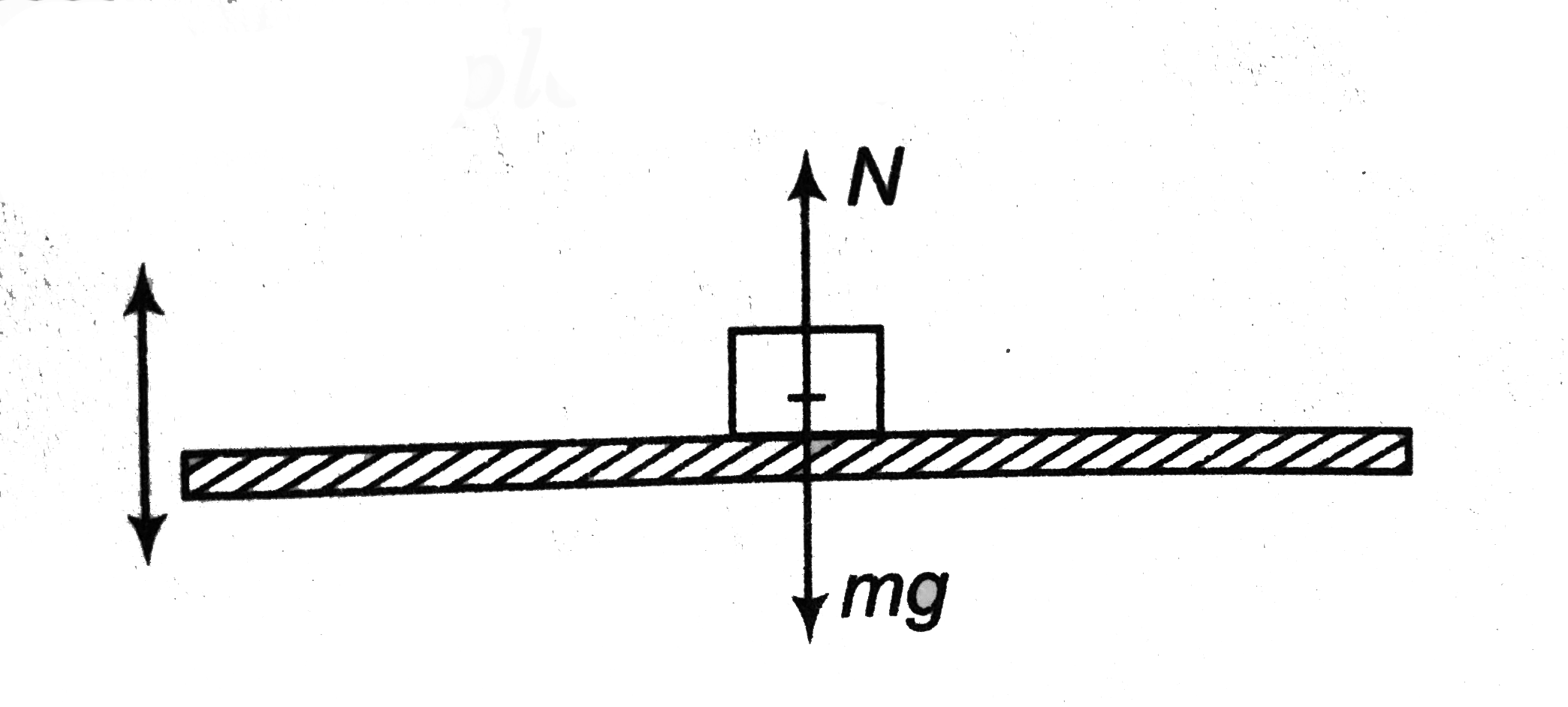
A horizontal plank has a rectangular block placed on it. The plank starts oscillating vertically and simple harmonically with an amplitude of 40 cm. The block just loses contact with the plank when the latter is at momentary rest Then.
the period of oscillation is (2π5)
the block weight double its weight, when the plank is at one of the positions of momentary rest.
the block weighs 0.1 times its weight on the plank halfway up.
the block weighs 1.5 times its weight on the plank halfway down.
The correct Answer is:A, B, C, D
Given A=4 and a=g
ω2A=g⇒ω2=104=25
ω=5,T=2πω=2π5sec.
At lowest position acc. =ωA+g=g+g=2g
So weight =m(2g)=2mg
At half distance a=g2
So weight =m(g−g2)=mg2
At upper half distance and weight at lower half distance
=m(g+g2)=3mg2 actual weight of a equilibrium position (maximum v)
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning
6 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Comprehension
33 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICSCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct
107 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
A block is resting on a piston which is moving vertically with simple harmonic motion of period 1.0 second. At what amplitude of motion will the block and piston separate? What is the maximum velocity of the piston at this amplitude?
View SolutionA block is resting on a piston which is moving vertically with simple harmonic motion of period 1.0 second. At what amplitude of motion will the block and pisto separate? What is the maximum velocity of the piston at this amplitude?
View Solution
Knowledge Check
A clock is performing SHM along a vertical line with amplitude of 40 cm on a horizontal plank. The block just lose the contact with plank when plank is momentarily at rest. Then, (Take, g=10m/s2)
Athe period of its oscillation is 2π5sBthe period of is oscillation is 2π6sCthe period of its oscillation is π5sDNone of the aboveA horizontal platform with a small block of mass 1 kg kept on it performs vertical SHM of amplitude 1 cm. The block does not lose contact with the platform anywhere. The minimum possible time period of the platform
A0.1 sB0.2 sC0.3 sD0.4 sA book is resting on shelf that is undergoing vertical simple harmonic oscillations with an amplitude of 2.5 cm. What is the minimum frequency of oscillation of the shell for which the book will lose contact with the shelf ? (Assume that g=10m/s2)
A20 HzB3.18 HzC125.6 HzD10 Hz
A plank is being pushed on a frictionless horizontal floor with a constant velocity u=1.5ms .When a block is gently placed on the plank,it slides for a while,acquires velocity of the plank and then moves together with the plank.If the agency pushing the plank has to do a work W = 11.25 J during the sliding of the block,find mass of the block in kg.
View SolutionA block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
View SolutionA plank with a small block on top of it is under going vertical SHM. Its period is 2sec. The minium amplitude at which the block will separate from plank is :
View Solution
A plank of mass 12 kg is supported by two identical springs as shown is Fig. The plank always remains horizontal. When the plank is pressed down and released it performs simple harmonic motion with time period 3 s. When a block of m is attached to the plank the time priod changes to 6 s. The mass of the block is
View SolutionA block of mass 2 kg rests on a rough horizontal plank , the coefficient of friction between the plank and block is 0.3. If the plank is pulled horizontally with a constant acceleration of 4m/s2 , the distance moved by the block on the plank in 5 second starting from rest (in m) is : (Take g = 10m/s2)
View Solution
