A lift of mass 'm' is connected to a rope which is moving upward with maximum acceleration 'a'. For maximum safe stress, the elastic limit of the rope is 'T'. The minimum diameter of the rope is
(g = gravitational acceleration)
[2m(g+a)πT]1/2
[4m(g+a)πT]1/2
[m(g+a)πT]1/2
[m(g+a)2πT]1/2
The correct Answer is:B
When the lift is moving upwards, the maximum tension in the rope = m (g + a)
Stress in the rope =m(g+a)πr2
T=m(g+a)πr2=m(g+a)π(d2)2
∴T=4m(g+a)πd2∴d2=4m(g+a)πT
∴d=[4m(g+a)πT]1/2 .... Option (b)
Topper's Solved these Questions
- ELASTICITYBOOK - MARVEL PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Test Your Grasp - 5
15 Videos
- CURRENT ELECTRICITYBOOK - MARVEL PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - MCQ
151 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS EXERCISE - TEST YOUR GRASP - 16
30 Videos
Similar Questions
A lift of mass 2000 kg is supported by thick steel ropes . If maximum upward acceleration of the lift be 1.2m/s2 , and the breaking stress for the ropes be
2.8×108Nm−2
what should be the minimum diameter of rope ?
View SolutionThe maximum tension a rope can withstand is 60 kg - wt. The ratio of maximum acceleration with which two boys of masses 20kg and 30kg can climb up the rope at the same time is
View Solution
Knowledge Check
An object of mass 5 kg is attached to the end ofa rope. If the rope is pulled upward with an acceleration 0.30ms−2, what is the tension in the rope?
A30.5 NB40.5 NC50.5 ND60.5 NA monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
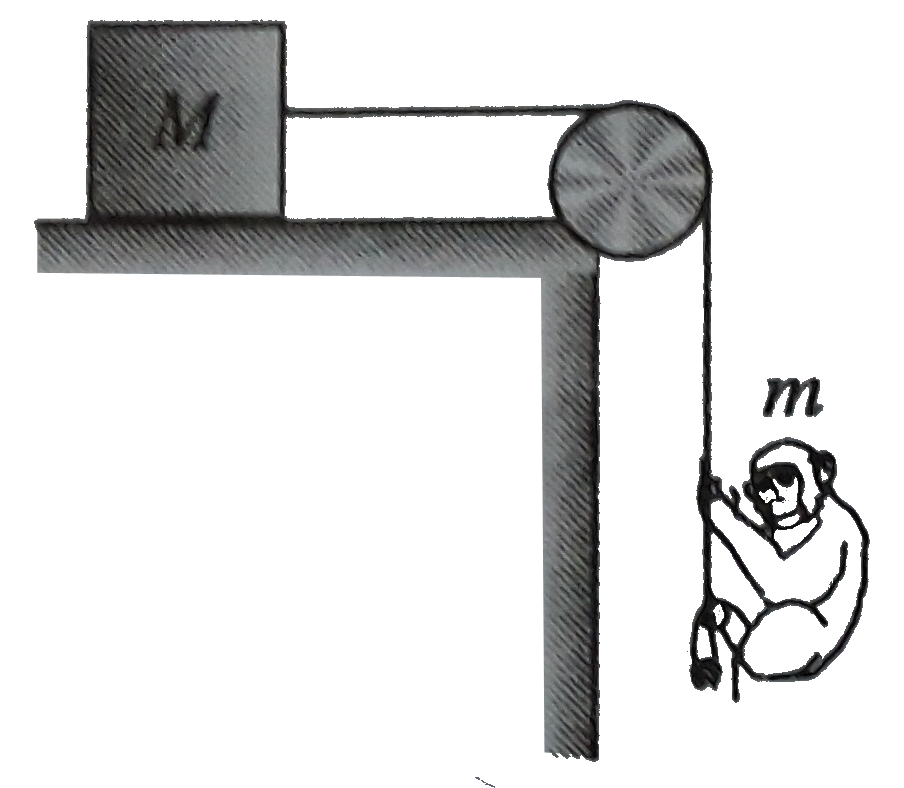
The acceleration of weight isA2m(g+b)−μMgM+2mBm(g+b)−μMg2(M+m)Cm(g+b)−3μMgM+3mDm(g+b)−μMgM+2mA bucket of mass 'm' tied to a light rope is lowered at a constant acceleration of g/4. IF the bucket is lowered by a distance 'd' , the work done by the rope will be (neglect the mass of the rope)
A14mgdB34mgdC−34mgdD−54mgd
A block of 'm' is lowered with the help of a rope of negligible mass through a distance 'd' with an acceleration of g/3. Find the work done by the rope on the block ?
View SolutionThe maximum tension a rope can withstand is 60kg−wt The ratio of maximum accelertion with which two boys of masses 20kg and 3kg can climb up the rope at the same time is .
View SolutionA 40 kg boy climbs a rope that passes over an ideal pulley. The other end of the rope is attached to a 60 kg weight placed on the ground. What is the maximum upward acceleration the boy can have without lifting the weight ? If he climbs the rope with upward acceleration 2 g, with what acceleration the weight will rise up ?
View SolutionBlock A of mass m/2 is connected to one end of light rope which passes over a pulley as shown in fig. A man of mass m climbs the other end of rope with a relative acceleration of g/6 with respect to rope. Find the acceleration of block A and tension in the rope.

View SolutionA monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
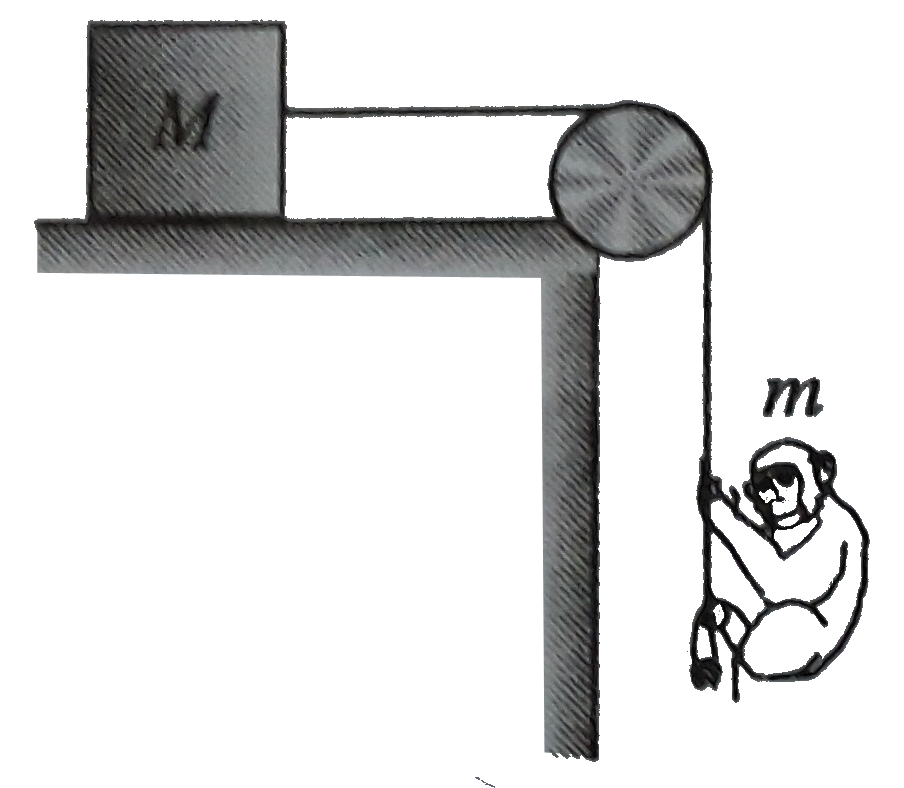
The tension of rope is
View Solution
