A light ray is incident along the principal axis of a mirror after reflection ______.
retraces its path
passes through center of curvature
makes zero angle with the normal.
All the above.
The correct Answer is:D
The light ray which is incident along the principal axis of a mirror falls normally on the mirror, and hence, it retraces its path.
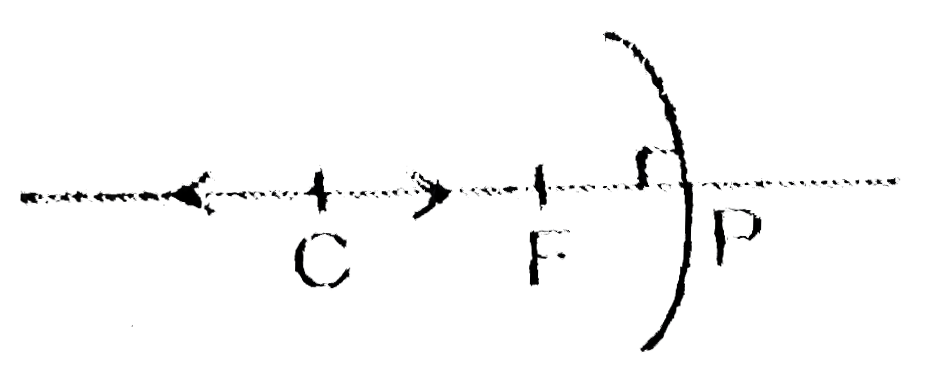
From the figure it is clear that is passes through the centre of curvature.
As the line passing through the centre of curvature is perpendicular to the mirror, the angle made by the light ray with the normal is zero.
Topper's Solved these Questions
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Level 2
23 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - Level 3
5 Videos
- LIGHTBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LIGHTEXERCISE - LONG ANSWER TYPE QUESTIONS
7 Videos
- KINEMATICSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - KINEMATICSEXERCISE - ASSESSMENT TEST (TEST 2 (SELECT THE CORRECT ALTERNATIVE)
15 Videos
- MACHINES AND TOOLSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - MACHINES AND TOOLSEXERCISE - CONCEPT APPLICATION (LEVEL 2)
12 Videos
Similar Questions
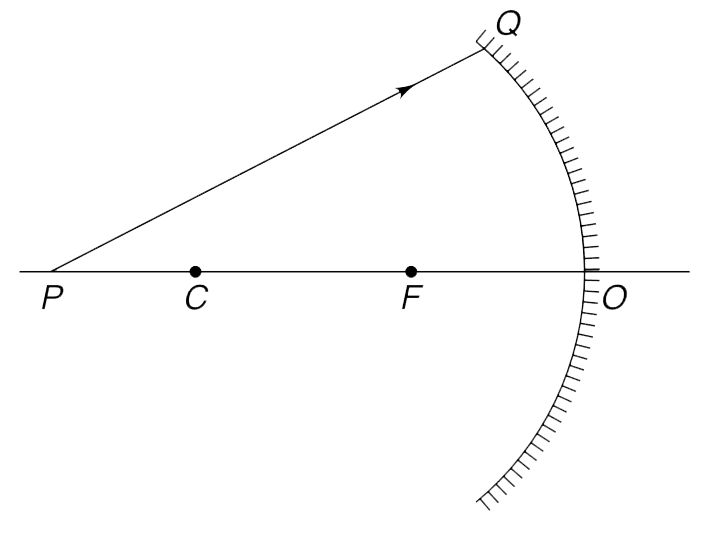
We know that parallel light rays which are inclined to the principal axis of a spherical mirror, after reflection converge at a point in the focal plane of the mirror. With this knowledge explain how you will trace the reflected ray for incident ray PQ shown in the Fig. F is focus and C is centre of curvature of the mirror.
View SolutionWhen a light ray is incident normally on the surface of a plane mirror, the reflected ray deviates through an angle of ________
View Solution
Knowledge Check
A light ray is incident along the principal axis of a mirror after reflection ______.
Aretraces its pathBpasses through centre of curvatureCmakes zero angle with the normalDAll the aboveA light ray is incident along the principal axis of a mirror after reflection ______.
Aretraces its pathBpasses through centre of curvatureCmakes zero angle with the normalDAll the aboveAfter reflecton, a light ray which is incident along the principal axis of a mirror,
Aretraces its pathBpasses through center of curvatureCmakes zero angle with the normal.DAll the above.
Radius of curvature of concave mirror is R and a light ray is incident on it parallel to principal axis at a height h above the principal axis. Calculate distance of the point from pole of mirror where reflected ray intersects with principal axis.
View SolutionMark the statement True (T) or False(F).A ray of light incident parallel to the principal axis of a spherical mirror retraces its path after reflection.
View SolutionA light ray is incident normally on a plane mirror. The angle of reflection will be
View SolutionA light ray incidents at an angle 'i' on a plane mirror and undergoes reflection. Arrange the following steps in sequential order to calculate the angle of deviation of the light ray.
(A) The angle between the incident ray and the extended light ray is 180∘ (B) In case of reflection, the angle of incidence (i) is equal to the angle of reflection (r). Then, the angle between the incident ray and the reflected ray is 2i. (C) Draw an imaginary line, extending the incident light ray, which represents the actual path of the light ray. (D) The angle of deviation is the angle between the reflected light ray and the actual path of the light ray, i.e., 180∘−2i
View SolutionA light ray incidents at an angle 'l' on a plane mirror and undergoes reflection. Arrange the following steps in sequential order to calculate the angle of deviatino of the light ray.
The angle between the incident ray and the extended light ray is 180∘.
In case of reflection, the angle of incidence (i) is equal to the angle of reflection (r). Then the angle between the incident ray and the reflected ray is 2i.
Draw an imaginary line, extending the incident light ray, which reprsesnts the actual path of the light ray.
The angle of deviation is the angle between the reflected light ray and the actual path of the light ray, i.e., 180∘−2i.
View Solution
