A light rays is incident on a prism of prism angle A and refractive index $μ$ . Show that the smallest value of the angle of incidence for which the reflected ray does not suffer total internal reflection at the second face, i.e. just comes out of it, is given by
$i = {sin}^{- 1} [{(μ^{2} - 1)}^{1 / 2} sin A - cos A]$ .

Video Solution

Text Solution

Verified by Experts

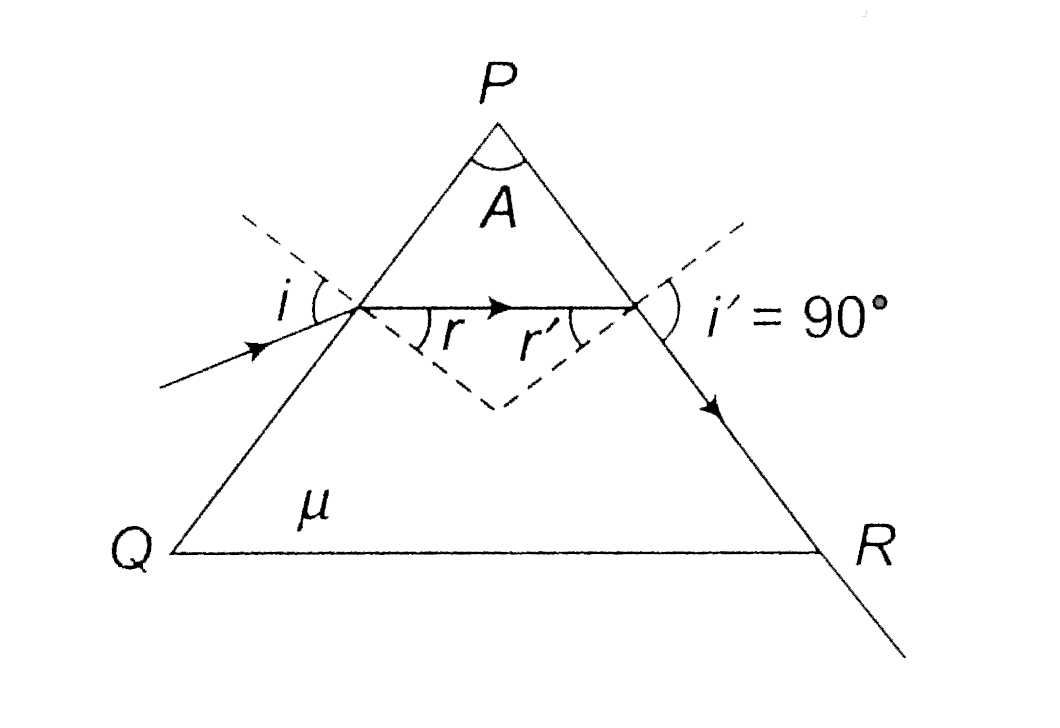
$i^{'} = 90^{\circ}$ i.e. $r^{'} = C$ , $sin C = 1 / μ$
$r + r^{'} = A$
$r + C = A \Rightarrow r = A - C$
Refraction on PQ:
$1 \times sin i = μ sin r = μ sin (A - C)$
$= μ (sin A cos C - cos A sin C)$
$= μ [sin A \sqrt{1 - {sin}^{2} C} - cos A sin C]$
$= μ [sin A \sqrt{1 - \frac{1}{μ^{2}}} - cos A \cdot \frac{1}{μ}]$
$= μ [\frac{sin A \sqrt{μ^{2} - 1} - cos A}{μ}]$
$= sin A \sqrt{μ^{2} - 1} - cos A$
$i = {sin}^{- 1} [sin A \sqrt{μ^{2} - 1} - cos A]$

|

Updated on:21/07/2023

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Light ray is incident on a prism of angle $A = 60^{\circ}$ and refractive index $μ = \sqrt{2}$ .the angle of incidence at which the emergent ray grazes the surface is given by
A ${sin}^{- 1} (\frac{\sqrt{3} - 1}{2})$
B ${sin}^{- 1} (\frac{1 - \sqrt{3}}{2})$
C ${sin}^{- 1} (\frac{\sqrt{3}}{2})$
D ${sin}^{- 1} (\frac{2}{\sqrt{3}})$
Question 2 - Select One
Light ray is incident on a prism of angle $A = 60^{\circ}$ are refractive index $μ = \sqrt{2}$ . The angle of incidence which the emergent rays grazes the surface is given
A ${sin}^{- 1} (\frac{\sqrt{3} - 1}{2})$
B ${sin}^{- 1} (\frac{\sqrt{2} - 1}{2})$
C ${sin}^{- 1} (\frac{\sqrt{3}}{2})$
D ${sin}^{- 1} (\frac{2}{3 \sqrt{3}})$
Question 3 - Select One
The angle of incidence at which reflected light is totally polarized for reflection form air to glass ( refraction index $μ$ ) is
A ${sin}^{- 1} (μ)$
B ${sin}^{- 1} (\frac{1}{μ})$
C ${tan}^{- 1} (\frac{1}{μ})$
D ${tan}^{- 1} (μ)$

CP SINGH-REFRACTION OF LIGHT BY PLANE SURFACES-Exercises

Home
Ask Doubt
Profile