A light spring of force constant k is attached to a block of mass M placed on a horizontal firctionless surface from its one end and other end is fixed to a right support. The system is executing S.H.M. of amplitude A1 and time period T1. At some instant, the block passed through the equilibrium position and a small object of mass m is placed on it. The new amplitude and time period of the system now becomes A2 and T2 respectively.
The ratio A2/A1 is
(M+mM)1/2
(MM+m)1/2
(M−mM1/2)
(M+m(M−m)1/2)
The correct Answer is:B
To solve the problem, we need to find the ratio of the new amplitude A2 to the original amplitude A1 after a small mass m is placed on a block of mass M that is already executing simple harmonic motion (SHM) with amplitude A1 and time period T1.
1. Understanding the Initial Conditions:
- The system consists of a block of mass M attached to a spring with force constant k.
- The block is executing SHM with amplitude A1 and time period T1.
2. At the Equilibrium Position:
- When the block passes through the equilibrium position, it has a maximum velocity V1.
- The kinetic energy at this position is given by:
KE1=12MV21
- The potential energy at the extreme position (maximum amplitude) is:
PE1=12kA21
- At maximum displacement, all kinetic energy converts to potential energy:
12MV21=12kA21
3. Introducing the Small Mass m:
- When the small mass m is placed on the block of mass M at the equilibrium position, the total mass of the system becomes M+m.
- The new velocity V2 of the combined mass system can be found using the conservation of momentum:
MV1=(M+m)V2
- Rearranging gives:
V2=MM+mV1
4. Calculating the New Kinetic Energy:
- The new kinetic energy when both masses are moving together at the equilibrium position is:
KE2=12(M+m)V22
- Substituting V2:
KE2=12(M+m)(MM+mV1)2=12M2M+mV21
5. Relating Kinetic Energy to Potential Energy for the New System:
- The potential energy at the new maximum amplitude A2 is:
PE2=12kA22
- Setting the kinetic energy equal to the potential energy at maximum displacement:
12M2M+mV21=12kA22
6. Finding the Ratio of Amplitudes:
- From the original condition, we have:
12MV21=12kA21⟹V21=kA21M
- Substituting this into the equation for PE2:
M2M+m⋅kA21M=kA22
- Simplifying gives:
MM+mkA21=kA22⟹A22=MM+mA21
- Taking the square root:
A2A1=√MM+m
Final Result:
The ratio of the new amplitude to the original amplitude is:
A2A1=√MM+m
- The system consists of a block of mass M attached to a spring with force constant k.
- The block is executing SHM with amplitude A1 and time period T1.
- When the block passes through the equilibrium position, it has a maximum velocity V1.
- The kinetic energy at this position is given by:
KE1=12MV21
- The potential energy at the extreme position (maximum amplitude) is:
PE1=12kA21
- At maximum displacement, all kinetic energy converts to potential energy:
12MV21=12kA21
- When the small mass m is placed on the block of mass M at the equilibrium position, the total mass of the system becomes M+m.
- The new velocity V2 of the combined mass system can be found using the conservation of momentum:
MV1=(M+m)V2
- Rearranging gives:
V2=MM+mV1
- The new kinetic energy when both masses are moving together at the equilibrium position is:
KE2=12(M+m)V22
- Substituting V2:
KE2=12(M+m)(MM+mV1)2=12M2M+mV21
- The potential energy at the new maximum amplitude A2 is:
PE2=12kA22
- Setting the kinetic energy equal to the potential energy at maximum displacement:
12M2M+mV21=12kA22
- From the original condition, we have:
12MV21=12kA21⟹V21=kA21M
- Substituting this into the equation for PE2:
M2M+m⋅kA21M=kA22
- Simplifying gives:
MM+mkA21=kA22⟹A22=MM+mA21
- Taking the square root:
A2A1=√MM+m
The ratio of the new amplitude to the original amplitude is:
A2A1=√MM+m
Topper's Solved these Questions
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Matching Type Questions)
2 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (MATRIX MATCH TYPE QUESTIONS)
2 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Multiple choice question)
11 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST
16 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
Similar Questions
A block of mass M executes SHM with amplitude a and time period T. When it passes through the mean position, a lump of putty of mass m is dropped on it. Find the new amplitude and time period.
View SolutionA horizontal spring block system of mass M executes simple har monic motion.w hen the block is passingle through its equilibrium position, an object of mass m is put on it and the two move tother. Find the new amplitude and frequency of vibration.
View Solution
Knowledge Check
A light spring of force constant k is attached to a block of mass M placed on a horizontal firctionless surface from its one end and other end is fixed to a right support. The system is executing S.H.M. of amplitude A1 and time period T1. At some instant, the block passed through the equilibrium position and a small object of mass m is placed on it. The new amplitude and time period of the system now becomes A2 and T2 respectively.
The velcities of block before and after the object is placed on it are V1 and V2 respectively. Then the ratio V2/V1A(M+mM)B(MM+m)C(M−mM01/2D(MM+m)(A2A1A horizontal spring-block system of mass 1kg executes SHM of amplitude 10 cm. When the block is passing through its equilibrium position another mass of 1kg is put on it and the two move together.
Aamplitude will remain unchangedBamplitude will become 5√2 cmCthe frequency of oscillations will remain sameDthe frequency of oscillations will decrease.A mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
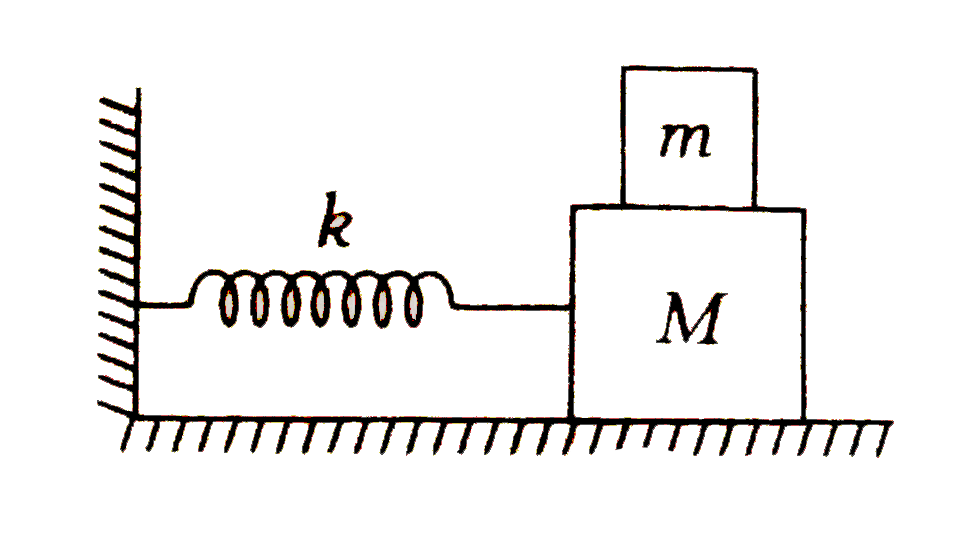 A√(M+m)MAB√(M−m)MAC√M(M+m)AD√M(M−m)A
A√(M+m)MAB√(M−m)MAC√M(M+m)AD√M(M−m)A
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View SolutionA block of mass M is lying on a frictionless horizontal surface and it connected to a spring of spring constant k, whose other end is fixed to a wall, system executes simple harmonic motion of amplitude A0, when the block is passing through its equilibrium positions, an object of mass m is put on it and the two move together with a new time period T and amplitude A. Find the value of T and A in terms of given constants.
View SolutionA block of mass M attached to the free end of a spring of force constant k is nounted on a smooth horizontal table as shown in figure.
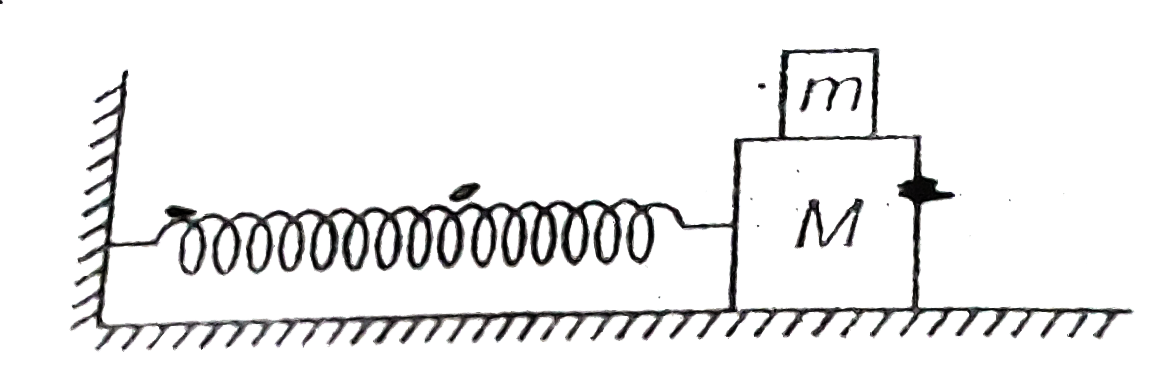
The block executes SHM with amplitude A and frequency f . If an object of mass m is put on it, when the block is passing through its equilibium position and the two move together ,then what is the new amplitude and frequency of vibration?
View SolutionA sping of spring constant k is attached to the ceiling. A block of mass M is attached to its lower end and is released suddenly.
(ii) Find its speed at the instant it passes throught the equilibrium position.
View SolutionA horizontal spring -block system of mass 2kg executes S.H.M when the block is passing through its equilibrium positiojn an object of mass 1kg is put on it the two move togather The new amplitude of vibration is (A being its initial amplitude)
View Solution
