A long copper wire carries a current of I ampere. Calculate the magnetic flux per meter of the wire for a plane surface S inside the wire as shown in Fig.
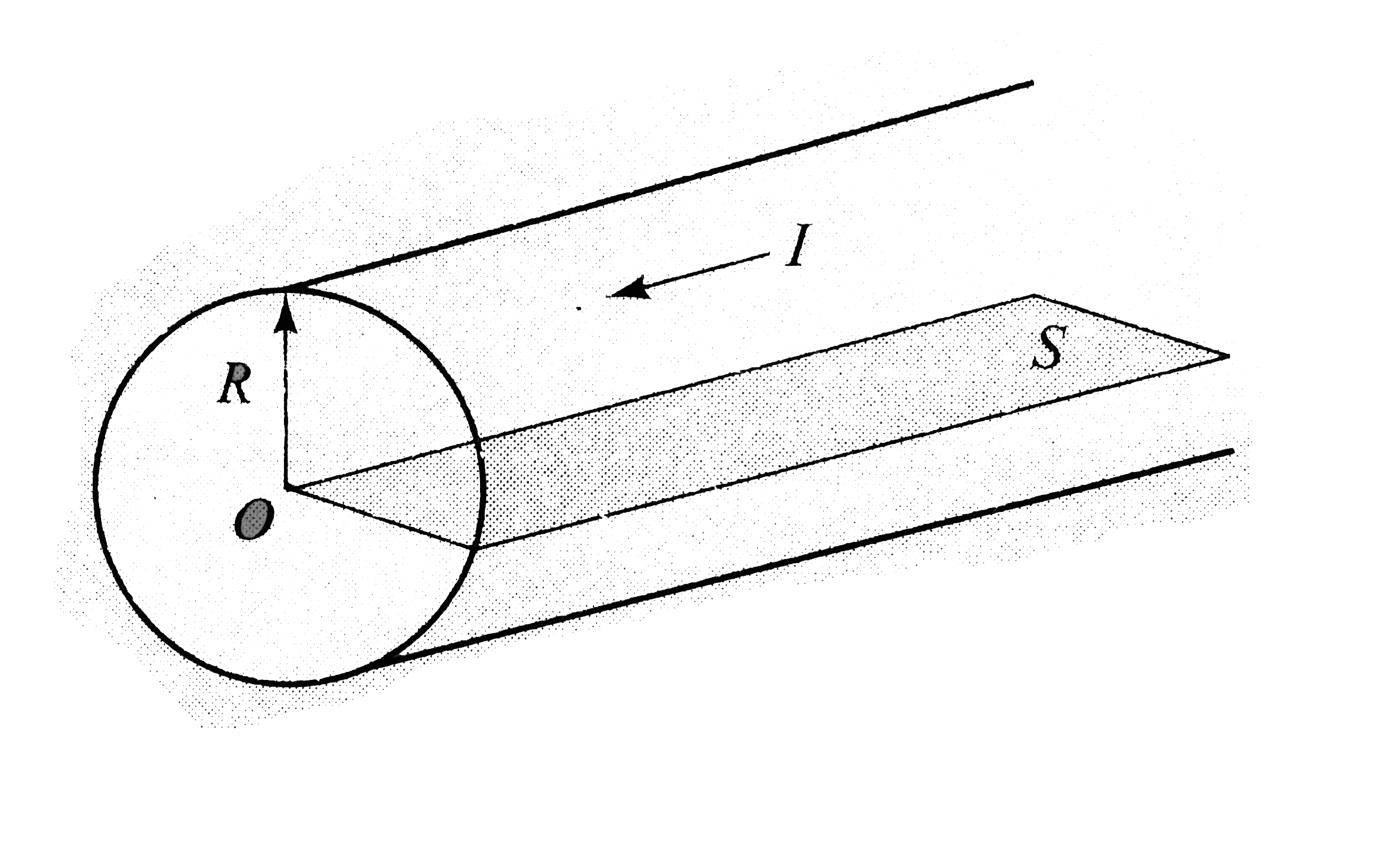
Consider an element of width dr at a distance r from the axis of the wire. The field due to the current I in the wire at the position of element will be B=μ0Ir2πR2.
And as its direction is perpendicular to the plane surface, flux linked with the element is given by
dϕ=Bdscosθ=μ02πIR2r(1dr)cosθ
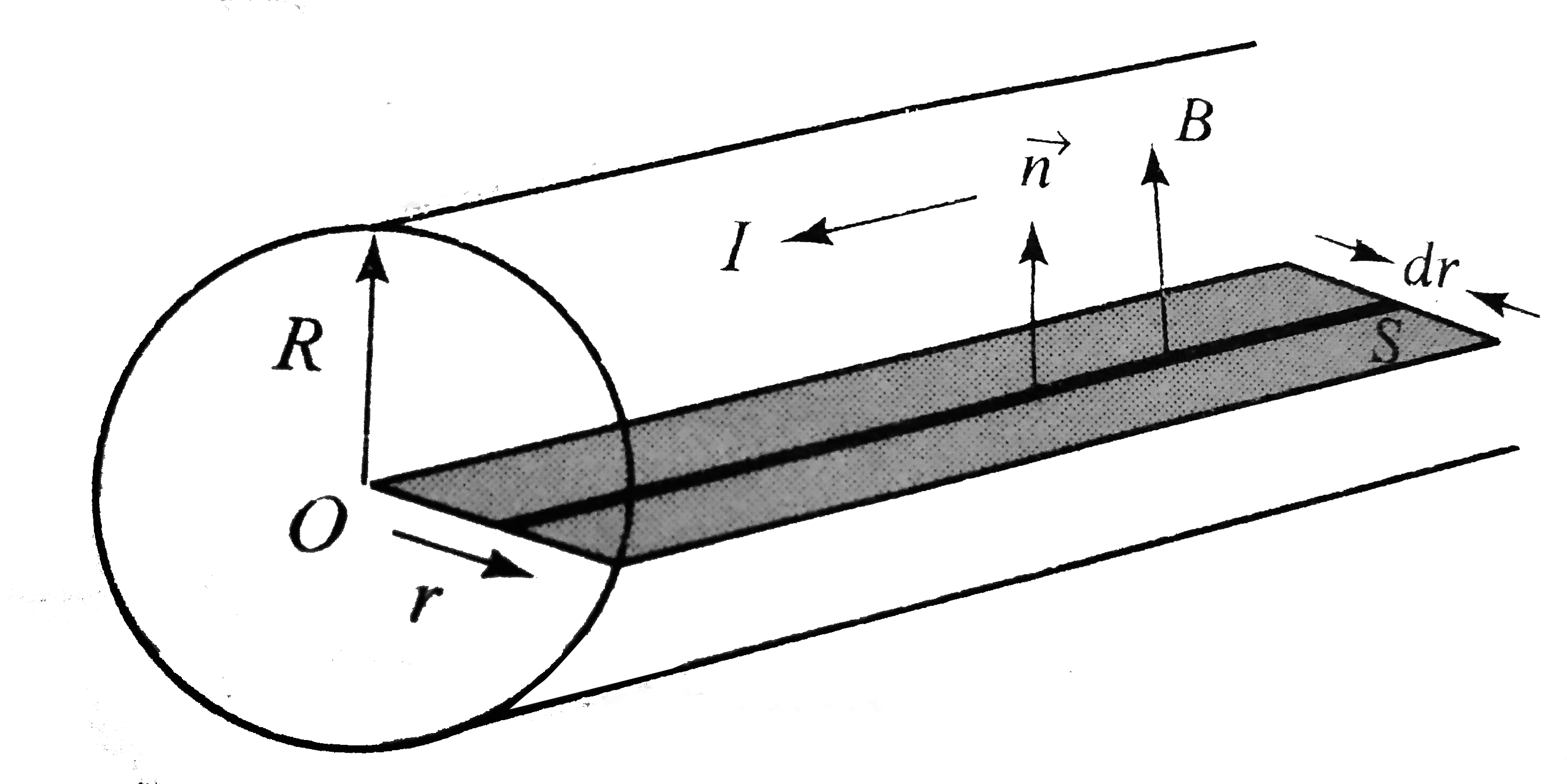
So, the flux linked with the plane surface is given by
ϕ=μ02πIR2∫R0rdr=μ0I4π
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Solved Example
5 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise 3.1
15 Videos
- ELECTROMAGENTIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGENTIC INDUCTIONEXERCISE - QUESTION BANK
40 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
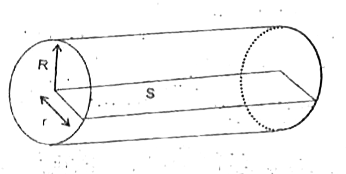
A long copper wire carries a current of 10 ampere . Calculate the magnetic flux per metre of the wire for a plane surface S inside the wire as shown in the figure.
View SolutionA long straight wire carries a current of 35A. What is the magnetic of the field →B at a point 20cm from the wire?
View Solution
Knowledge Check
A wire shaped to a regular hexagon of side x carries a current I ampere. Calculate the strength of the magnetic field at the centre of the hexagon.
A√3μ0IπxB3μ0IπxCμ0I3πxDμ0I√3πxA uniform copper wire carries a current i amperes and has p carries per meter3. The length of the wire is l metres and its cross-section area is s meter2. If the charge on a carrier is q coluombs, the drift velocity in ms−1 is given by-
Ai/lsqBi/psqCpsq/iDi/pslqA long straight wire carries a current of 10.05 A to the right. A circular loop of wire lies below the long straight wire, as shown in the following figure. The current in the long straight wire decreases to zero in a time of 4.652 s. What direction does the induced current flow around the circular loop of wire?
 ACounterclockwiseBClockwiseCNo induced current flows around the loopDThe information given is insufficient for determining the direction of the induced current
ACounterclockwiseBClockwiseCNo induced current flows around the loopDThe information given is insufficient for determining the direction of the induced current
A long, straight wire carries a current. Is Ampere's law
valid for a loop that does not enclose the wire, or that
encloses the wire but is not circular?
View SolutionA straight thick long wire of uniform cross-section of radius 5cm is carrying a steady current 5A. Calculate the magnetic field at a point 2cm from the axis of wire, well inside the wire.
View SolutionA0.5 m long conducting wire is bent in form of a circle, Calculate the magnetic field at the centre if wire carries a current of 5 A.
View SolutionTwo long, parallel copper wires of diameter 4.0 mm carry currents of 7.0 A in opposite directions. (a) Assuming that their central axes are 20 mm apart, calculate the magnetic flux per meter of wire that exists in the space between those axes. (b) What percentage of this flux lies inside the wires? (c) Repeat part (a) for parallel currents.
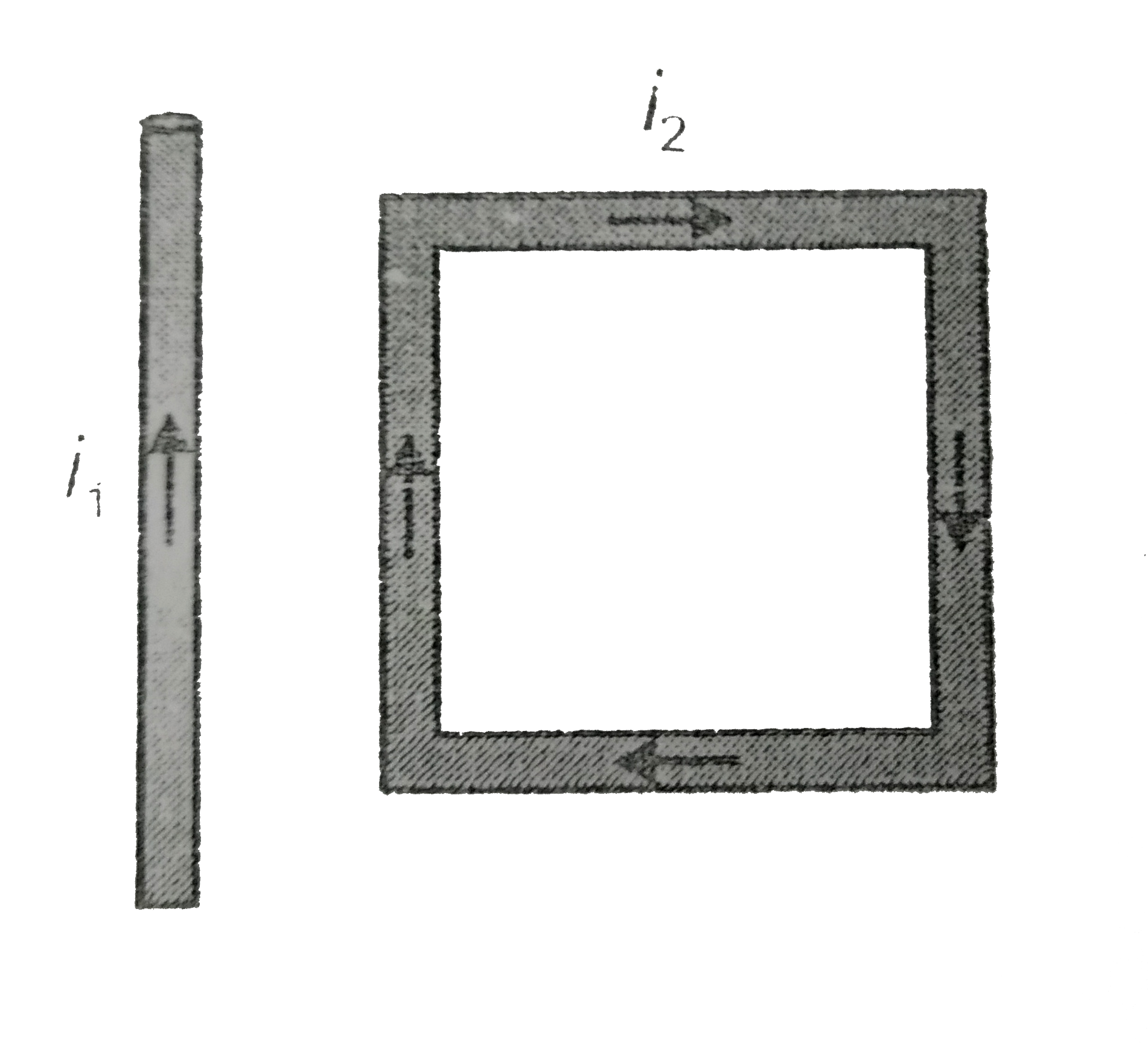
View SolutionA rectangular loop carrying a current i2 situated near a long straight wire carrying a steady current i1. The wire is parallel to one of the sides of the loop and is in the plane of the loop as shown in the figure. Then the current loop will
View Solution
