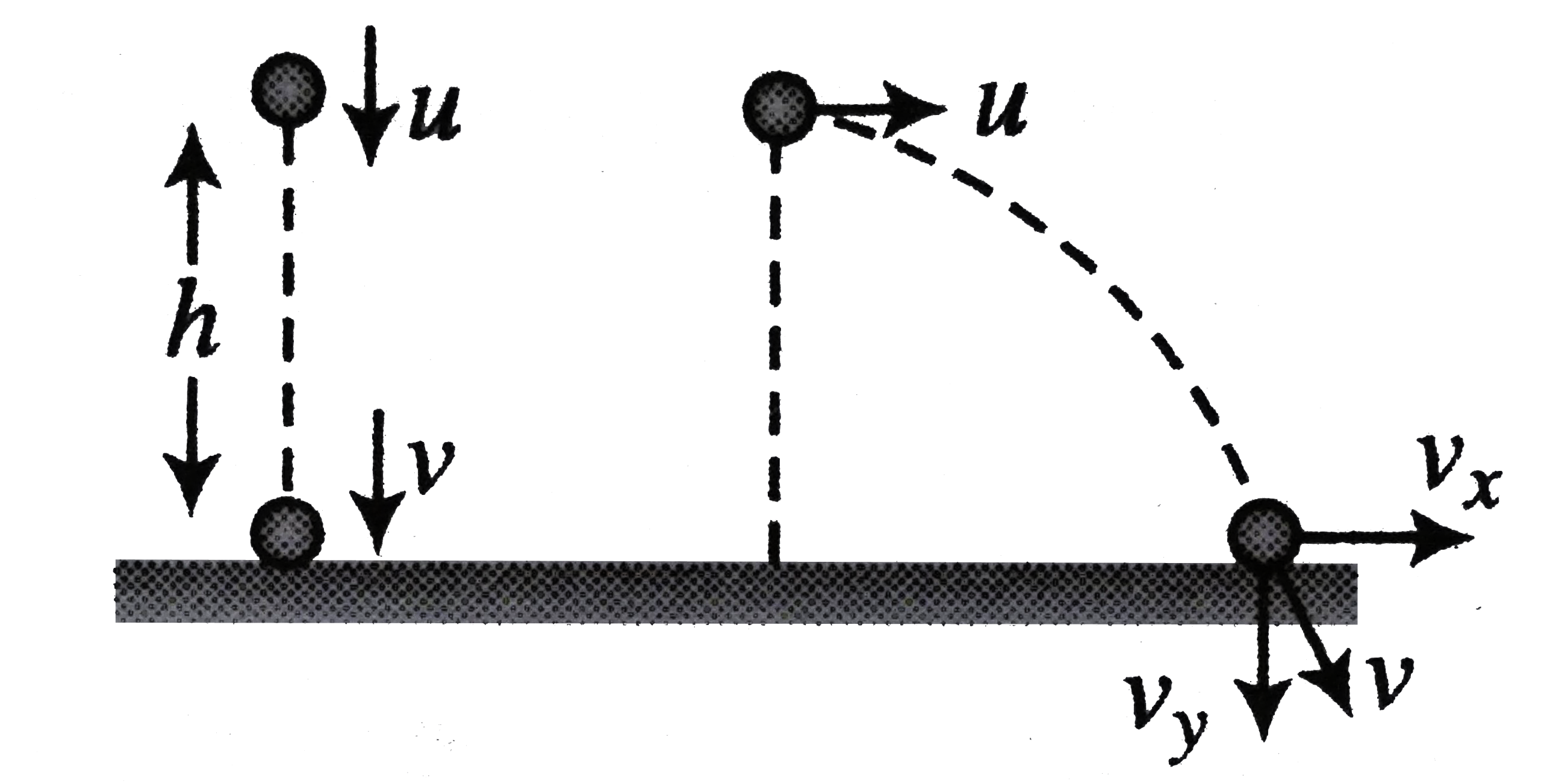
A man standing on the roof a house of height $h$ throws one particle vertically downwards and another particle horizontally with same velocity $u$ . Find the ratio of their velocities when they reach the earth's surface.

A

$4 : 1$

B

$3 : 1$

C

$1 : 2$

D

$1 : 1$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

For first particle : $v^{2} = u^{2} + 2 g h$
$\Rightarrow v = \sqrt{u^{2} + 2 g h})$
For second particle :
$v = \sqrt{v_{x}^{2} + v_{y}^{2}} = \sqrt{u^{2} + {(\sqrt{2 g h})}^{2}}$
= $\sqrt{u^{2} + 2 g h}$
So the ratio of velocities will be $1 : 1$ .
.

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A man standing on the roof of a house of height h throws one particle vertically downwards and another particle horizontally with the same velocity u . The ratio of their velocities when they reach the earth's surface will be
A $\sqrt{2 g h + u^{2}} : u$
B $1 : 2$
C $1 : 1$
D $\sqrt{2 g h + u^{2}} : \sqrt{2 g h}$
Question 2 - Select One
A person standing on the roof of a house of height h throws a particle vertically downwards and otherparticle in a horizontal direction with the same speed u. The ratio ofspeeds of the particles on reaching the earth is :
A $\sqrt{2 g h} : u$
B $1 : 2$
C $\sqrt{2} : 1$
D $1 : 1$
Question 3 - Select One
Two bodies of masses 5 kg and 80 kg are at same height if one is dropped vertically downward and another one horizontally with same initial velocity, which one will reach on earth sooner?
AHeavier
BLighter
CBoth on same time
DUpreductable

CENGAGE PHYSICS-KINEMATICS-2-Exercise 5.2

Home
Ask Doubt
Profile