A mass M is suspended from a massless spring. An additional mass m stretches the spring further by a distance x. The combined mass will oscillate with a period
2π ⎷{(M+m)xmg}
2π ⎷{mg(M+m)x}
2π ⎷{(M+m)mgx}
π2 ⎷{mg(M+m)x}
The correct Answer is:A
To find the time period of the oscillation of the combined mass suspended from a spring, we can follow these steps:
Step 1: Understand the forces acting on the system
When the additional mass m is added to the mass M, the total force acting on the spring is due to the weight of the combined mass, which is (M+m)g, where g is the acceleration due to gravity.
Step 2: Determine the spring constant k
The spring stretches by a distance x due to the additional mass m. At equilibrium, the force exerted by the spring (which is kx) must balance the weight of the additional mass mg:
kx=mg
From this, we can express the spring constant k:
k=mgx
Step 3: Write the formula for the time period of oscillation
The formula for the time period T of a mass-spring system is given by:
T=2π√mtotalk
where mtotal is the total mass attached to the spring.
Step 4: Substitute the total mass and spring constant into the time period formula
The total mass mtotal is M+m. Substituting k from Step 2 into the time period formula gives:
T=2π√M+mmgx
Step 5: Simplify the expression
This simplifies to:
T=2π√(M+m)xmg
Final Answer
Thus, the time period T of the oscillation of the combined mass is:
T=2π√(M+m)xmg
---
When the additional mass m is added to the mass M, the total force acting on the spring is due to the weight of the combined mass, which is (M+m)g, where g is the acceleration due to gravity.
The spring stretches by a distance x due to the additional mass m. At equilibrium, the force exerted by the spring (which is kx) must balance the weight of the additional mass mg:
kx=mg
From this, we can express the spring constant k:
k=mgx
The formula for the time period T of a mass-spring system is given by:
T=2π√mtotalk
where mtotal is the total mass attached to the spring.
The total mass mtotal is M+m. Substituting k from Step 2 into the time period formula gives:
T=2π√M+mmgx
This simplifies to:
T=2π√(M+m)xmg
Thus, the time period T of the oscillation of the combined mass is:
T=2π√(M+m)xmg
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective39videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Single Correct28videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Assertion And Reason10videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
Find the period of oscillation of the system shown in figure.
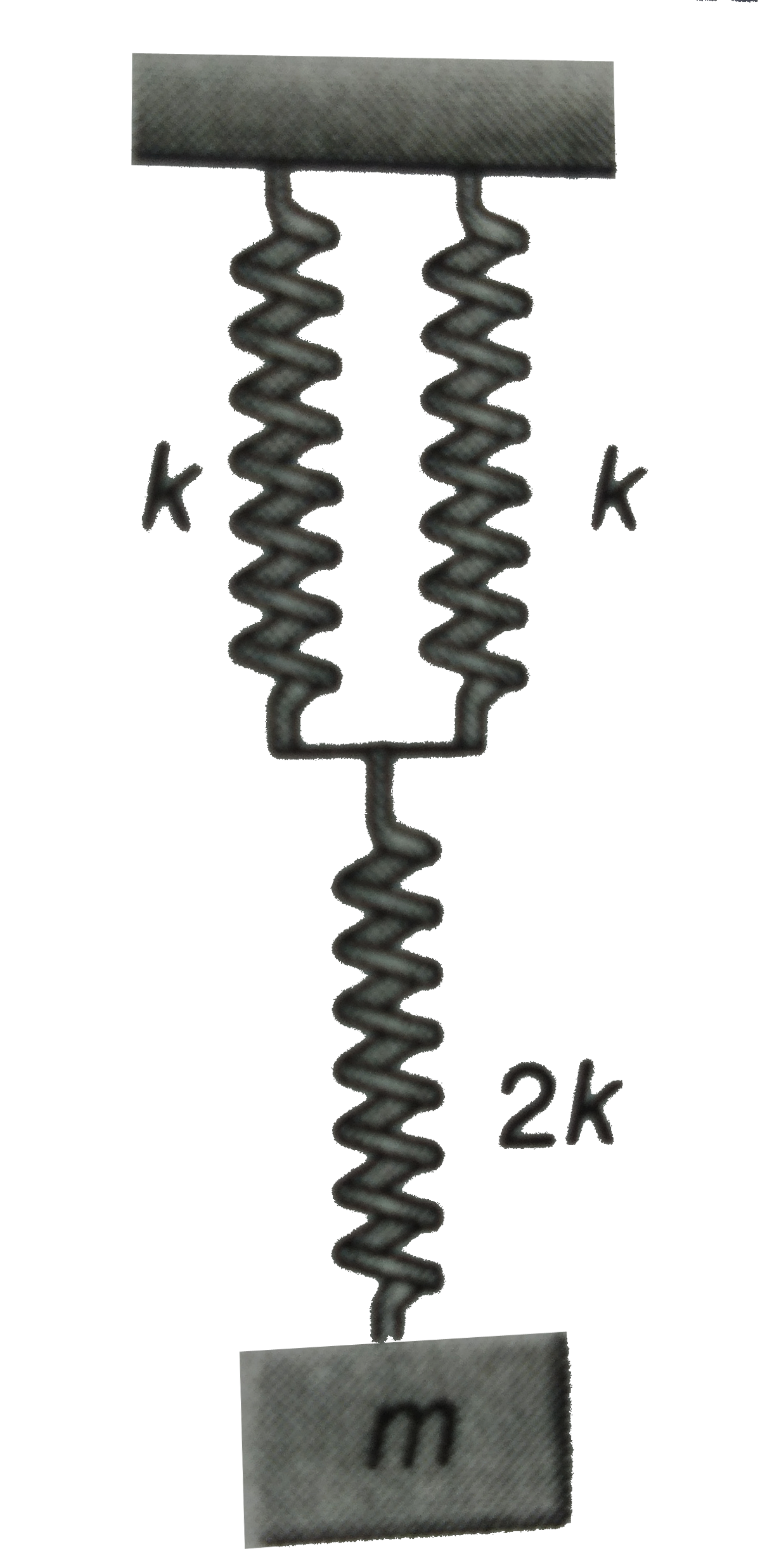 02:50View Solution
02:50View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is
 01:18View Solution
01:18View SolutionAssertion : In x=Acosωt, x is the displacement measured from extreme position.
Reason : In the above equation x=A at time t=0.03:15View SolutionWhen an electric field is applied across a semiconductor,
03:43View SolutionIf a machine perform 4000 J output work and 1000 J is inside loss due to friction , then the find efficiency?
03:31View SolutionA mass M is suspended from a massless spring. An additional mass m stretches the spring further by a distance x. The combined mass will oscillate with a period
02:43View SolutionA body of mass m is suspended from a massless spring of natural length l. It stretches the spring through a vertical distance y. The potential energy of the stretched spring is
03:04View SolutionA mass M is suspended from a light spring. An additional mass m added to it displaces the spring further by distance x then its time period is
03:04View SolutionThe period of oscillation of a mass M suspended from a spring of spring constant K is T. the time period of oscillation of combined blocks is
02:11View Solutionएक हल्की स्प्रिंग से M द्रव्यमान लटकाया जाता है। m द्रव्यमान और लटकाने पर इसमें दूरी x की अतिरिक्त वृद्धि हो जाती है। अब संयुक्त द्रव्यमान का इस स्प्रिंग पर दोलनकाल होगा।
01:02View SolutionThe period of oscillation of a mass M suspended from a spring of negligible mass is T. If along with it another mass M is also suspended, the period of oscillation will now be :
01:59View SolutionA mass M is suspended from a light spring. An additional mass m added displaces the spring further by a distance x. Now the combined mass will oscillate on the spring with a period :
01:59View SolutionA mass M is suspended from a light spring. An additional mass m added displaces the spring further by a distance X. Now the combined mass will oscillate on the spring with period.
07:04View SolutionThe period of oscillation of a mass m suspended from a spring of negligible mass is T. If along with it another mass m is also suspended the period of oscillation will now be……..
02:01View SolutionThe oscillation period of mass M suspended from a spring of negligible mass is T. If suspended too, now there will be oscillation period:
02:10View Solution

