A metal piece of density is suspended from a wooden hook by an weightless string. The tension in the string is What will be the tension in the string, if the system is immersed in a liquid at ? The surrounding temperature during the experiment is . At the specific gravity of the liquid is 1.24. The coefficients of volume expansion of the liquid and the metal are respectively.
Volume of the metal piece at ,
Volume at
Volume of the displaced liquid
Mass of displaced liquid
density of the liquid at
i.e., weight of the displaced liquid
upthrust
Tension in the string
Topper's Solved these Questions
- EXPANSION OF SOLIDS AND LIQUIDSBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF SOLIDS AND LIQUIDSEXERCISE - SECTION RELATED QUESTIONS
6 Videos
- EXPANSION OF SOLIDS AND LIQUIDSBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF SOLIDS AND LIQUIDSEXERCISE - HIGHGER ORDER THINKING SKILL (HOTS) QUESTIONS
31 Videos
- EXPANSION OF GASESBOOK - CHHAYA PUBLICATIONCHAPTER - EXPANSION OF GASESEXERCISE - CBSE Scanner
1 Videos
- FIRST AND SECOND LAW OF THERMODYNAMICSBOOK - CHHAYA PUBLICATIONCHAPTER - FIRST AND SECOND LAW OF THERMODYNAMICSEXERCISE - CBSE SCANNER
18 Videos
Similar Questions
A block of bras of mass 0.5 kg and density 8×103kg/m3 is suspended from a string. What will be the tension in the string if the block is completely immersed in water? (g=10ms−2)
View SolutionA 'block' of mass 10 kg is suspended with string as shown in figure. Find tension in the string. (g=10m/s2)
View Solution
Knowledge Check
A pices of metal weighs 46 g air. When it is immersed in a liquid of specific gravity 1.24 at 27∘C, it weighs 30g. When the temperature si raised to 42∘C, the metal piece weighs 30.5 g. If the specific gravity of the liquid at 42∘C is 1.20, the coefficient of linear expansion of the metal is
A(1.4×10−5)/∘CB(2.3×10−5)/∘CC(4.3×10−5)/∘CD(3.4×10−5)/∘CA 0.5kg block of brass (density 8×103Kgm−3) is suspended from a string. What is the tension in the string if the block is completely immersed in water? (g=10ms−2)
A5NB0.58×103NC58ND[5−58]NA wooden cylindrical block floats vertically with 70% of its volume immersed in a liquid at 0∘C . When temperature increases to 70∘C , the block sinks in the liquid. The coefficient of cubical expansion of liquid in K−1 is
A12.6×10−3K−1B61.2×10−3K−1C6.12×10−3K−1D6.00×10−3K−1
A 10 kg steel ball is suspended by two strings as shown. The tensions in the two strings are: (g=9.8 m/s2)
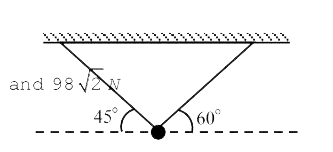
View SolutionA 10 kg steel ball is suspended by two strings as shown. The tensions in the two strings are: (g=9.8 m/s2)

View SolutionA metallic bob weights 50g in air. If it is immersed in a liquid at a temperature of 25∘C, it weights 45g . When the temperature of the liquid is raised to 100∘C, it weights 45.1g. Calculate the coefficient of cubical expansion of the liquid. Given that coefficient of cubical expansion of the metal is 12×10−6.∘C−1.
View SolutionA metallic bob weights 50g in air. If it is immersed in a liquid at a temperature of 25∘C, it weights 45g . When the temperature of the liquid is raised to 100∘C, it weights 45.1g. Calculate the coefficient of cubical expansion of the liquid. Given that coefficient of cubical expansion of the metal is 12×10−6.∘C−1.
View SolutionThe density of a liquid at 100∘C is 8.0g/cm3 and at 0∘C is 8.4g/cm3, the coefficient of cubical expansion of the liquid is
View Solution
