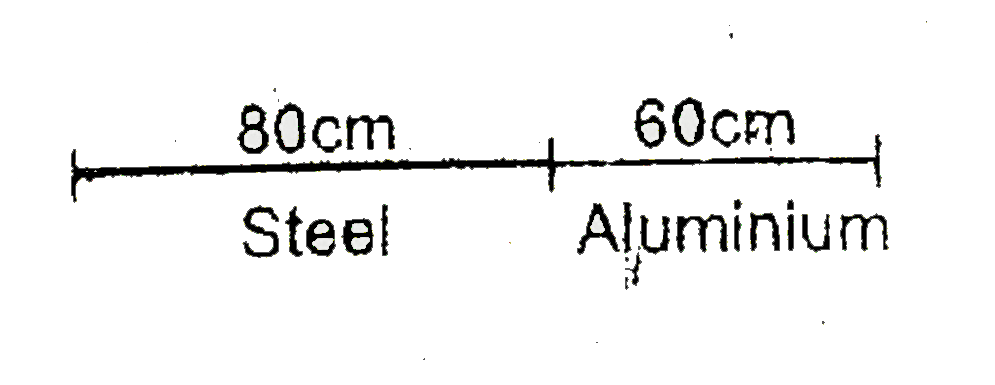
A metal wire of length 80 cm, area of cross-section 3 mm2 and material density 3000 kg m−3 is joined to another metal wire of length 60 cm, area of cross-sectional 1 mm2 and material density 9000 kg−m3.
The free ends of the two wires are stretched between two rigid supports and a tension of 40 N is produced in the wires. The minimum frequency of the tuning fork which can produce stationary waves with the joint of the wires as a node isSolution in Telugu
2003Hz
4003Hz
5003Hz
70C3Hz
The correct Answer is:C
Given, l1=80cm=80×10−2m
Al=3mm2=3×10−6m2
ρ1=3000kg.m−3
l2=60cm=60×10−2m
A2=1mm2=10−6m2
ρ2=9000kg.m−3⇒T=40N
According to the question,
for stationary wave to be formed, frequency of P th harmonic due ot Ist metal wise = frequency of Q th harmonic due to 2nd metal wire
P×12l1√Tρ1A1=Q×12l2√Tρ2A2
or PQ=l1l2√ρ1A1ρ2A2=80×10−260×10−2√3000×3×10−69000×10−6
or PQ=43
Hence, p is 4th harmonic and Q is 3rd harmonic. So, the minimum frequency of the tuning fork, E0 produce stationary waves with given wire of 2 metals, frequency of tuning fork = frequency of Pth harmonic/Q th harmonic of 2 respective wire.
n=P×12l1√Tρ1A1
=4×12×80×10−2√403000×3×10−6=5003Hz
Topper's Solved these Questions
Similar Questions
Two intervals of time are measured as Δt1=(2.00±0.02)sandΔt2(4.00±0.02)s. The value of √(Δt1)(Δt2) with correct significant figures and error is
View SolutionA nucleus of deuterons or deuterium is a bound atomic system best described by
View SolutionFigure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is . 1.0 mm^2 and that of the aluminium wire is 3.0 mm 2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node ? The density of aluminium is 2.6 g cm^-3 and that of steel is 7.8gcm^-3
View SolutionCalculate the speed of a transverse wave in a wire of 1.0mm2 cross-section under a tension of 0.98N. Density of the material of wire is 9.8×103kg/m3
View SolutionCalculate the fundamental frequency of a sonometer wire fo length 20cm, tension 25N, cross sectional area 10^(-2)cm^(2) and density of material =10^(4)kg//m^(3) .
View SolutionA steel wire of length 60 cm and area of cross section 10^(-6)m^(2) is joined with a n aluminimum wire of length 45 cm and are of cross section 3xx10^(-6)m^(2) . The composite string is stretched by a tension of 80 N. Density of steel is 7800kgm^(-3) and that of aluminimum is 2600kgm^(-3) the minimum frequency of tuning fork. Which can produce standing wave in it with node at joint is
View SolutionA metal wire of length jl and area of cross - section A is fixed between rigid supports negligible tension . If this is cooled. The tension in the wire will be
View SolutionFigure shows an aluminium wire of length 60cm joined to a steel wire of length 80cm and stretched between two fixed supports. The tension produced is 40N. The cross-sectional area of the steel wire is 1.0mm2 and that of the aluminimum wire is 3.0mm2 The minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node is 10P (in Hz) the find P. Given density of aluminimum is 2.6g/cm3 and that of steel is 7.8g/cm3.
View SolutionThe speed of a trasnsverse wave along a unifrom metal wire, when it is under at tension of 1 kg wt, is 68 m/s . If the density of the metal is 7900 Kg//m^(3) . Find the area of cross section of the wire.
View SolutionTwo wires of the same material and same cross sectional area are vibrating with the same frequency. The first wire of length 80 cm is loaded with 8 kg and the second wire is loaded with 2 kg. The length of the second wire is
View SolutionWork done in stretching a wire of length 1 m and of cross-sectional area 1mm2 through 2 mm if Young's modulus of the material of the wire is 2×1011N/m2 is .......
View SolutionCalculate the speed of a transverse wave in a wire of 1.0mm2 cross-section under a tension of 0.98 N. Density of the material of wire is 9.8×103kg/m3.
View SolutionWhen a wire 2 m long and 0.05 cm^(2) in cross-section is stretched by a mass of 2 kg, it increases in length by 0.04 mm. Young's modulus of the material of the wire is (g=10ms^(-2))
View SolutionCalculate the speed of a transverse wave in a wire of 1.0mm2 cross-section under a tension of 0.98N. Density of the material of wire is 9.8×103kg/m3
View SolutionAn aluminium wire of length 60 cm is joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40N. Cross sectional area is 1 mm^2 (steel) and 3 mm^2 (aluminium). Minimum frequency of the tuning fork which can produce standing waves with the joint as a node is (density of Al= 2.6 g cc^(-1) ) and density of steel =7.8 g cc^(-1))
View Solution
