Question

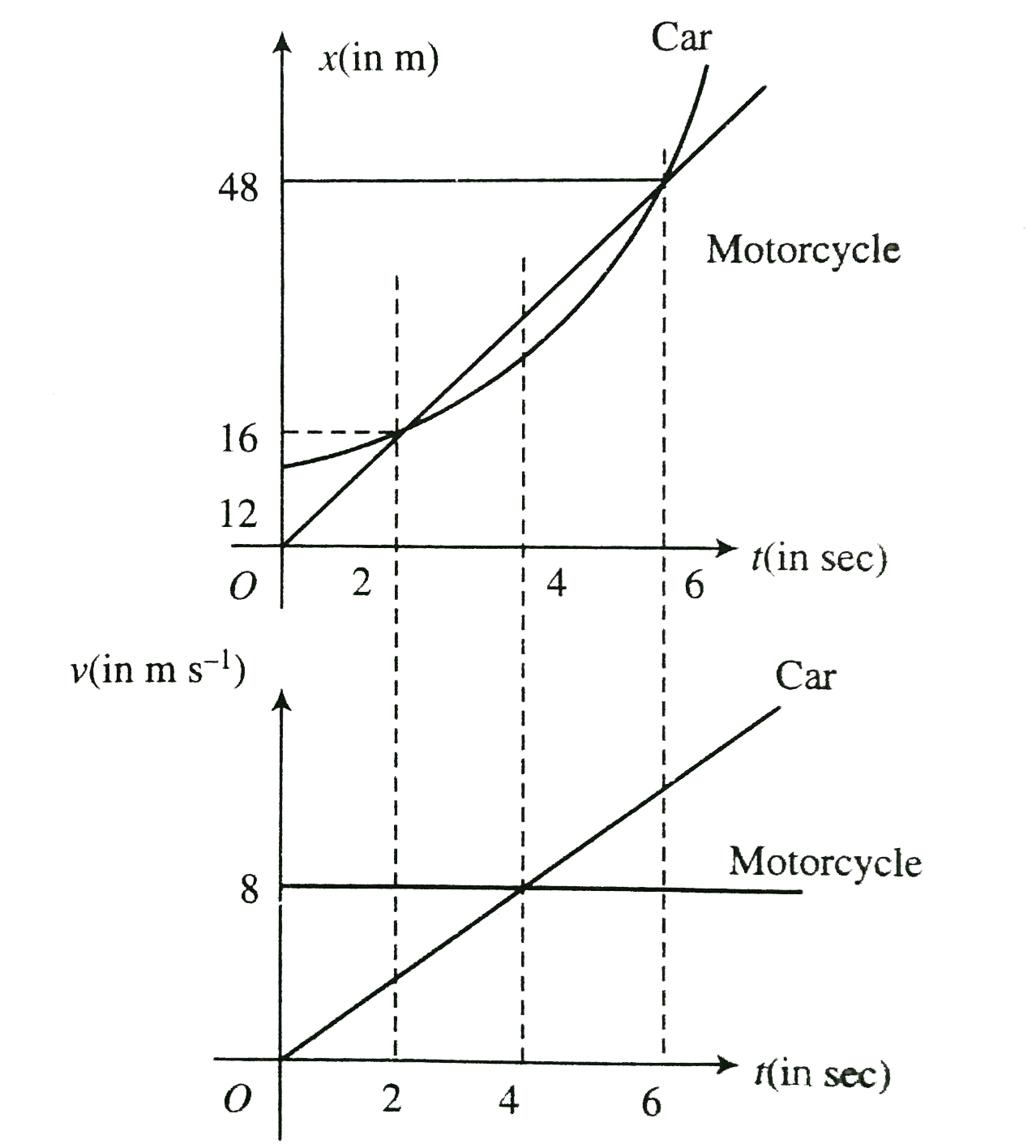
A motorcyclist situated at origin is located at a distance ${\displaystyle 12m}$. Behind a car (Fig. 4.150).
.
At${\displaystyle t=0}$ the motorcyclist stars moving with a constant velocity ${\displaystyle v=8m{s}^{-1}}$ and same time the car starts acceleration from rest with ${\displaystyle a=2ms\&(-2)}$, (a) When and wher do they meet?

Explanation

Let the car and motorcycle meet, after a time `t`. During the time, the motorcycle comers a distance `d_(m)` say. Position of motorcycle, `x_(m)=d_(m)=vt` ..(i)
During the time `t` the car moves through a distance
`d_(c)=(1)/(2)at^(2)`
Position of car, `x_(c)=12 +d_(c) =12 +(1)/(2)at^(2)` ...(ii)
When both motorcycle and car meet their positions will be same.
From (i) and (ii) `x_(m)=x_(c)`.
We have `vt=12+(1)/(2) at^(2)`
Then substituting `v=8 m s^(-1)` and `a=2 m s^(-2)`, We have
`r^(2)-8t+ 12 =0`
.
This yields two real values of time, i.e., `t-2 s` and `6 s`.
The following conclusion can be made from the above mathematical proceedings.
At ``t=2 s` the motorcycle approaches the car.
The position of motorcyclist
`(x_(m))_(t=2s)=8 xx2 =16 m`
At `t=6 s`, the car overtakes the motorcycle.
At `t=2 s`, the motorcycle overtakes the car. The it diverges (moves away from the car )till the acquires a velocity equal to that of motorcycle at `t=4 s`. Then the distance jof separation will gradually decreases as the car moves faster than the motorcycle. After that, the car will take a lead leaving the motorcyle behind it.