A particle at the end of a spring executes S.H,M with a period t2 If the period of oscillation with two spring in .
(a) T−1=t11+t−12
(b) T2=t21+t22
(c )T=t1+t2
(d) T^(-2)=t_(1)^(-2)+t_(2_^(2)
The correct Answer is:B
(b) For first spring, t_(1)=2pisqrtm/k_(1),<br>Forsecondspr∈g,t_(2)=2pisqrtmk_(2).<br>whenspr∈gsare∈seriesthen,(k_(1)k_(2))/(k_(1)k_(2)<br>∴T=2pisqrt((m(k_(1)+k_(2))))/((k_(1)k_(2).<br>∴T=2pisqrtm/k_(2)+m/k_(1) =2pisqrt((t_(2)^(2))/(2pi^(2)+t_(1)^(2)/(2pi)^(2))<br>rArr T^(2)=t(1)^(2)+t_(1)^(2).
where x is the displacement from the mean position.
Topper's Solved these Questions
Similar Questions
A particle connected at the end of a spring executes SHM with a period T1. While the corresponding period for another spring is T2. When the two springs are connected inseries and particle is ocnnected at the one T. Find the relation between T, T1 and T2
View SolutionA particle at the end of a spring executes simple harmonic motion with a period t1 while the corresponding period for another spring is t2 if the oscillation with the two springs in series is T then
View Solution
Knowledge Check
A body at the end of a spring executes SHM with a period t1, while the corresponding period for another spring is t2, If the period of oscillation with the two spring in series is T, then
AT=t1+t2BT2=t21+t22C1T=1t1+1t2D1T2=1t21+1t22A particle at the end of a spring executes simple harmonic motion with a period t1 while the corresponding period for another spring is t2 if the oscillation with the two springs in series is T then
AT2=t21+t22BT=t1+t2CT−1=t−11+t−12DT−2=t−21+t−22The time period of oscillation of a particle in S.H.M. depends
Anot upon its mass,Bupon its mass,Cupon amplitude of the S.H.M.Dupon phase of the particle.
A mass is suspended at the end of a spring and performs SHM with a time period 3 seconds, while the time period f same mass with other spring is 4 second. If that mass is connected with the combination of the two springs connected in series then time period of oscillator is
View SolutionA body of mass 0.4 kg is held between two massless springs whose ends are fixed to rigid walls as shown in the figure. The spring constants are 8 N/m and 2 N/m. If the body is displaced along the direction of the lengths of the springs and released, the time period (in second) of the oscillations will be (ignore vertical oscillation, if any )
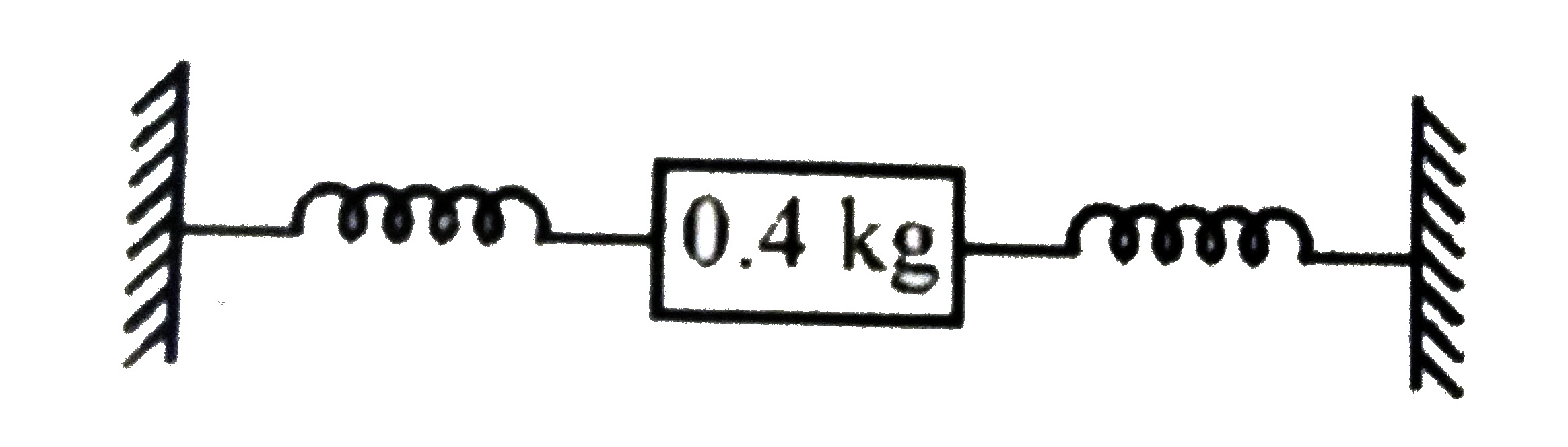
View SolutionWhen a boy is playing on a swing in the sitting position , the time period of oscillations of the swing is T. If the boy stands up , the time period of oscillation of the spring will be
View SolutionA simple harmonic oscillator consists of a particle of mass m and an ideal spring with spring constant k. The particle oscillates with a time period T. The spring is cut into two equal parts. If one part oscillates with the same particle, the time period will be
View SolutionA block of mass M suspended from a spring oscillates with time period T. If spring is cut in to two equal parts and same mass M is suspended from one part, new period os oscillation is
View Solution
