A particle moves along a circle of radius R with a constant angular speed . Its displacement (only magnitude) in time t will be
vt
The correct Answer is:D
Topper's Solved these Questions
Similar Questions
A particle moves along an arc of a circle of radius R according to the law l=asinωt, where l is the displacement from the initial position measured along the arc, and a and ω are constants. Assuming R=1.00m, a=0.80m, and ω=2.00rad/s, find:
(a) the magnitude of the total acceleration of the particle at the points l=0 and l=±a,
(b) the minimum value of the total acceleration wmin and the corresponding displacement lm.
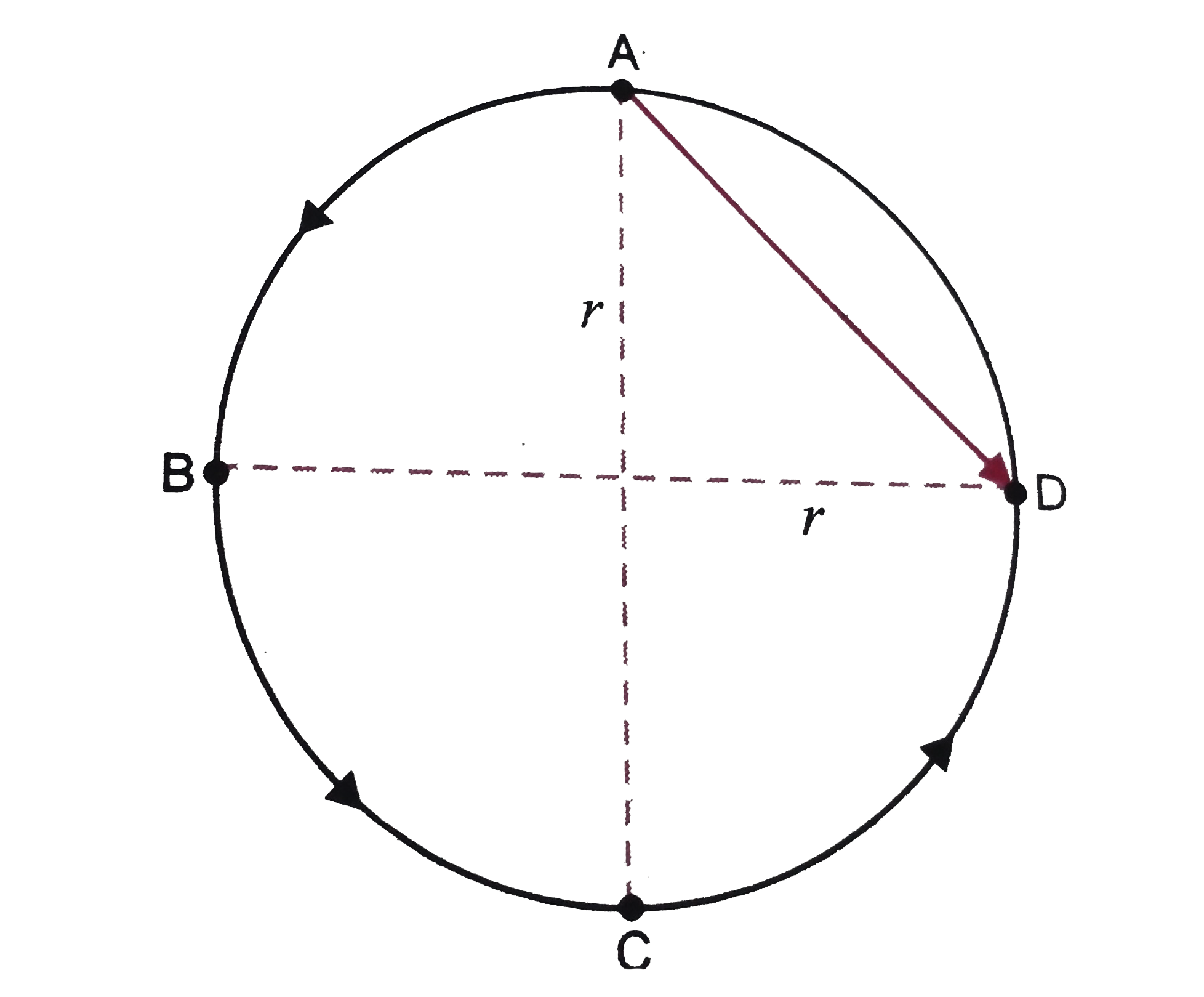
View SolutionA particle moves over three quarters of a circle of radius r. What is the magnitude of its displacement ?
View Solution
Knowledge Check
A particle moves along a semicircular path of radius R in time t with constant speed. For the particle calculate
Adistance travelledBdisplacementCaverage speedDaverage velocityA particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
Arω2Br/ωCzeroDω/rA particle is moving in a circle with a constant speed. Its moving is
APeriodicBOscillatoryCSimple harmonicDboth 'a' and 'b'
A particle moves over three quarters of a circle of radius r. What is the magnitude of its displacement ?

View SolutionAn α is moving along a circle of radius R with a constant angular velocity ω. Point A lies in the same plane at a distance 2R from the centre. Point A records magnetic field produced by the α-particle. If the minimum time interval between two successive time at which A records zero magnetic field is t, the angular speed ω, in terms of t, is
View SolutionA particle moves in circle of radius R with a constant speed v. Then, find the magnitude of average
acceleration during a time interval πR2v.
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View Solution
