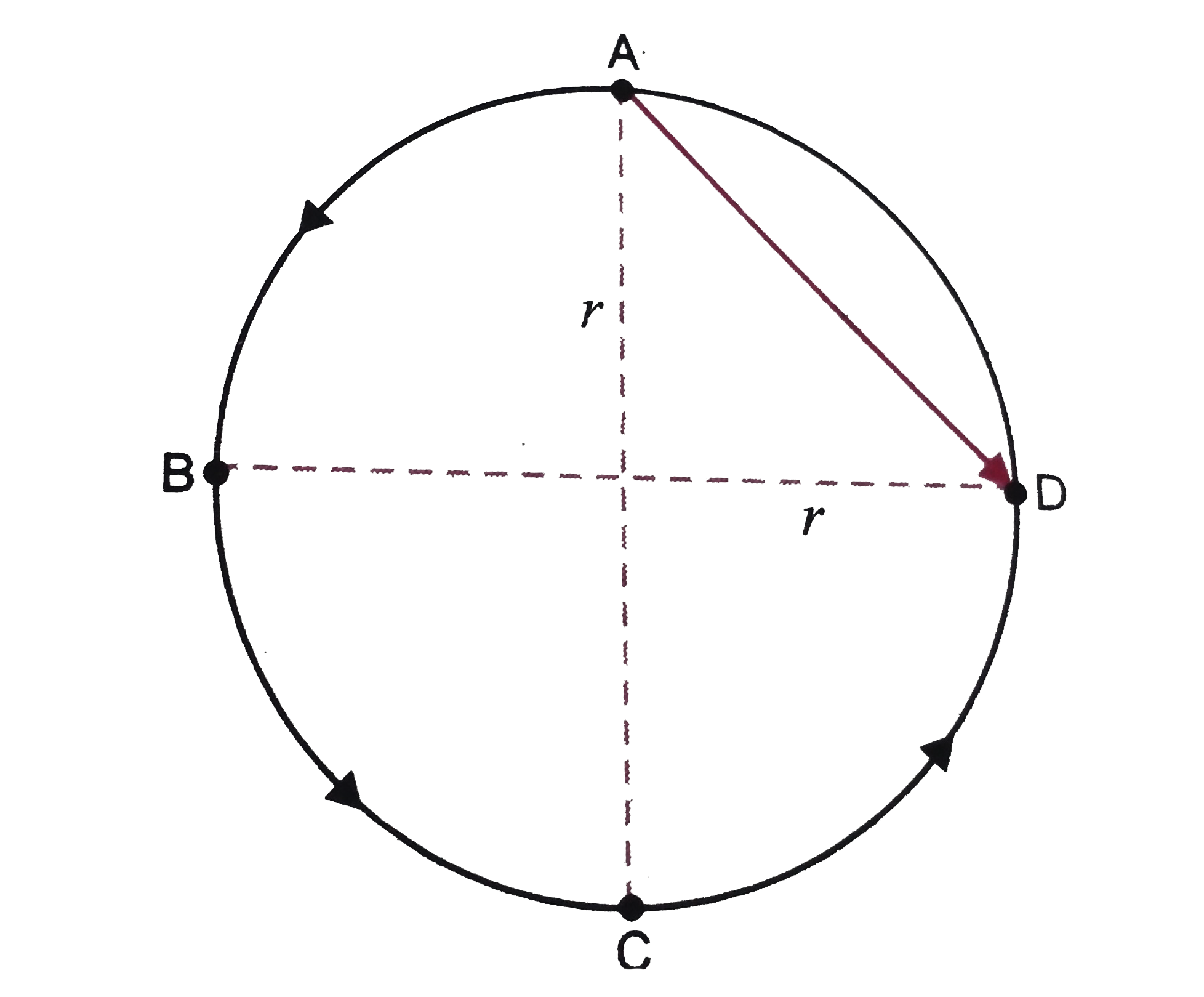
A particle moves over three quarters of a circle of radius $r$ . What is the magnitude of its displacement ?

Video Solution

Text Solution

Verified by Experts

When the particle goes from A to D, over three quarters of a circle
Displacement $A D = \sqrt{r^{2} + r^{2}} = r \sqrt{2}$

|

Updated on:21/07/2023

Class 9 PHYSICS QUESTION BANK

Topper's Solved these Questions

BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - Section - B
12videos
FOOTSTEPS TOWARDS CBSE BOARD
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS CBSE BOARDEXERCISE - Section - C
16videos
FOOTSTEPS TOWARDS CBSE BOARD
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS JEE MAIN EXERCISE - Section B (Numerical Value Type Questions)
10videos
FOOTSTEPS TOWARDS JEE MAIN
BOOK - MTG IIT JEE FOUNDATIONCHAPTER - FOOTSTEPS TOWARDS NEETEXERCISE - Multiple Choice Question
45videos
FOOTSTEPS TOWARDS NEET

Similar Questions

Knowledge Check

Question 1 - Select One
A particle moves on the circumference of a circle of radius r. Starting from one point particle reaches to its diametric end in time t.The magnitude of average velocity of the particle is
A $\frac{π r}{t}$
B $\frac{π f}{2 t}$
C $\frac{r}{t}$
D $\frac{2 r}{t}$
Question 2 - Select One
A particle moves along a circle of radius R with a constant angular speed $ω$ . Its displacement (only magnitude) in time t will be
A $ω t$
B $2 R cos ω t$
C $2 R sin ω t$
D $2 R sin . \frac{ω t}{2}$
Question 3 - Select One
A particle moves along a circle of radius $R$ with a constant angular speed $ω$ . Its displacement (only magnitude) in time $t$ will be
A $ω t$
B $2 R cos ω t$
C $2 R sin ω t$
D $2 R sin \frac{ω t}{2}$

MTG IIT JEE FOUNDATION-FOOTSTEPS TOWARDS CBSE BOARD-Section - D

Home
Ask Doubt
Profile