A particle of charge −16×10−18coo–mb moving with velocity 10ms−1 along the x−aξs, and an electric field of magnitude (104)/(m) is along the negative z−ais. If the charged particle continues moving along the x−aξs , the magnitude of B is
(103Wb)/(m2)
(105Wb)/(m2)
(1016Wb)/(m2)
(10−3Wb)/(m2)
The correct Answer is:A
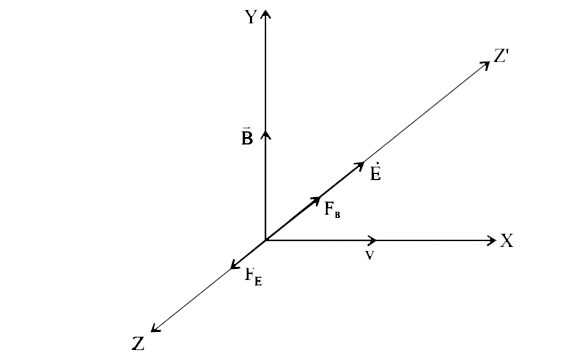
The situation is shown in the figure .
FE=Forcedue→e≤ctricfield
FB=Forcedue→mag≠ticfield
It is given that the charged particle remins moving along X−aξs( i.e. undeviated). Therefore FB=FE
rArr qvB = qE rArr B = (E)/(v) = (10^(4))/(10) = 10^(3) weber//m^(2)
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - MCQs(d )
1 Videos
- MODERN PHYSICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - MODERN PHYSICSEXERCISE - MCQ (One Correct Answer
1 Videos
- RAY AND WAVE OPTICSBOOK - SUNIL BATRA (41 YEARS IITJEE PHYSICS)CHAPTER - RAY AND WAVE OPTICSEXERCISE - JEE Main And Advanced
209 Videos
Similar Questions
A particle of charge −16×10−19C moving with velocity 10mg−1 along the x -axis ẹnters a region where magnetic field B is along the y axis and electric field of magnitude 104 (V/m) is along the negalive z-axis If the charged particle contintes moving along the x -axis, then the magnitude of B(Wb(m)2) is
View SolutionA particle of charge −1.6×10−19C moving with velocity 10ms−1 along the X-axis enters a region of magnetic field of induction B along V-axis and an electric field of strength 104V/m along negative Z-axis. If the charged particle continues to move along the X-axis, the magnitude of B is
View Solution
Knowledge Check
A particleofcharge −16×10−18 coulombmoving with velocity 10 m s−1 along the x-axis enters a region where a magnetic field B is along the y-axis, and an electric field of magnitude 104 V/m is along the negative z-axis. If the charged particle continues moving along the x-axis, the magnitude of B is
A103Wb/m−2B105Wb/m−2C1016Wb/m−2D10−3Wb/m−2A particle of charge −32×10−18 coulomb moving with velocity 20 ms−1 along the x-axis enters a region where a magnetic field of induction B is along the y-axis, and an electric field of magnitude 5×104 V/m is along the negative z-axis. If the charged particle continues moving along the x-axis, the magnitude of B is
A7×103Wb/m2B2.5×103Wb/m2C104Wb/m2D5×10−3Wb/m2A particle of charge 16×10−18 coulomb moving with velocity 10m/s along the x− axis enters a region where a magnetic field of induction B is along the y− axis, and an electric field of magnitude 101/m−1 is along the negative Z− axis. If the charged particle continues moving along the X− axis, the magnitude to B is
A1Wb/m2B105Wb/M2C106Wb/m2D10−3Wb/m2
A charged particle carrying charge q=10μC moves with velocity v1=106,s−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
Find the magnitude of the force F2.
View SolutionA proton is moving with a uniform velocity of 106ms−1 along the Y-axis, under the joint action of a magnetic field along Z-axis and an electric field of magnitude 2×104Vm−1 along the negative X-axis. If the electric field is switched off, the proton starts moving in a circle. The radius of the circle is nearly (given em ratio for proton =108Ckg−1)
View SolutionA charged particle carrying charge q=10μC moves with velocity v1=106,s−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
Find the magnetic field →B.
View SolutionA particle with 10−11 coulomb of charge and 10−7kg mass is moving wilth a velocity of 108m/s along the y-axis. A uniform static magnetic field B=0.5 Tesla is acting along the x-direction. The force on the particle is
View SolutionA charged particle is moving along a magnetic field line. The magnetic force on the particle is
View Solution
