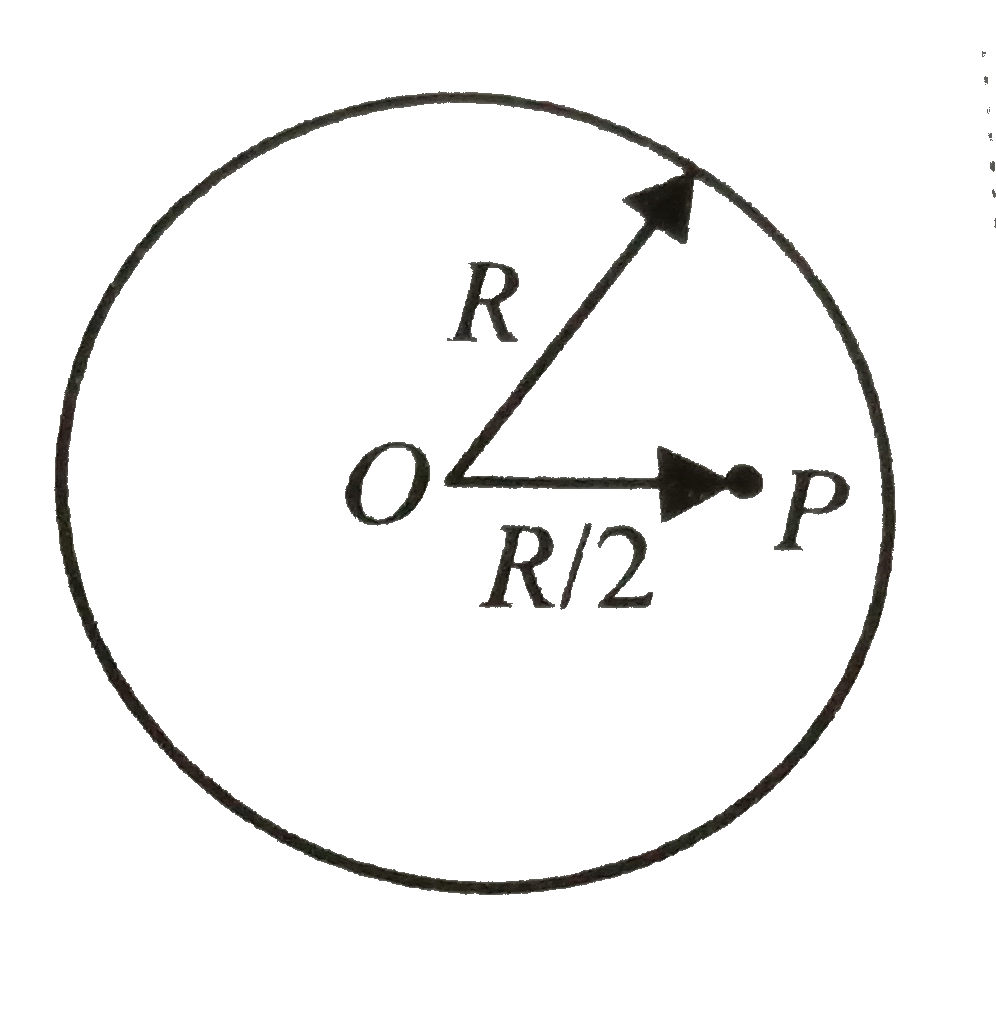
A particle of mass M is placed at the centre of a spherical shell of same mass and radius a. What will be the magnitude of the gravitational potential at a point situated at a/2 distance from the centre ?
−3GMa
−2GMa
−GMa
−4GMa
The correct Answer is:A
(a) Here mass of the particle=M
Mass of the spherical shell=M
Radius of the spherical shell = a
Let O be centre of spherical shell. Gravitational potential at point P due to particle at O is
V1=−GM(a/2)
Gravitational potential at point P due to spherical shell is V2=−GMa
Hence, total gravitational potential at the point P is V=V1+V2
=−GM(a/2)+(−GMa)=−2GMa−GMa=−3GMa
Topper's Solved these Questions
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Escape Speed
7 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Earth Satellite
4 Videos
- GRAVITATIONBOOK - NCERT FINGERTIPSCHAPTER - GRAVITATIONEXERCISE - Acceleration Due To Gravity Below And Above The Surface Of Earth
8 Videos
- KINETIC THEORYBOOK - NCERT FINGERTIPSCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
Similar Questions
A particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View SolutionA mass of 50 kg is placed at the centre of a uniform spherical shell of mass 100 kg and radius 50 m. If the gravitational potential at a point, 25 m from the centre is V kg/m. The value of V is:
View Solution
Knowledge Check
A particle of mass M is situated at the centre of a spherical shell of same mass and radius 'a'. The gravitational potential at a point situated at a2 distance from the centre, will be
A−3GMaB−2GMaC−GMaD−4GMaA particel of mass M is placed at the centre of a inform spherical shell of mass 2M and radius R. The gravitational potential on the surface of the shell is
A−GMRB−3GMRC−2GMRDzeroA particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
A−GmRB−3GmRC−4GmRD−2GmR
P is a point at a distance r from the centre of a spherical shell of mass M and radius a, where r<a. The gravitational potential at P is
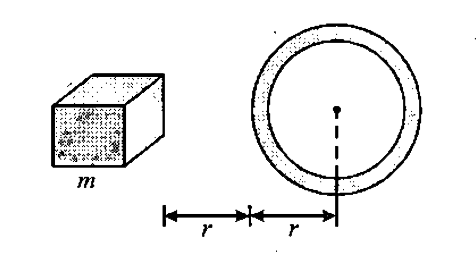
View SolutionA block of mass m is lying at a distance r from a spherical shell of mass m and radius r, then :
View SolutionA particle of mass M is at a distance a from surface of a thin spherical shell of equal mass and having radius a.

View SolutionA body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
View SolutionA particle of mass m is located inside a spherical shell of mass M and radius R. The gravitational force of attraction between them is
View Solution
