A particle of mass m is projected at t=0 from the point P on the ground with speed v0 at an angle of θ to the horizontal. Find the magnitude and direction of the angular momentum of the particle at time t=v0sinθ2g.
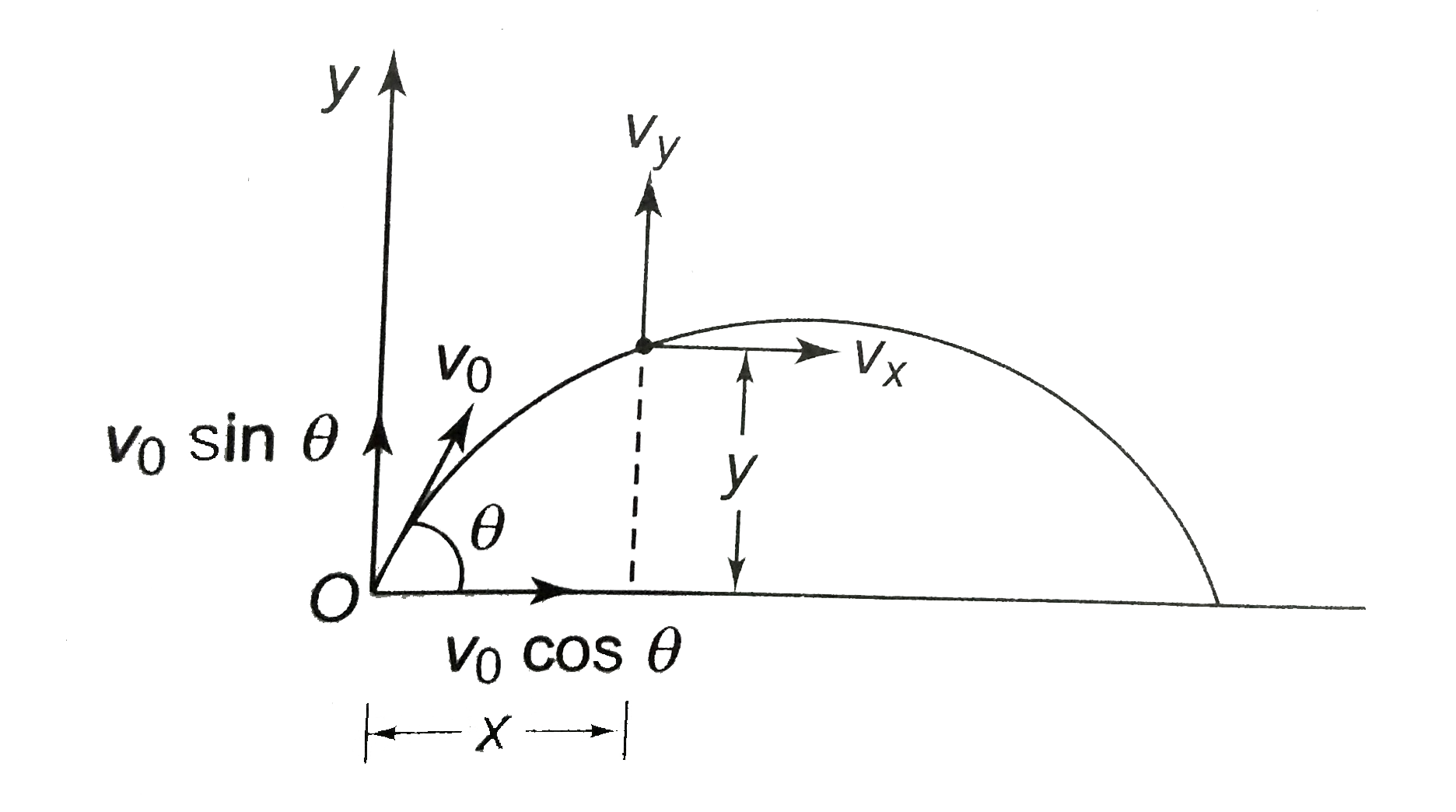
t=v0sinθ2g
x:vx=v0cosθ
x=v0cosθt=v0cosθ×v0sinθ2g=v20sinθcosθ2g
y:vy=v0sinθ−gt
=v0sinθ−g×v0sinθ2g=v0sinθ2
y=v0sinθt−12gt2
=v0sinθ×v0sinθ2g−12g(v0sinθ2g)2
=v20sin2θ2g−v20sin2θ8g=38v20sin2θg
L=mvxy−mvyx
=m(v0cosθ×38v20sin2θg−v0sinθ2×v20sinθcosθ2g)
=nv30sin2θcosθ8g
OR
In vector form
→v=vxˆi+vyˆj,→r=xˆi+yˆj
→L=→r×→p=→r×m→v=m∣∣
∣
∣∣ijkxyovxvyo∣∣
∣
∣∣
=m(xyy−yvx)ˆk
=m[v20sinθcosθ2×v0sinθ2−38×v20sin2θg×v0cosθ]ˆk
m=[−18v30sin2θcosθg]ˆk~
→L=−mv30sin2θcosθ8gˆk
L=∣∣→L∣∣=mv30sin2θcosθ8g
Topper's Solved these Questions
Similar Questions
A particle is projected at time t=0 from a point P on the ground with a speed v0, at an angle of 45∘ to the horizontal. Find the magnitude and direction of the angular momentum of the particle about P at tiem t=v0/g
View SolutionThe particle of mass m is projected at t=0 from a point O on the ground with speed v0 at an angle 45∘ to the horizontal as shown in Figure 3.44. Compute the magnitude and direction of the angular mometum of the particle about the point O at position :

When the velocity of the paticle is , →V=(0,7v0)ˆi−(0.3v0)ˆjView Solution
Knowledge Check
- Question 1 - Select One or More
A projectile is projected with a velocityv0 at an angle θ with the horizontal as shown in figure. The angular momentum of particle about the origin:
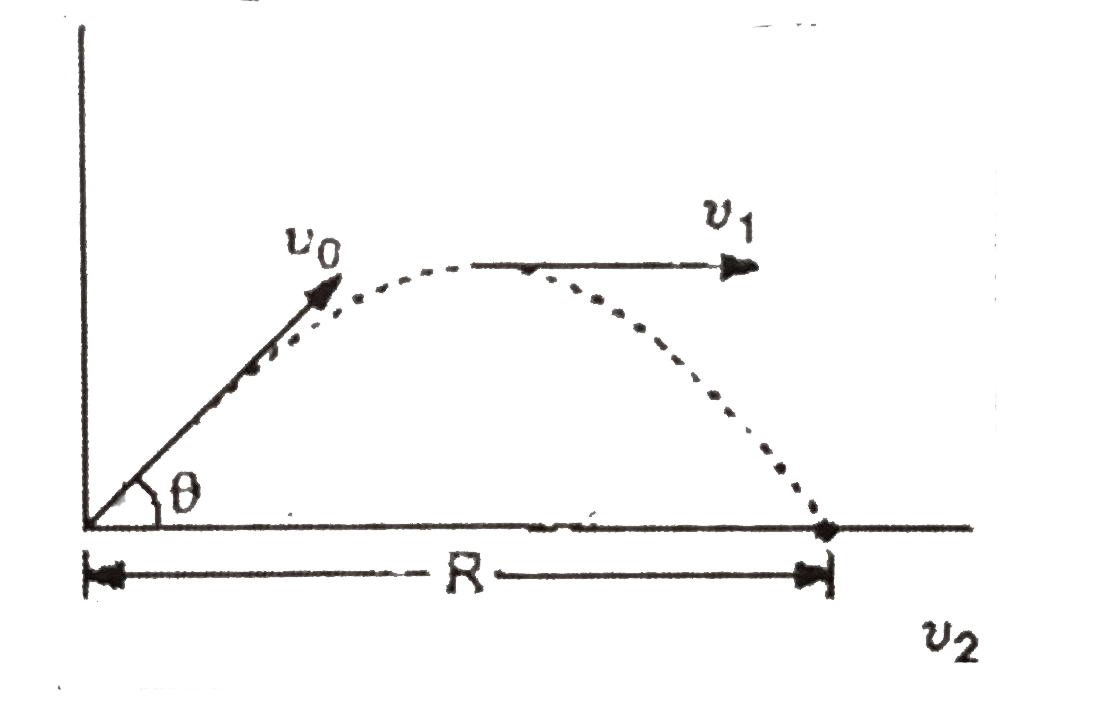 Ais zero when particle is at the originBis −mv3sin2θcosθ2gˆk when particle is at the highest point of trajectoryCis −2mv2sin2θcosθgˆk when particle is just about to hit groundDdownward force of gravity exerts a torque in −z direction.
Ais zero when particle is at the originBis −mv3sin2θcosθ2gˆk when particle is at the highest point of trajectoryCis −2mv2sin2θcosθgˆk when particle is just about to hit groundDdownward force of gravity exerts a torque in −z direction.
A particle is projected at time t=0 from a point P wilth a speed v0 at an angle of 45∘ to the horizontal. Find the magnitude and the direction of the angular momentum of the parallel about the point P at time t=v0g
View SolutionA particle is projected at time t=0 from a point (P) with a speed v0 at an angle of 45∘ to the horizontal.The magnitude of angular momentum of the particle about the point (P) at time (t=v0g) is mv302g√X .Then the value of X is?
View SolutionA particle is projected with a speed v at an angle θ with the horizontal. Find the mean power delivered by gravity during the ascent of the particle.
View SolutionA particle of mass m is projected with a speed v0 at an angle of projection θ with horizontal. Find the torque of the weight of the particle about the point of projection after time v0sinθg
View SolutionA particle is projected at time t=0 from a point O with a speed u at an angle θ to horizontal. Find the torque of a gravitational force on projectile about the origin at time t.(x,y plane is vertical plane)
View SolutionA particle of mass m is projected from origin O with speed u at an angle θ with positive x-axis. Positive y-axis is in vertically upward. Direction. Find the angular momentum of particle at any time t about O before the particle strkes the ground again.
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View Solution

