A particle of mass 'm' is projected with a velocity v=kVe(k<1) from the surface of the earth.(Ve = escape velocity)The maximum height above the surface reached by the particle is:
R2k1+k
Rk21−k2
R(k1−k)2
R(k1+k)2
To solve the problem of finding the maximum height above the surface reached by a particle of mass 'm' projected with a velocity v=kVe (where k<1) from the surface of the Earth, we will apply the principle of conservation of energy.
1. Understanding Escape Velocity:
The escape velocity Ve from the surface of the Earth is given by the formula:
Ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
2. Initial Velocity:
The particle is projected with a velocity v=kVe. Thus, we can express the initial kinetic energy KEi of the particle as:
KEi=12mv2=12m(kVe)2=12mk2V2e
3. Potential Energy at the Surface:
The gravitational potential energy PEi at the surface of the Earth is given by:
PEi=−GMmR
4. Total Initial Energy:
The total initial energy Ei when the particle is projected is the sum of its kinetic and potential energy:
Ei=KEi+PEi=12mk2V2e−GMmR
5. Final Energy at Maximum Height:
At the maximum height h, the velocity of the particle becomes zero, so the kinetic energy KEf at that point is:
KEf=0
The potential energy PEf at height h is:
PEf=−GMmR+h
6. Total Final Energy:
The total final energy Ef at maximum height is:
Ef=KEf+PEf=0−GMmR+h
7. Applying Conservation of Energy:
According to the conservation of energy, the initial energy equals the final energy:
Ei=Ef
Substituting the expressions we derived:
12mk2V2e−GMmR=−GMmR+h
8. Simplifying the Equation:
We can cancel m from both sides (assuming m≠0):
12k2V2e−GMR=−GMR+h
Rearranging gives:
12k2V2e=GMR−GMR+h
9. Finding h:
We can simplify the right side:
GMR−GMR+h=GM((R+h)−RR(R+h))=GMhR(R+h)
Thus, we have:
12k2V2e=GMhR(R+h)
Rearranging gives:
h=R(R+h)GM⋅12k2V2e
10. Final Expression for Maximum Height:
Solving for h yields:
h=R2k2V2eg
Substituting Ve=√2gR:
h=R2k2⋅2gRg=k2R
Conclusion:
The maximum height h above the surface reached by the particle is:
h=k2R
The escape velocity Ve from the surface of the Earth is given by the formula:
Ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
The particle is projected with a velocity v=kVe. Thus, we can express the initial kinetic energy KEi of the particle as:
KEi=12mv2=12m(kVe)2=12mk2V2e
The gravitational potential energy PEi at the surface of the Earth is given by:
PEi=−GMmR
The total initial energy Ei when the particle is projected is the sum of its kinetic and potential energy:
Ei=KEi+PEi=12mk2V2e−GMmR
At the maximum height h, the velocity of the particle becomes zero, so the kinetic energy KEf at that point is:
KEf=0
The potential energy PEf at height h is:
PEf=−GMmR+h
The total final energy Ef at maximum height is:
Ef=KEf+PEf=0−GMmR+h
According to the conservation of energy, the initial energy equals the final energy:
Ei=Ef
Substituting the expressions we derived:
12mk2V2e−GMmR=−GMmR+h
We can cancel m from both sides (assuming m≠0):
12k2V2e−GMR=−GMR+h
Rearranging gives:
12k2V2e=GMR−GMR+h
We can simplify the right side:
GMR−GMR+h=GM((R+h)−RR(R+h))=GMhR(R+h)
Thus, we have:
12k2V2e=GMhR(R+h)
Rearranging gives:
h=R(R+h)GM⋅12k2V2e
Solving for h yields:
h=R2k2V2eg
Substituting Ve=√2gR:
h=R2k2⋅2gRg=k2R
The maximum height h above the surface reached by the particle is:
h=k2R
Topper's Solved these Questions
Similar Questions
A particle of mass'm' is projected with velocity v0 at an angle 'α' with the horizontal. The coefficient of restitution for any of its impact with the smooth ground is e.
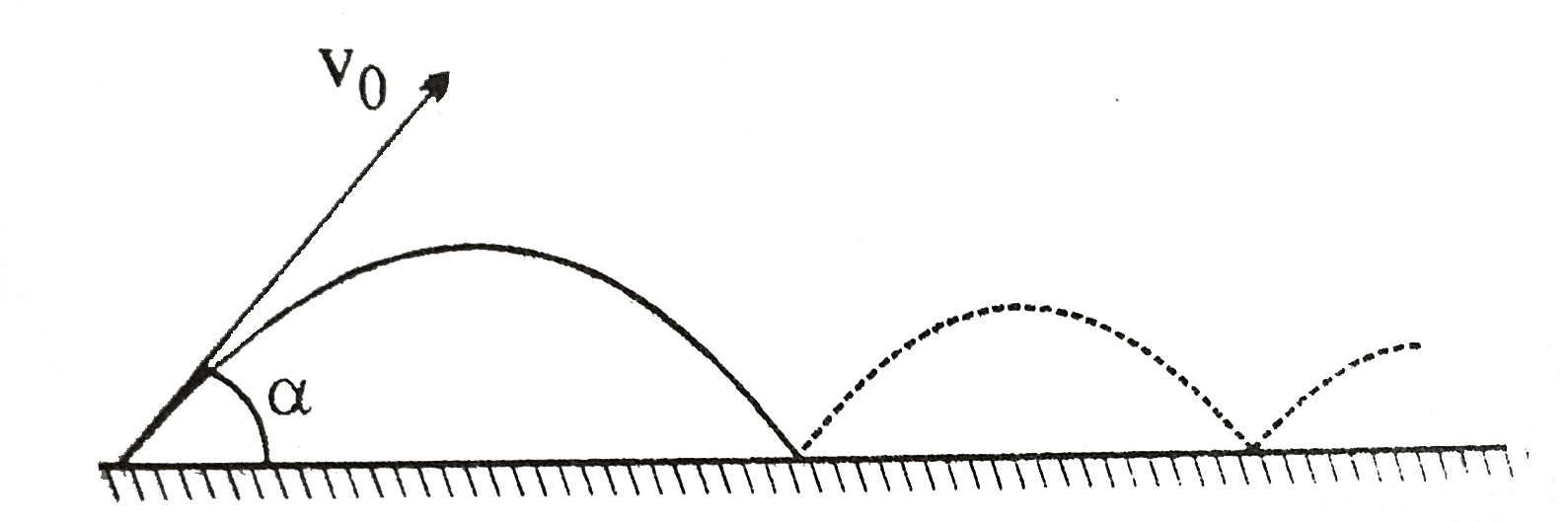
Find total time taken by the particle before it stops moving vertically?
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.
View Solution
Knowledge Check
A partricle of mass m is projected with a velocity v at an angle of 60∘ with horizontal When the particle is at the maximum height. The magnitude ofits angular momentum about the point ofprojection is
AzeroB3mv216gC√3mv316gD3mv316gA particle of mass m is projected with a velocity v making an angle of 45° with the horizontal. The magnitude of the angular momentum of the projectile about the point of projection, when the particle is at its maximum height h, is
AzeroBmv34√2gCmv3√2gDm√2gh3A particle of mass m is projected with a velocity v making an angle of 45∘ with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
AzeroB(mv)34√2gC(mv)3√2gDm√2gh3
A particle of mass m is projected with a speed v0 at an angle of projection θ with horizontal. Find the torque of the weight of the particle about the point of projection after time v0sinθg
View SolutionA particle having mass m and charge q is projected with a velocity v making an angle q with the direction of a uniform magnetic field B. Calculate the magnitude of change in velocity of the particle after time t=πm2qB.
View SolutionA particle of mass m is projected with velocity making an angle of 45∘ with the horizontal When the particle lands on the level ground the magnitude of the change in its momentum will be .
View SolutionA particle of mass m is projected upword with velocity v=ve2 (ve) escape of the particle is
View SolutionA particle of mass m is projected upwards with velocity v=ve2, where ve is the escape velocity then at the maximum height the potential energy of the particle is : (R is radius of earth and M is mass of earth)
View Solution
