A person looks into the mirror by placing it close to his face. if the image of the face was found erect and magnified, then the mirror must be
plane
concave
convex
plane or concave
The correct Answer is:B
To determine the type of mirror that produces an erect and magnified image when a person looks closely into it, we can analyze the properties of different types of mirrors: plane, concave, and convex.
1. Understanding Image Formation in Different Mirrors:
- A plane mirror produces an image that is erect but has no magnification (magnification = 1). Thus, it cannot be the answer since the image is not magnified.
- A convex mirror also produces an erect image, but it is diminished (the image size is smaller than the object). Therefore, it cannot be the answer either.
2. Analyzing the Concave Mirror:
- A concave mirror can produce an erect and magnified image when the object (in this case, the person's face) is placed between the mirror's focal point (F) and its pole (P).
- When the object is placed close to the mirror (between the pole and the focus), the rays of light diverge, and the extended rays appear to come from a point behind the mirror, creating a virtual image.
3. Conclusion:
- Since the image is erect and magnified when the person looks closely into the mirror, the only type of mirror that satisfies these conditions is a concave mirror.
Final Answer:
The mirror must be a concave mirror.
---
- A plane mirror produces an image that is erect but has no magnification (magnification = 1). Thus, it cannot be the answer since the image is not magnified.
- A convex mirror also produces an erect image, but it is diminished (the image size is smaller than the object). Therefore, it cannot be the answer either.
- A concave mirror can produce an erect and magnified image when the object (in this case, the person's face) is placed between the mirror's focal point (F) and its pole (P).
- When the object is placed close to the mirror (between the pole and the focus), the rays of light diverge, and the extended rays appear to come from a point behind the mirror, creating a virtual image.
- Since the image is erect and magnified when the person looks closely into the mirror, the only type of mirror that satisfies these conditions is a concave mirror.
The mirror must be a concave mirror.
Topper's Solved these Questions
- REFLECTION OF LIGHT BOOK - MCGROW HILL PUBLICATIONCHAPTER - REFLECTION OF LIGHT EXERCISE - HIGHER ORDER THINKING QUESTIONS
10 Videos
- MOTIONBOOK - MCGROW HILL PUBLICATIONCHAPTER - MOTIONEXERCISE - HIGHER ORDER THINKING QUESTIONS
48 Videos
- REFRACTION OF LIGHTBOOK - MCGROW HILL PUBLICATIONCHAPTER - REFRACTION OF LIGHTEXERCISE - HIGHER ORDER THINKING QUESTIONS
37 Videos
Similar Questions
A peson looks into a spherical mirror. The size of image of his face is twice the actual size of his face. If the face is at a distance of 20cm, then find the radius of curvature of the mirror.
View SolutionA tank contains a transparent liquid of refractive index μ. The bottom of the tank is a plane mirror as shown. A person at P looks at an object O and its image in the mirror. The distance between the object and its image in the mirror as preceived by the person is
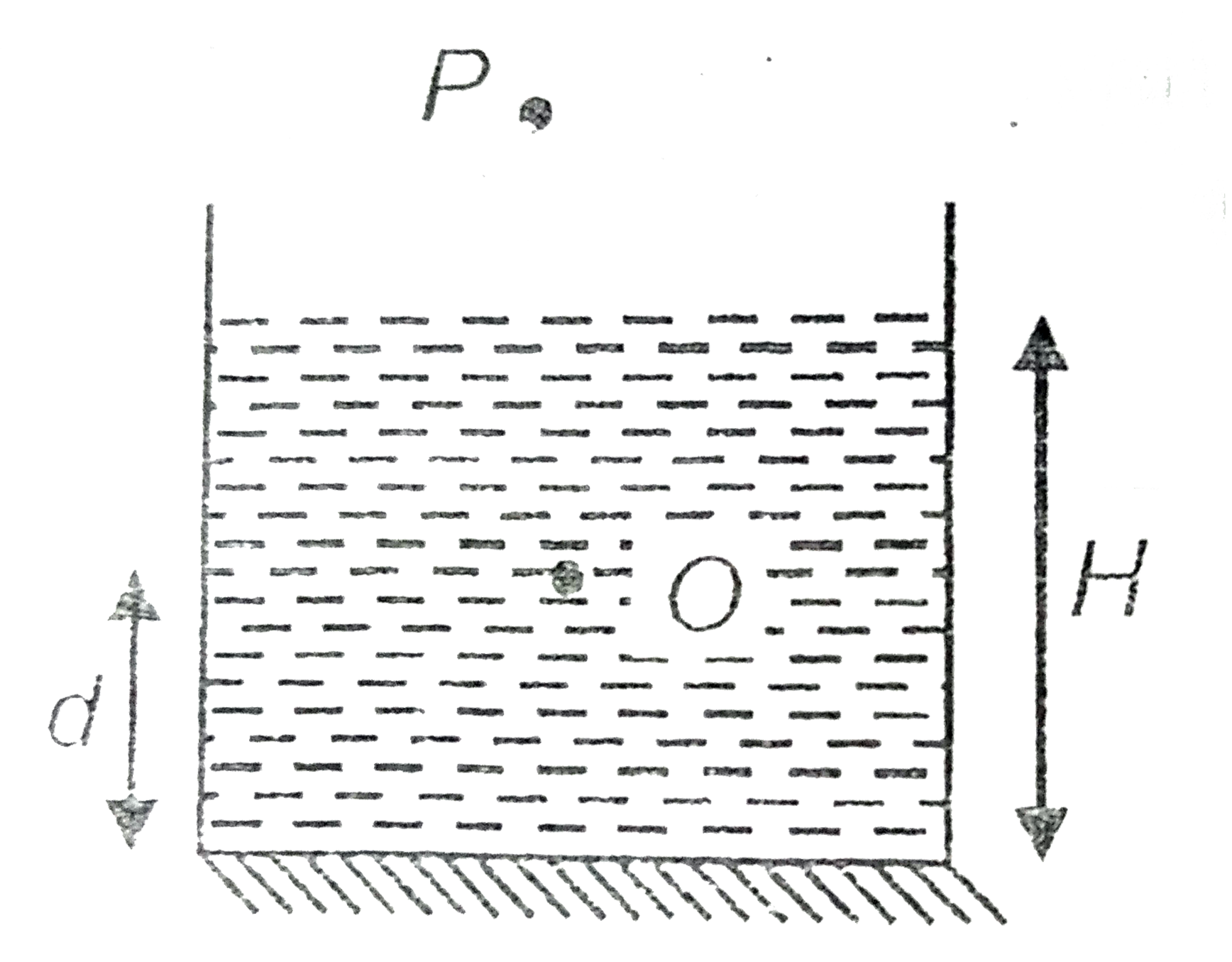
View Solution
Knowledge Check
A person looks into the mirror by placing it close to his face. The image of his face was erect, laterally inverted and of the same size. Then, the mirror must be
AplaneBconcaveCconvexDplane or concaveThe image of our face in a plane mirror
ArealBmagnifiedCdiminishedDnone of theseIn case of a virtual and erect image, the magnification of a mirror is
ApositiveBnegativeCunityDinfinity
What is the position of the object for which the image formed by a concave mirror is virtual, erect and magnified ?
View SolutionWhat is the position of the object for which the image formed by a concave mirror is virtual, erect and magnified ?
View SolutionThe image of our face in a plane mirror is
View SolutionA person uses a concave mirror for shaving, what should be the position of his face in front of it?
View SolutionWhatever be the position of the object, the image formed by a mirror is virtual, erect and smaller than the object. The mirror them must be :
View Solution
