A physics scholar took two spring balances $S_{1} and S_{2}$ that are calibrated on the surface of the Moon and the Earth, respectively. He then weight a body using both $S_{1} and S_{2}$ at a given place on the surface of the Earth. He determined the ratio of the readings. What is the ratio?

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem of determining the ratio of the readings from two spring balances calibrated on the Moon and Earth, we can follow these steps:
1. Understanding the Spring Balance:
- A spring balance measures weight based on the spring force, which is given by Hooke's Law: $F = k x$ , where $F$ is the force exerted by the weight, $k$ is the spring constant, and $x$ is the displacement of the spring from its equilibrium position.
2. Weight on Earth:
- When the scholar weighs a body of mass $m$ on Earth, the weight $W$ is given by the formula:
$W = m g$
- Here, $g$ is the acceleration due to gravity on Earth.
3. Weight on the Moon:
- Similarly, when the same mass $m$ is weighed on the Moon, the weight $W^{'}$ is given by:
$W^{'} = m g^{'}$
- Here, $g^{'}$ is the acceleration due to gravity on the Moon.
4. Using the Spring Balances:
- The spring balance calibrated on the Moon (S1) and the one calibrated on Earth (S2) will give readings based on the respective gravitational accelerations.
- For spring balance S1 (calibrated on the Moon):
$k x_{1} = m g^{'}$
- For spring balance S2 (calibrated on Earth):
$k x_{2} = m g$
5. Setting Up the Ratio:
- Since both balances are measuring the same mass $m$ when brought to Earth, we can set up the ratio of their readings:
$\frac{x_{1}}{x_{2}} = \frac{m g^{'}}{m g}$
- The mass $m$ cancels out:
$\frac{x_{1}}{x_{2}} = \frac{g^{'}}{g}$
6. Values of g and g':
- The acceleration due to gravity on the Moon $g^{'}$ is approximately $\frac{1}{6}$ of that on Earth $g$ . Thus:
$g^{'} \approx \frac{g}{6}$
7. Final Ratio Calculation:
- Substituting $g^{'}$ into the ratio:
$\frac{x_{1}}{x_{2}} = \frac{\frac{g}{6}}{g} = \frac{1}{6}$
Conclusion:
The ratio of the readings from the two spring balances $S_{1}$ and $S_{2}$ when weighing the same body on Earth is:
$\frac{x_{1}}{x_{2}} = \frac{1}{6}$

Updated on:18/9/2024

Reading of spring balance $S_{1}$ and $S_{2}$ (Pulley are ideal)

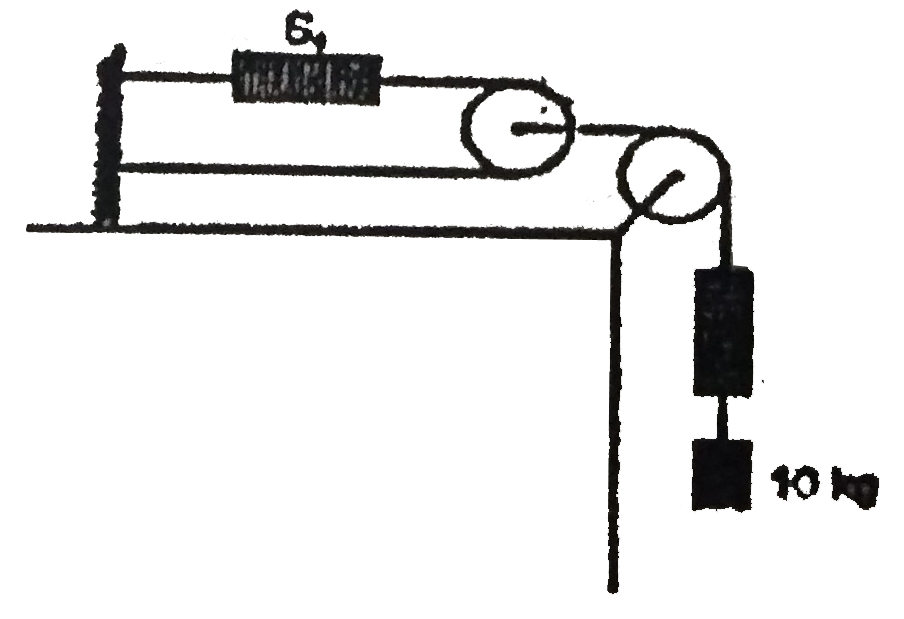A10 kg, 10 kg
B5 kg,5 kg
C5 kg, 10 kg
D10 kg,5 kg
$S_{1}$ and $S_{2}$ are two equipotential surfaces on which the potentials are not equal
A $S_{1}$ and $S_{2}$ cannot intersect.
B $S_{1}$ and $S_{2}$ cannot both be plane surfaces.
CIn the region between $S_{1}$ and $S_{2}$ the field is maximum where they are closest to each other.
DA line of force from $S_{1}$ and $S_{2}$ must be perpendicular to both.
The height above the earths surface at which the weight of a person becomes 1/4th of his weight on the surface of earth is (R is the radius of earth)
A2 R
BR
C3 R
D5 R