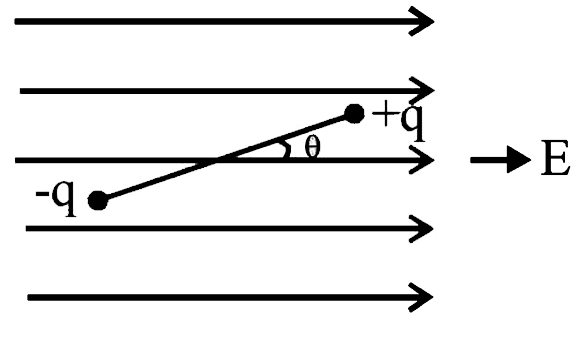
A point mass M is attached to one end of a masslesa rigid non-conducting rod of length L. Another equal point mass is attached to the other end of the rod. The two particles carry charges $+ q$ and $- q$ respectively. This arrangement is held I the region of uniform electric field E such that the rod makes a small $θ$ (say about $5^{\circ}$ ) with field direction. Moment of inertia of the rod is I.
Now answer the question
Time period for the rod to become parallel to E is

A

$2 π \frac{\sqrt{M L}}{q E})$

B

$\frac{2 π}{3} \sqrt{\frac{M L}{q E}}$

C

$\frac{π}{3} \sqrt{\frac{M L}{2 q E}}$

D

$\frac{π}{4} \frac{\sqrt{M L}}{2 q E})$

Text Solution

Verified by Experts

The correct Answer is:C

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two point masses, m each carrying charges $- q$ and $+ q$ are attached to the ends of a massless rigid non - conducting wire of length L. When this arrangement is placed in a uniform electric field, then it deflects through an angle $θ$ . The minimum time needed by the rod to align itself along the field is
A $2 π \sqrt{\frac{m L}{q E}}$
B $\frac{π}{2} \sqrt{\frac{m L}{2 q E}}$
C $π \sqrt{\frac{2 m L}{q E}}$
D $2 π \sqrt{\frac{3 m L}{q E}}$
Question 2 - Select One
The moment of inertia of a thin rod of mass M and length L about an axis perpendicular to the rod at a distance L/4 from one end is
A $\frac{M L^{2}}{6}$
B $\frac{M L^{2}}{12}$
C $\frac{7 M L^{2}}{24}$
D $\frac{7 M L^{2}}{48}$
Question 3 - Select One
Two masses each of mass $M$ are attached to the end of a rigid massless rod of length $L$ . The moment of interia of the system about an axis passing centre of mass and perpendicular to its length is.
A $\frac{M L^{2}}{4}$
B $\frac{M L^{2}}{2}$
C $M L^{2}$
D $2 M L^{2}$

CHHAYA PUBLICATION-ELECTRIC FIELD-ENTRACNCE CORNER ( COMPREHENSION TYPE )

Home
Ask Doubt
Profile