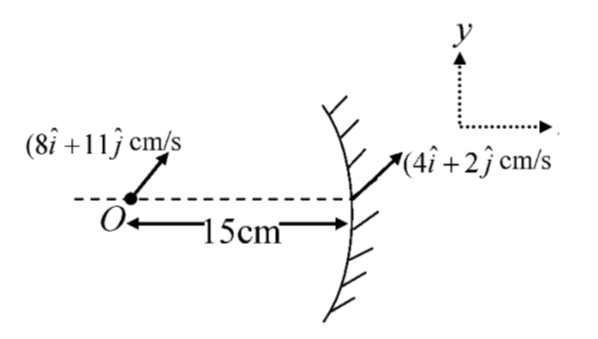
A point object located at a distance of 15 cm from the pole of concave mirror of focal length 10 cm on its principal axis is moving with velocity (8ˆi+11ˆj) cm/s. the velocity of mirror is (4ˆi+2ˆj) cm/s. if the speed of the image in cm/s 4k, find the value of k.
The correct Answer is:5
Step by step video & image solution for A point object located at a distance of 15 cm from the pole of concave mirror of focal length 10 cm on its principal axis is moving with velocity (8hati+11hatj) cm/s. the velocity of mirror is (4hati+2hatj) cm/s. if the speed of the image in cm/s 4k, find the value of k. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
An object is placed at a distance 20 cm from the pole of a convex mirror of focal length 20 cm. The image is produced at
View SolutionAn object is placed at a distance of 20 cm from a concave mirror of focal lenght 10 cm. What is the image distance?
View SolutionA point object is placed at distance of 30 cm in front of a convex mirror of Focal length 30 cm. The image will form at
View SolutionAn object is placed 40 cm from a concave mirror of focal length 20 cm. The image formed is
View SolutionAn object is placed at 6 cm distance in front of a concave mirror of focal length 4 cm.
Find the position of the image period.
View SolutionAn object is at 20 cm from a concave mirror of focal length 10 cm, the nature of image is
View SolutionAn object is placed at 6 cm distance in front of a concave mirror of focal length 4 cm.
What will be the nature of the image?
View SolutionAn object 4cm in size is placed at a distance of 25.0cm from a concave mirror of focal length 15.0 cm. Find the position, nature and height of the image.
View SolutionFind the distance of object from a concave mirror of focal length 10 cm so that image size is four time the size of the object.
View SolutionObject is placed 15 cm from a concave mirror of focal length 10 cm, then the nature of the image formed will be
View Solution
